Set11
Set12
Set13
Set14
Set21
Set22
Set23
Set31
Set32
Set33
Set41
Set42
Set43
Set51
Set52
Set53
Set61
Set62
Set63
Set71
Set72
Set73
Set81
Set82
Set83
FraimanN01
FraimanN03
FraimanN04
FraimanN06
FraimanN07
Supplemental data: The Brain of Dante
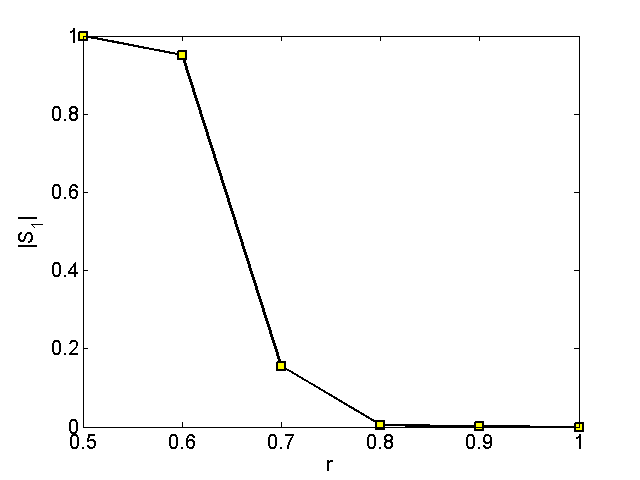
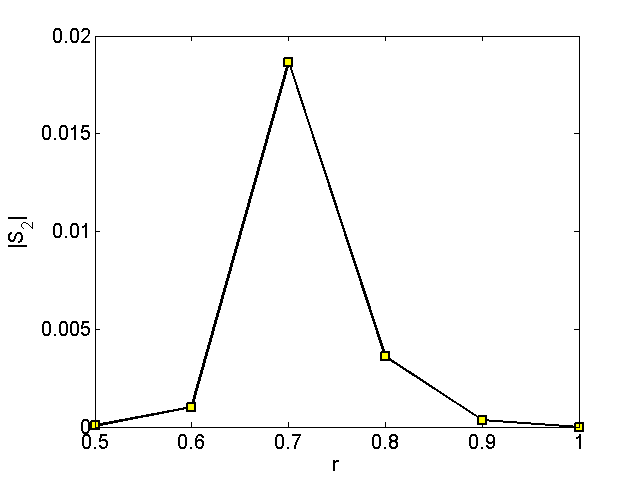
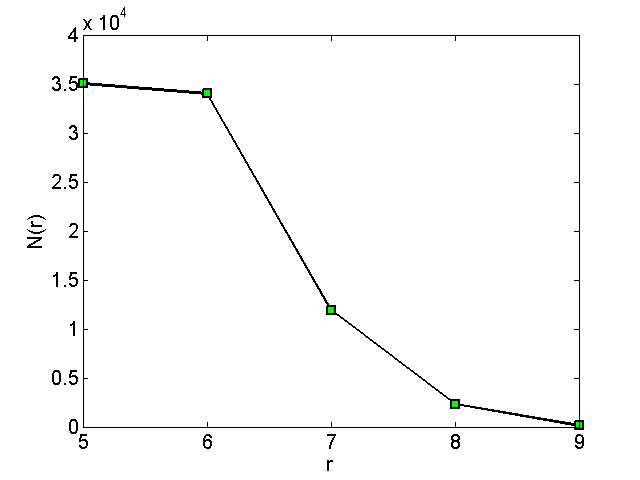
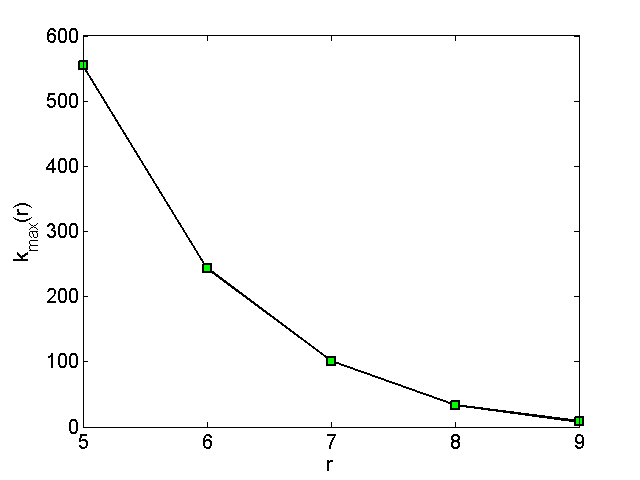
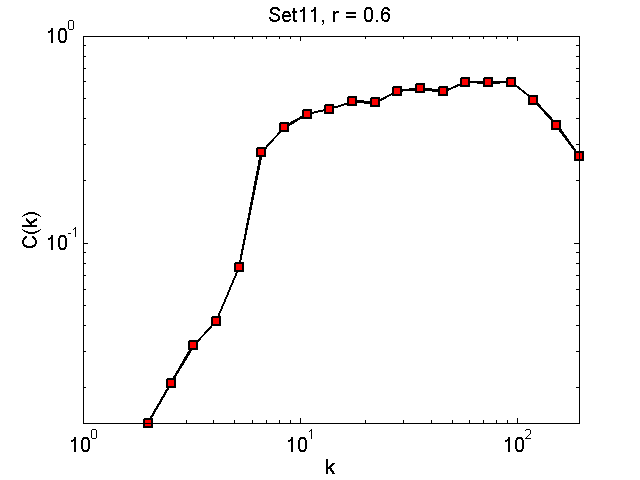
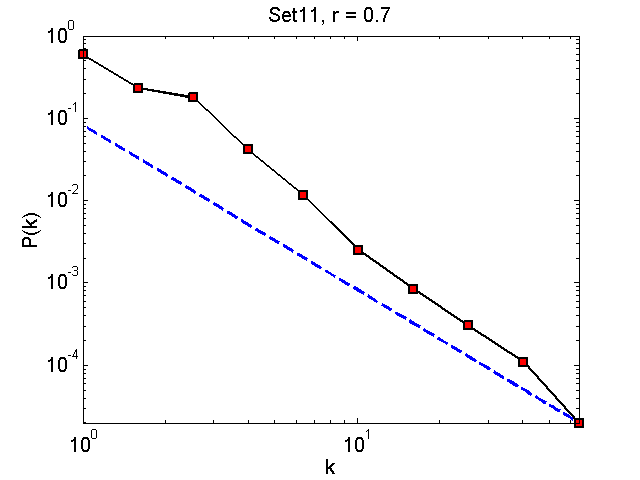
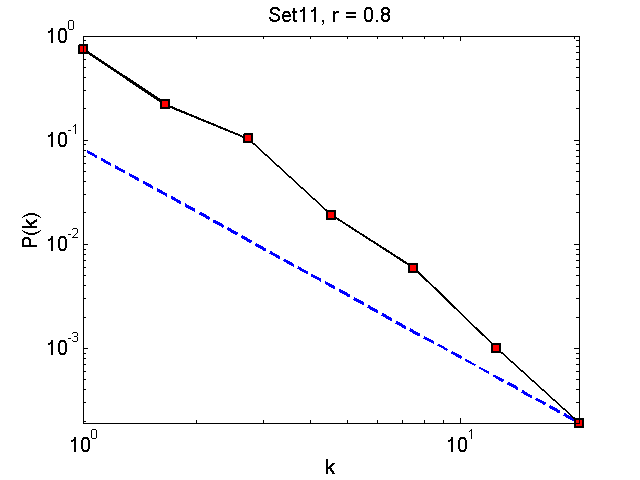
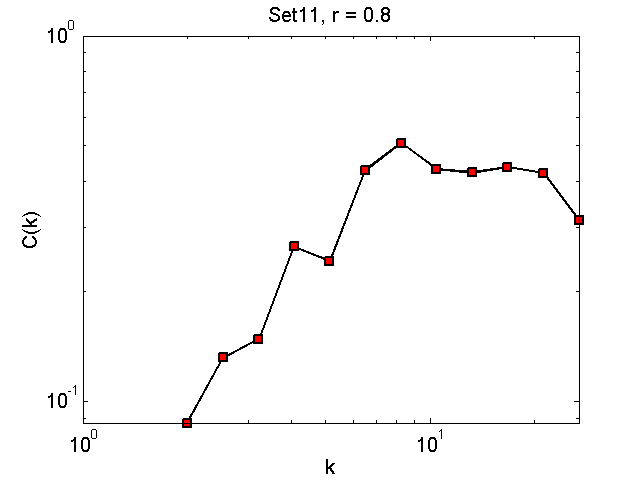
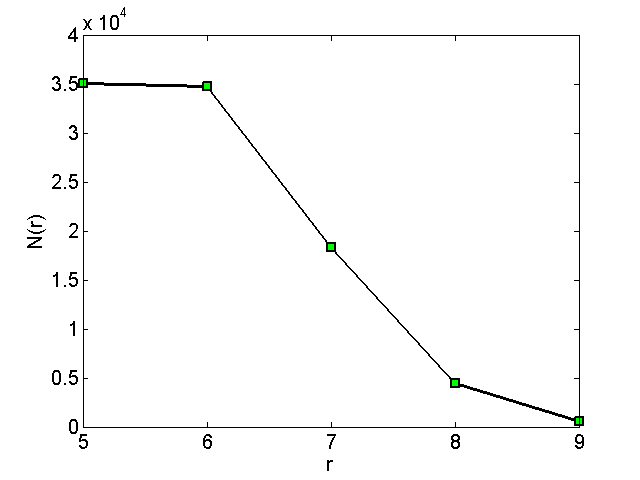
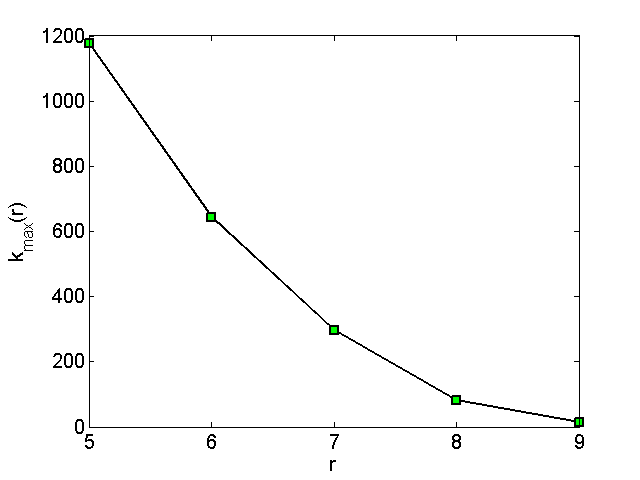
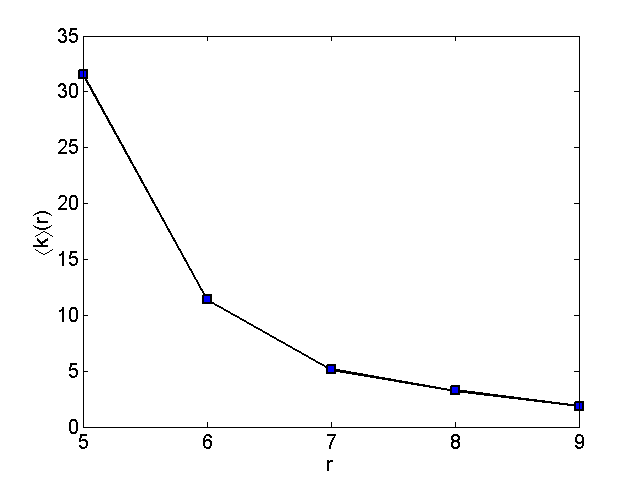
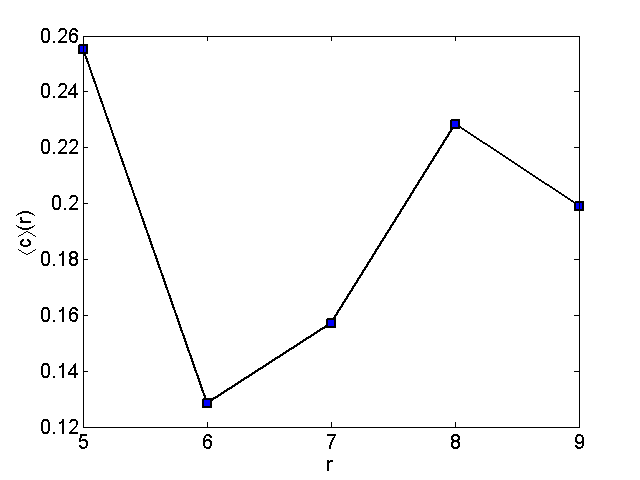
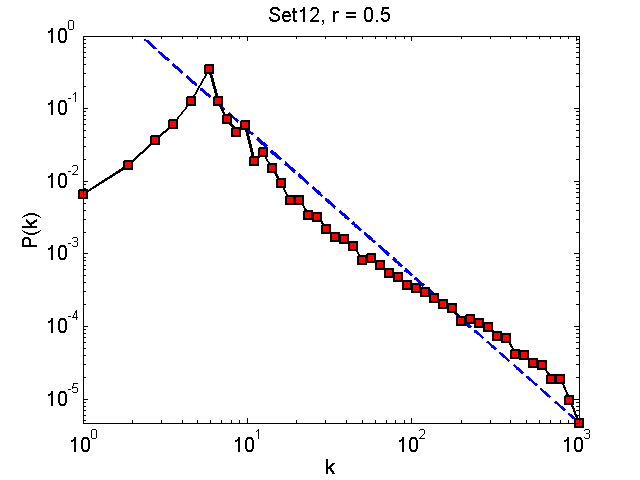
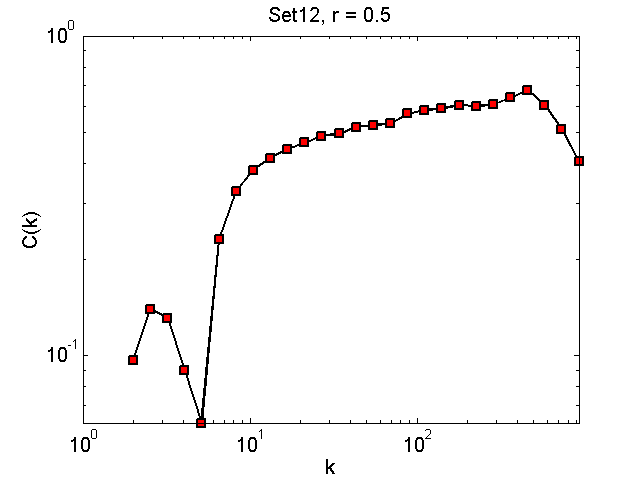
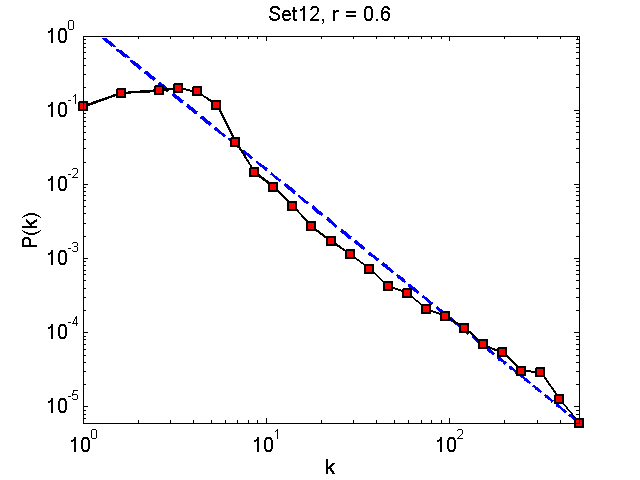
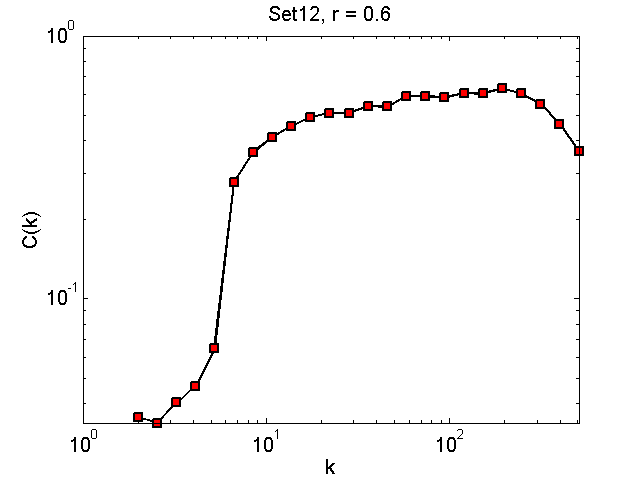
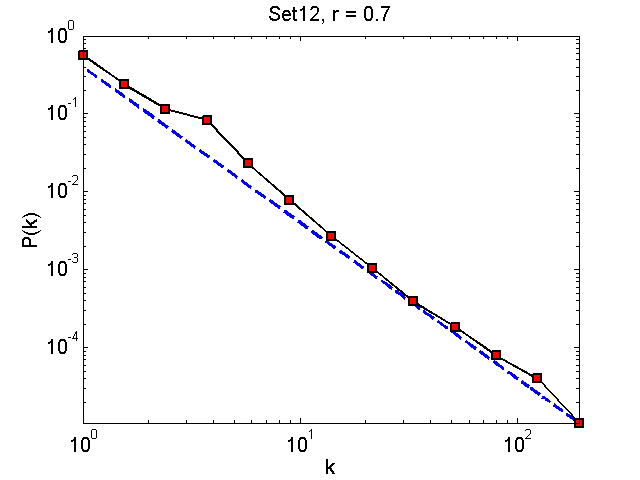
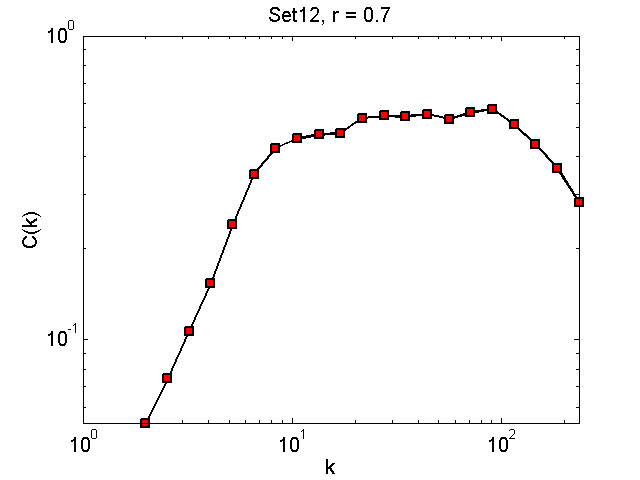
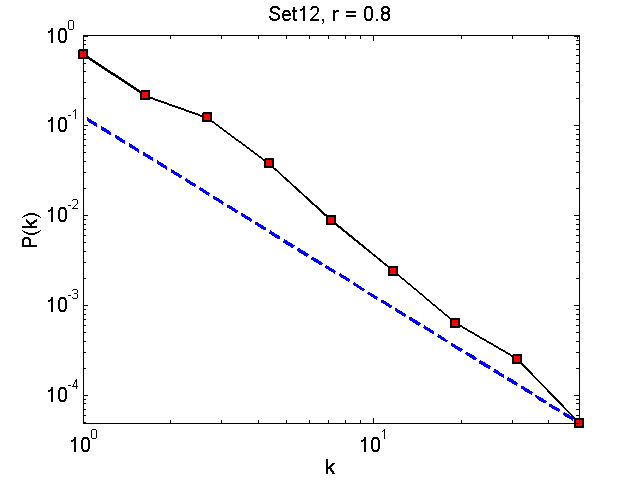
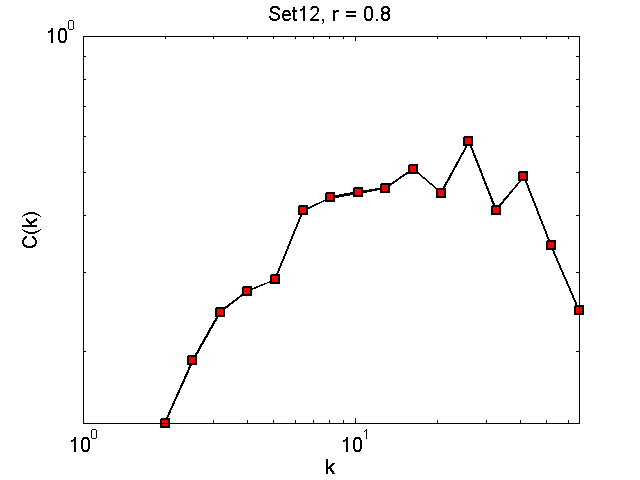
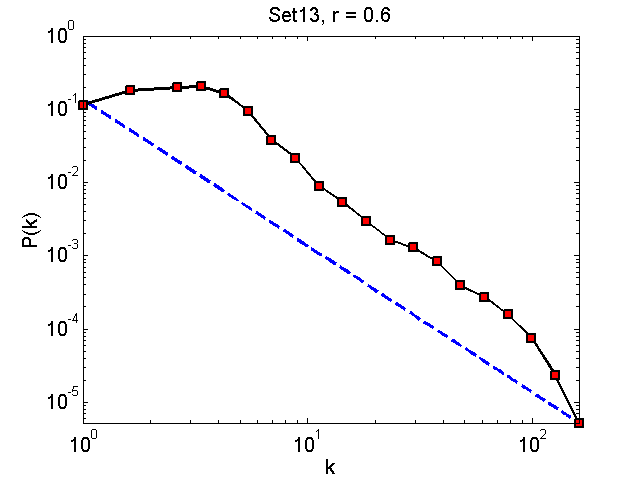
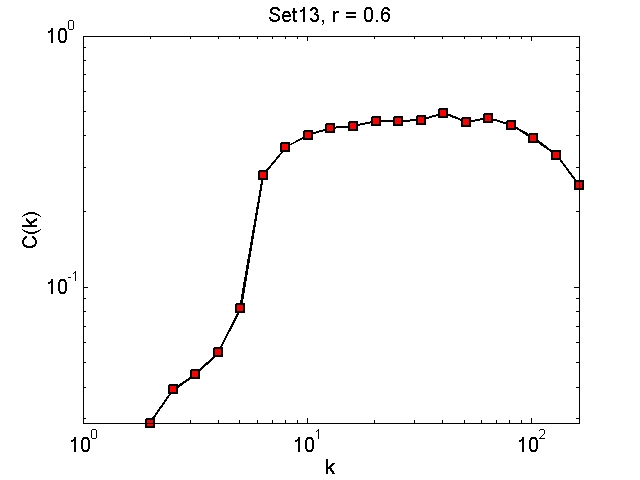
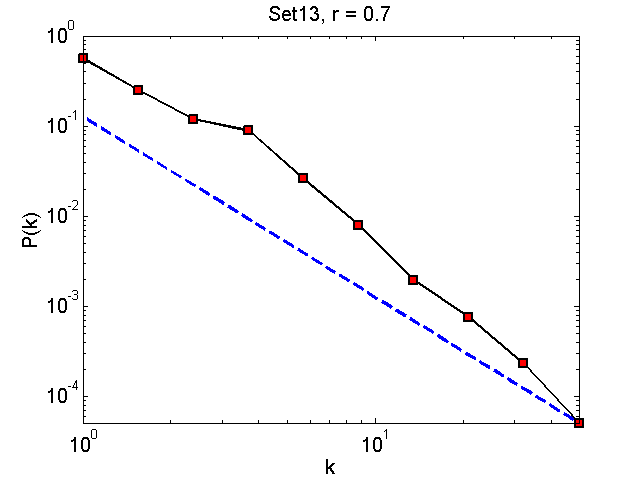
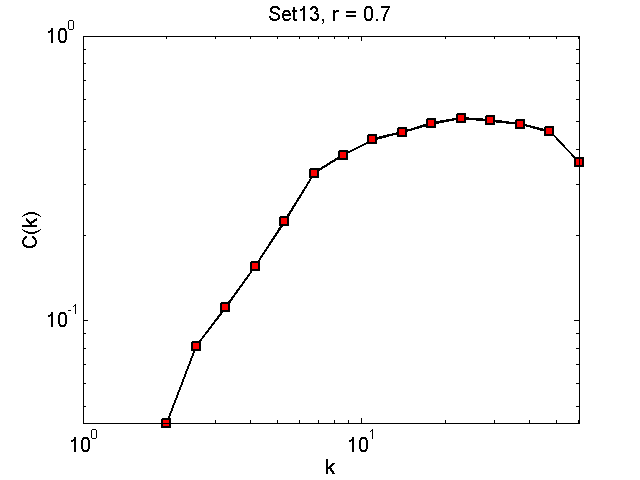
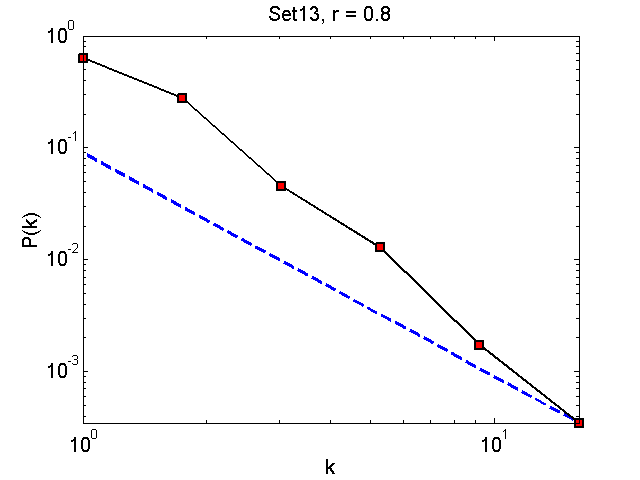
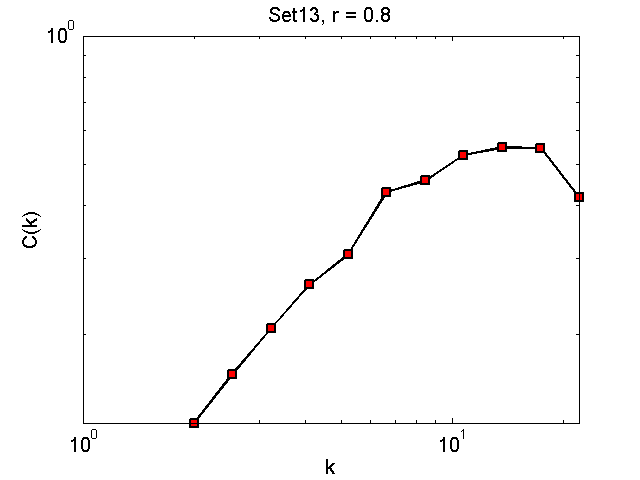
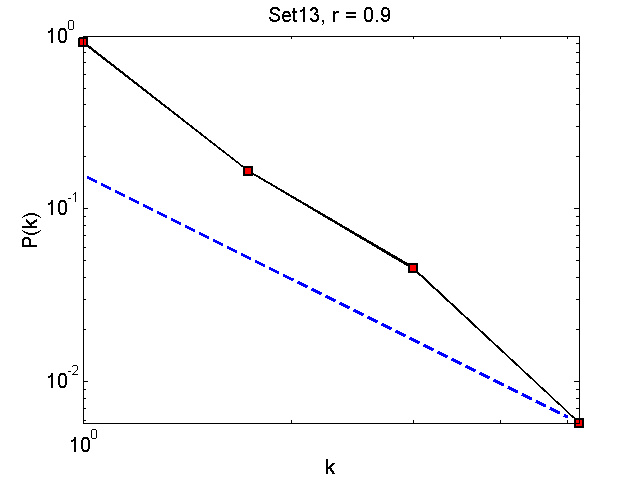
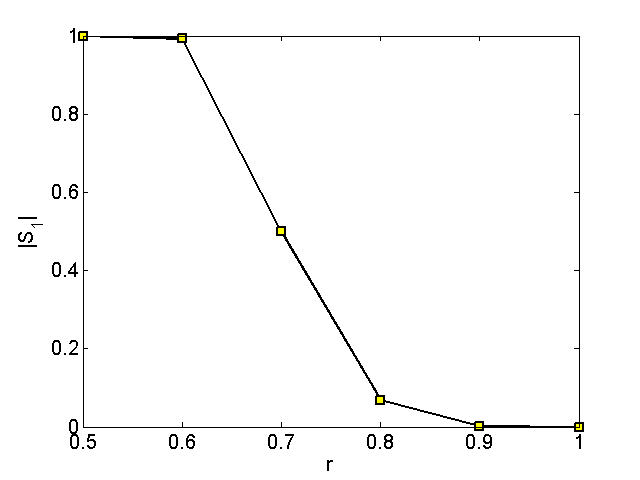
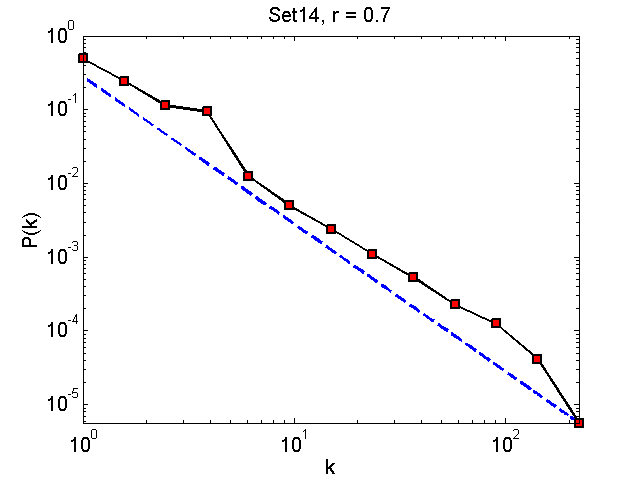
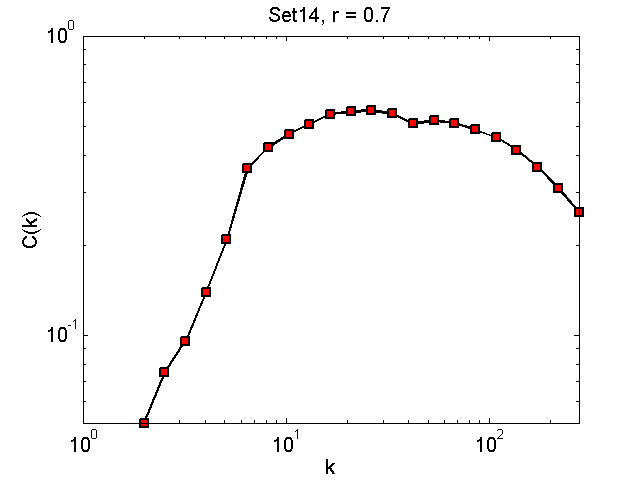
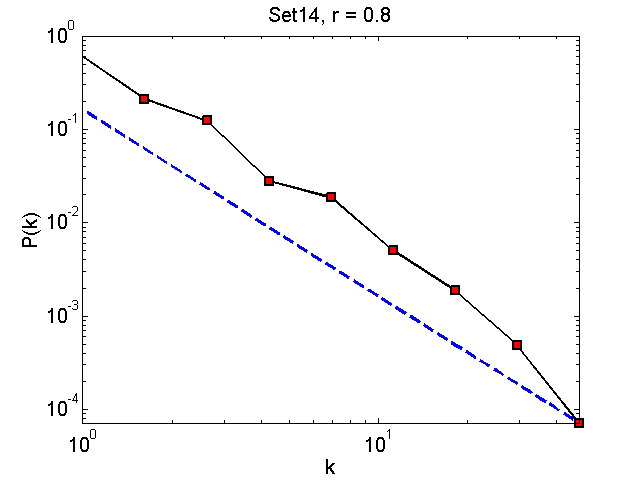
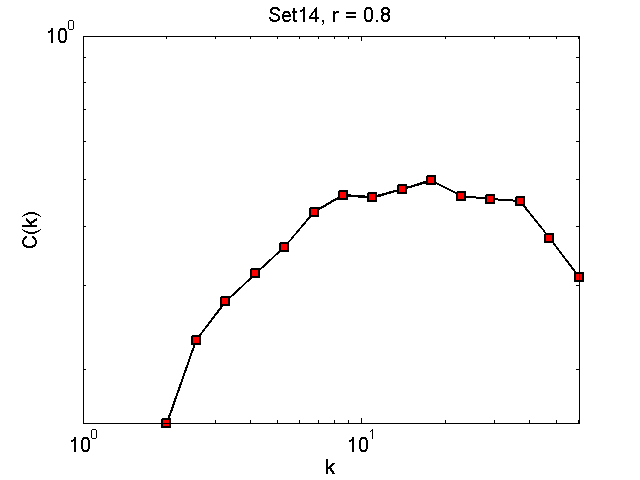
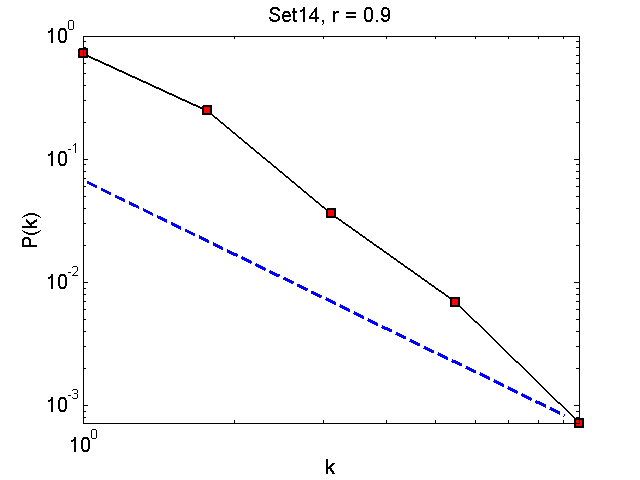
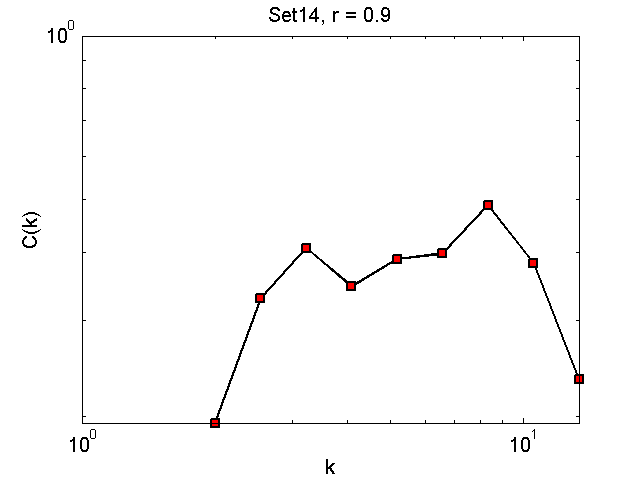
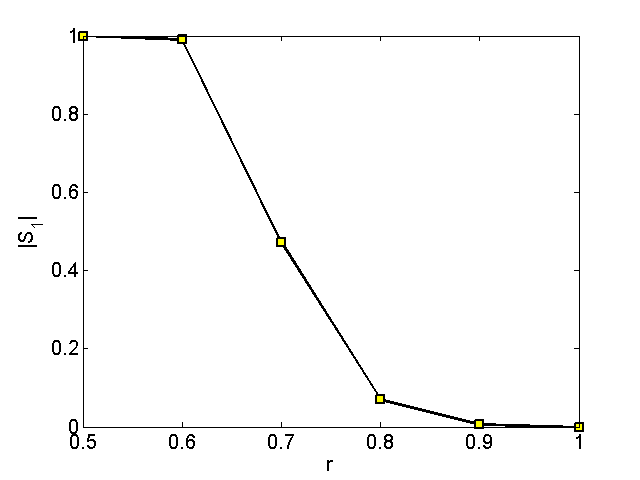
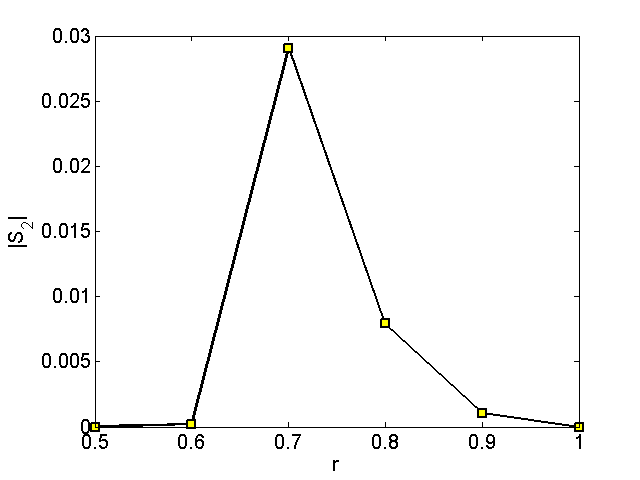
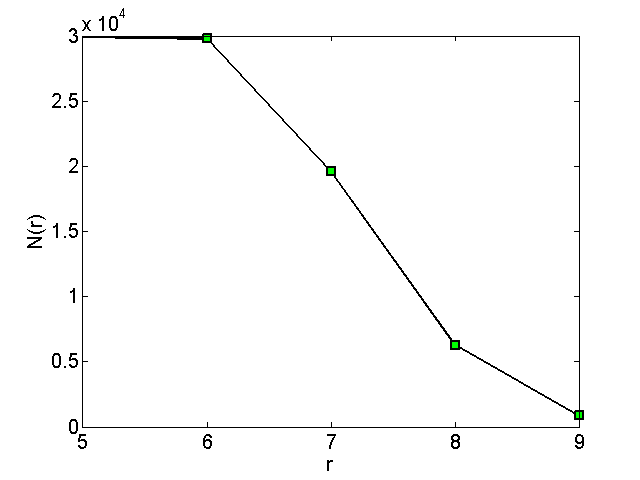
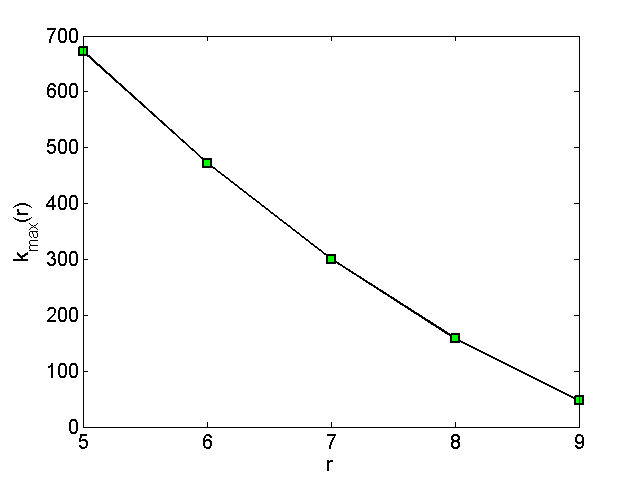
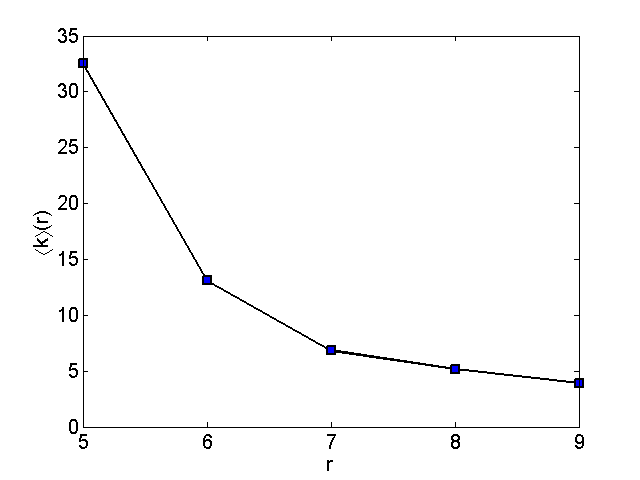
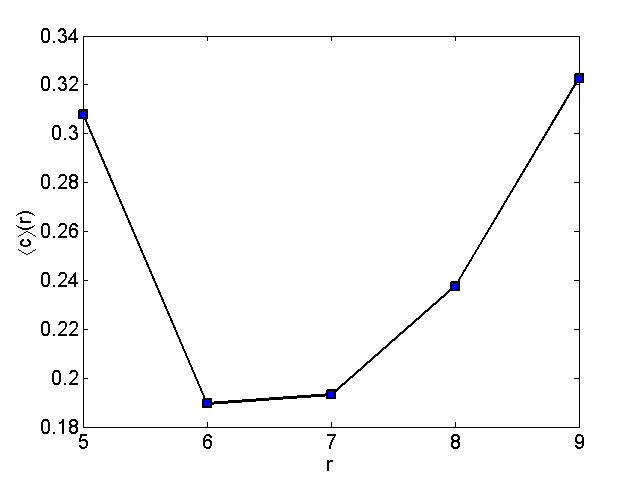
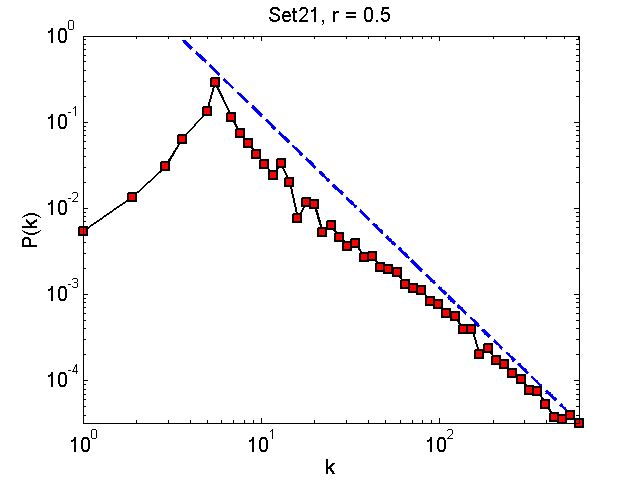
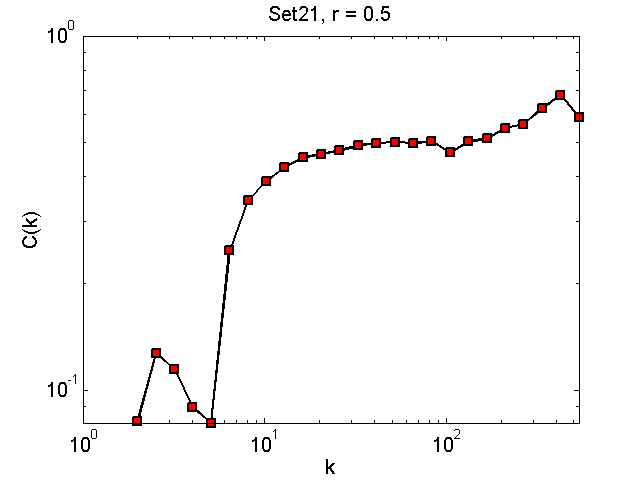
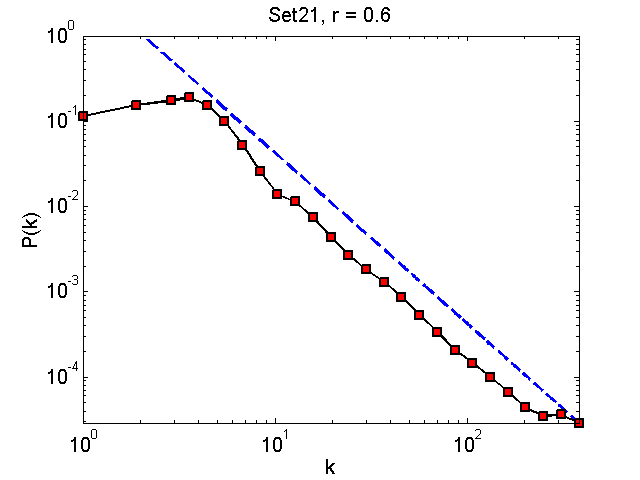
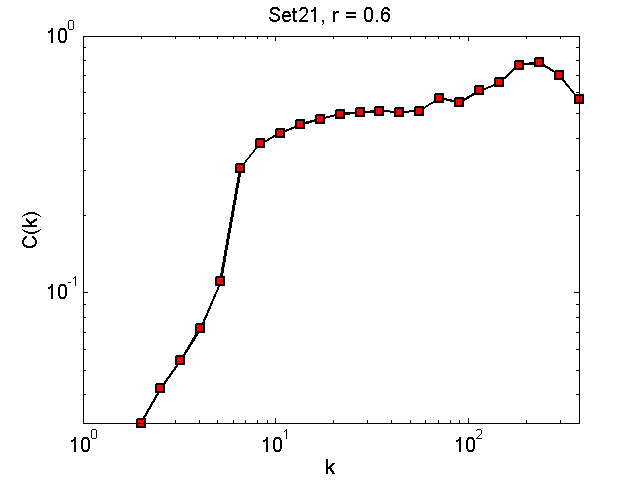
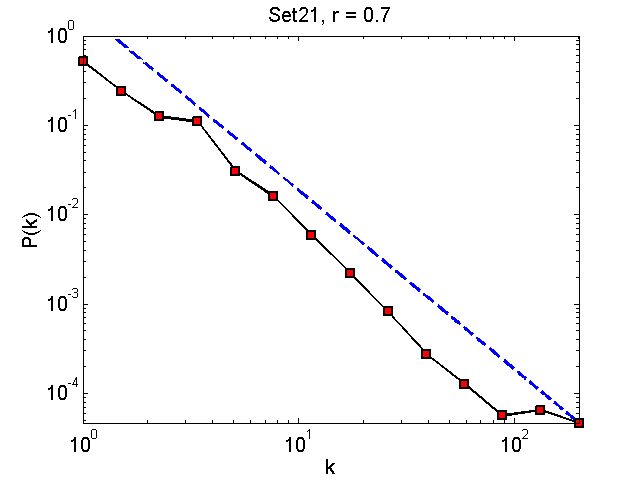
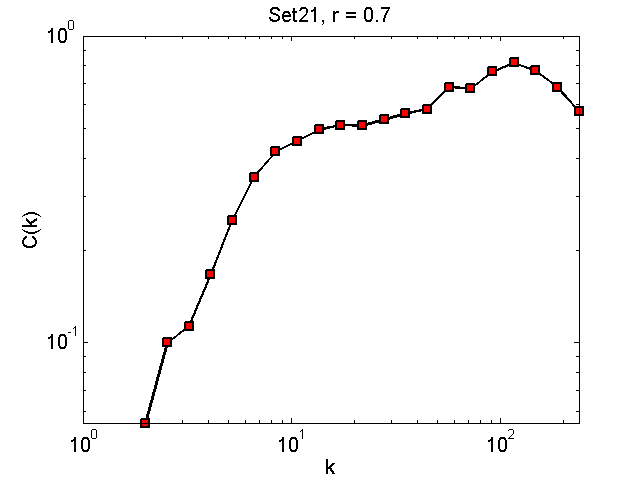
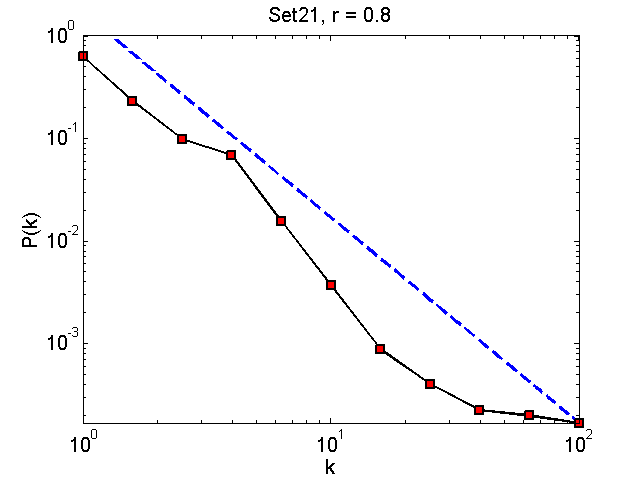
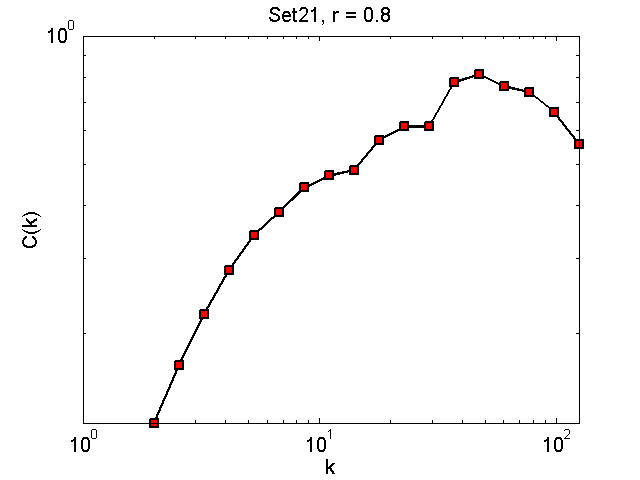
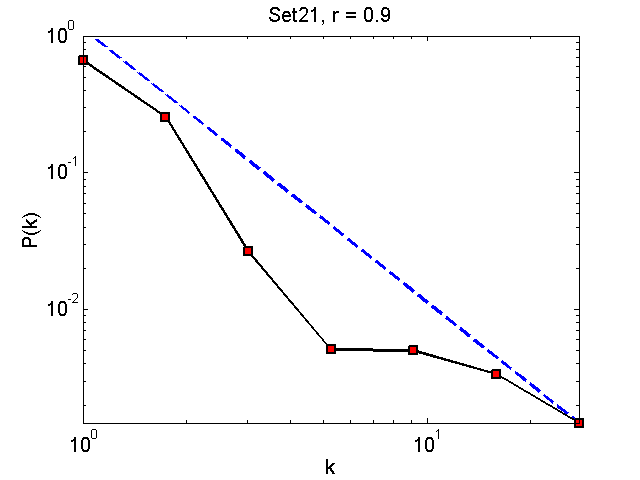
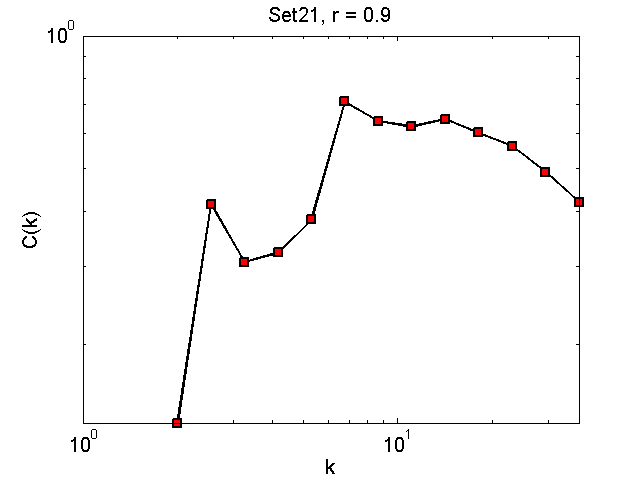
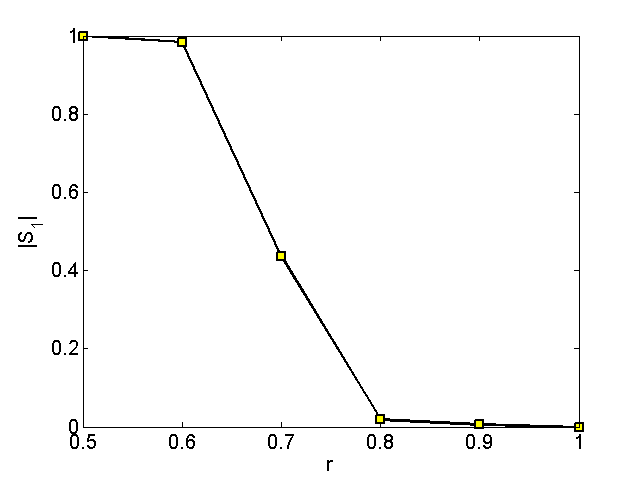
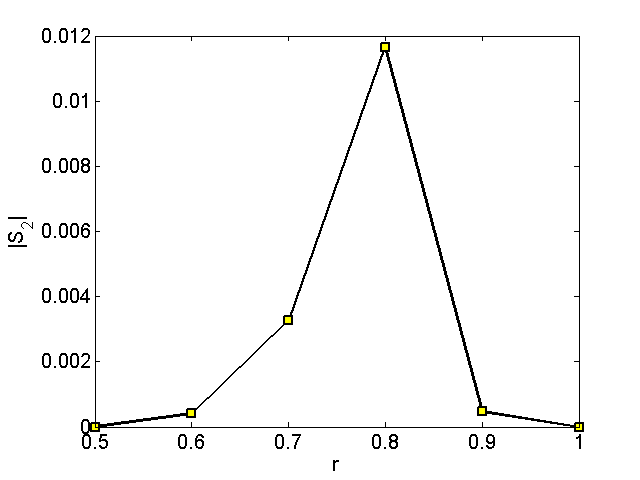
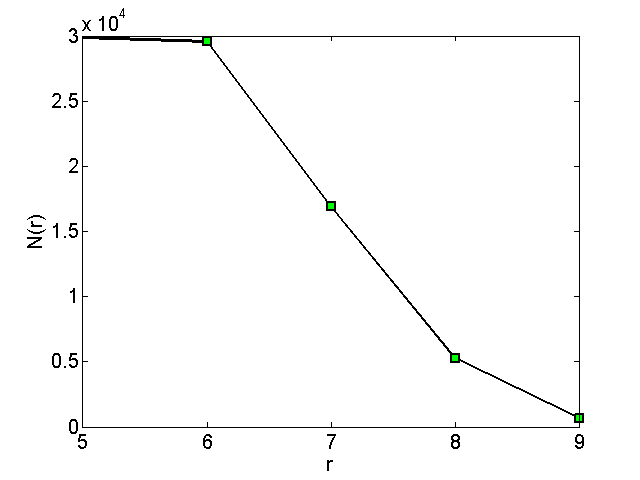
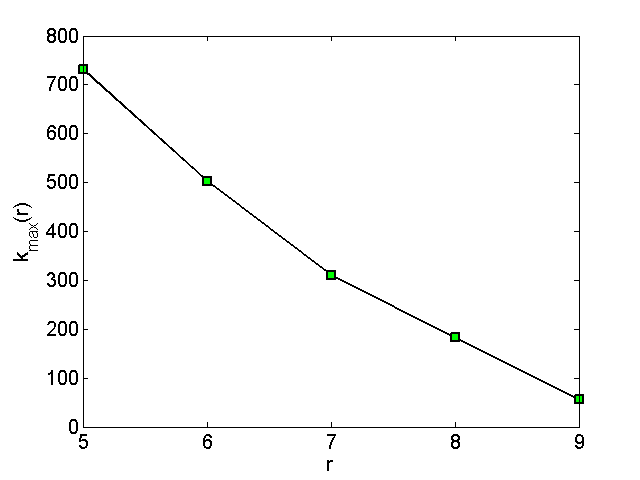
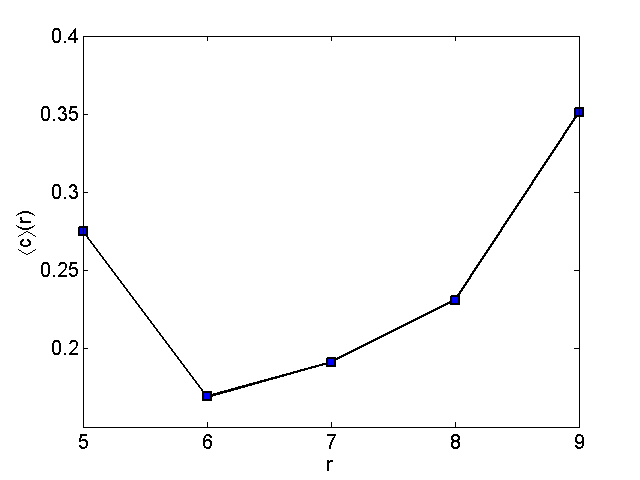
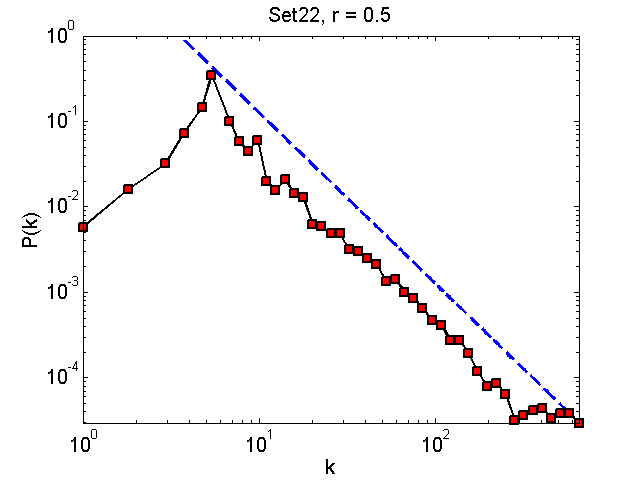
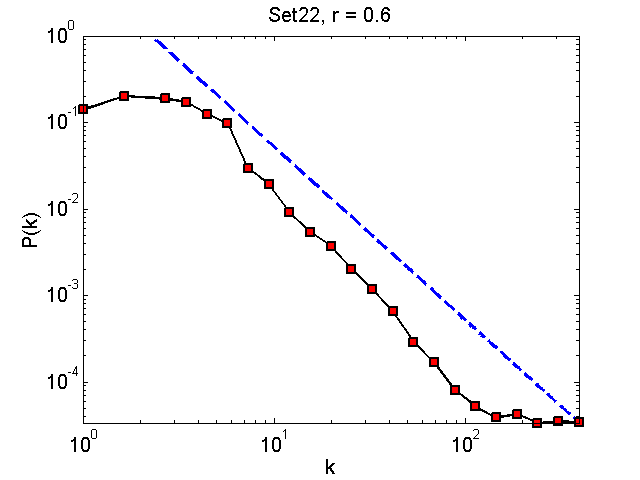
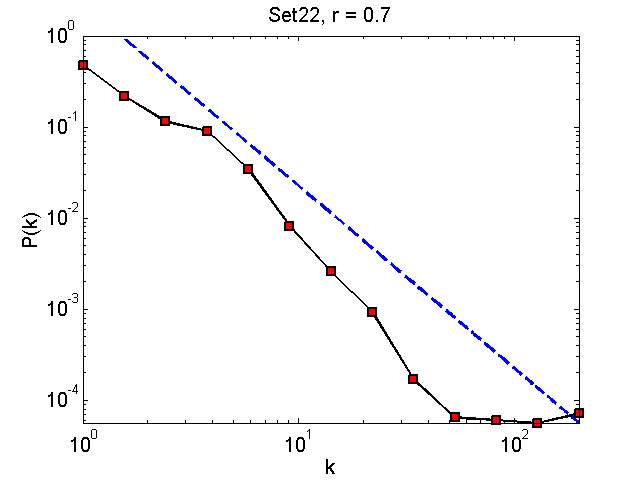
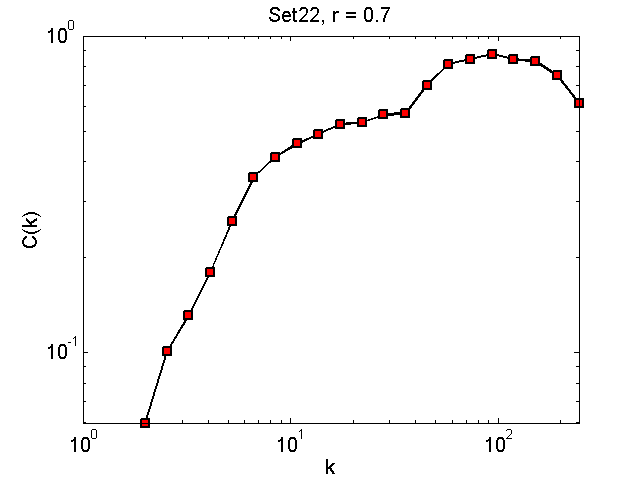
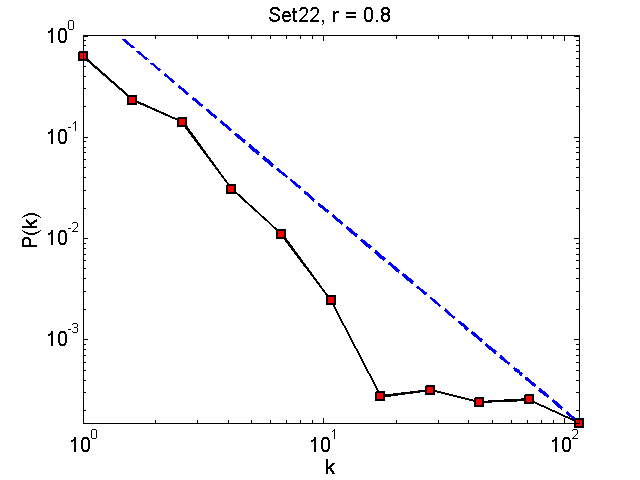
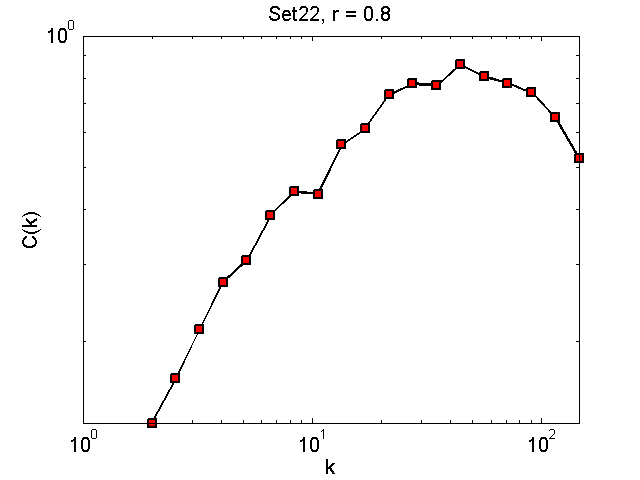
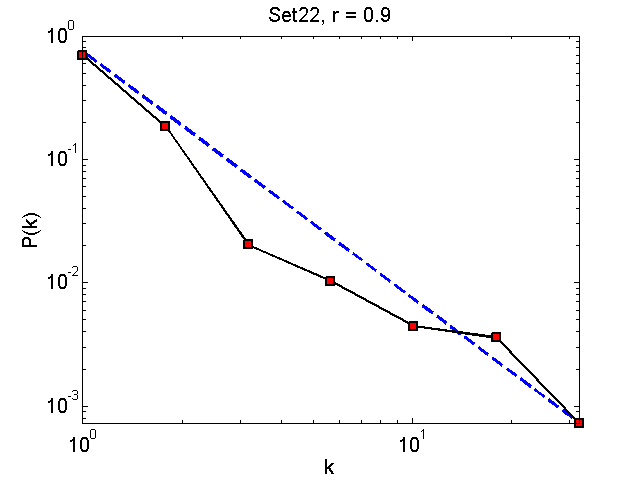
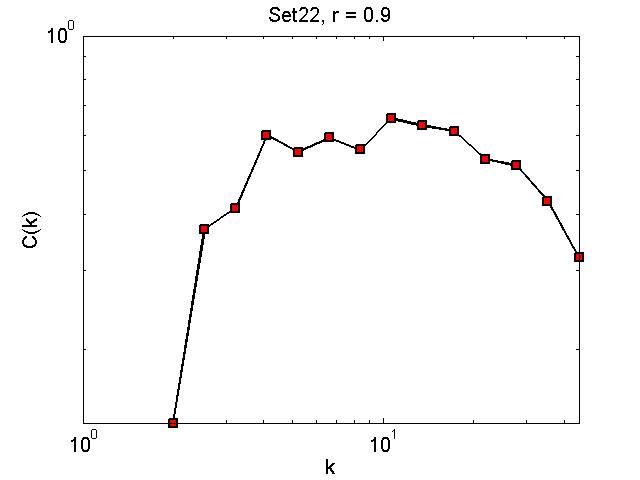
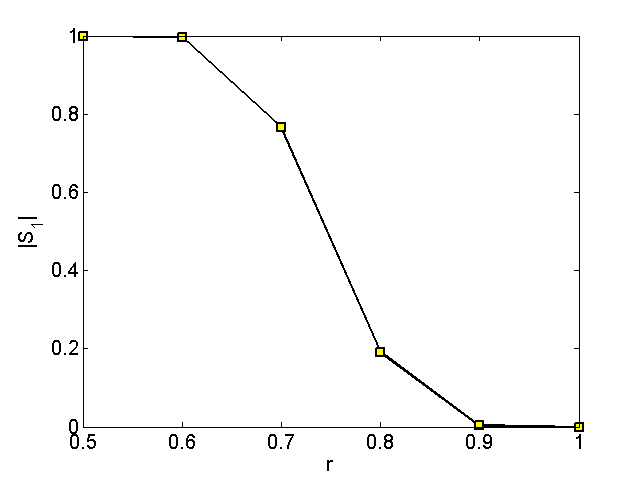
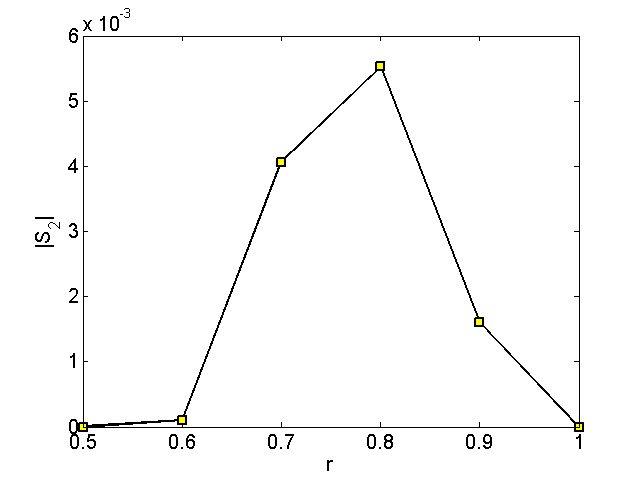
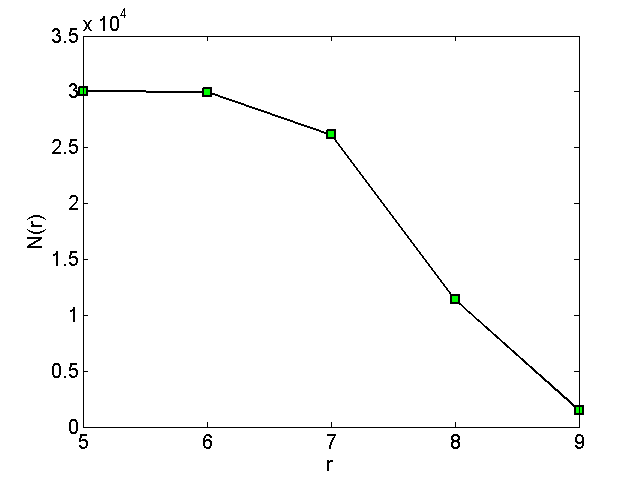
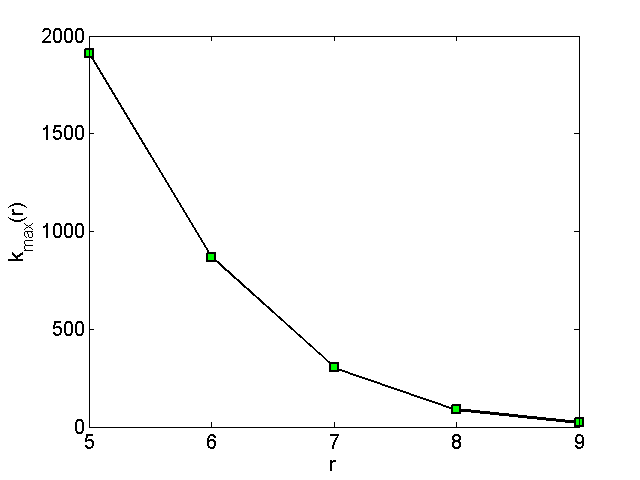
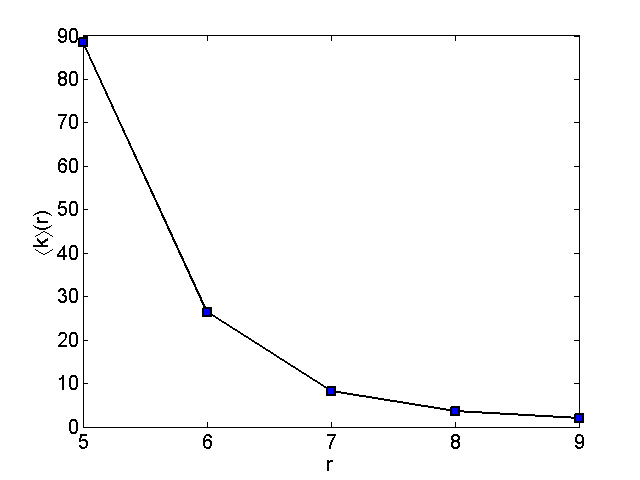
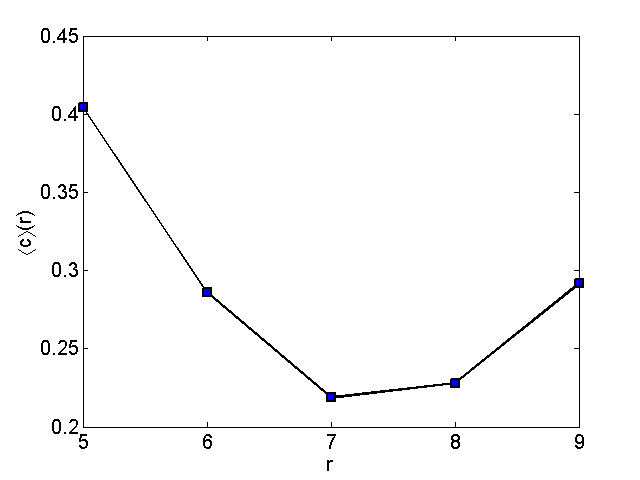
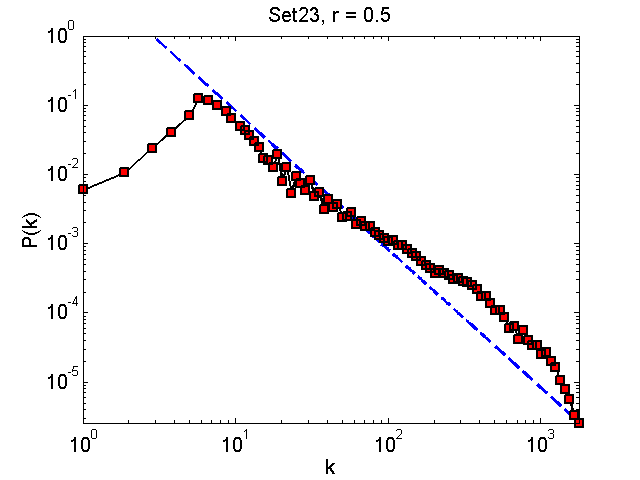
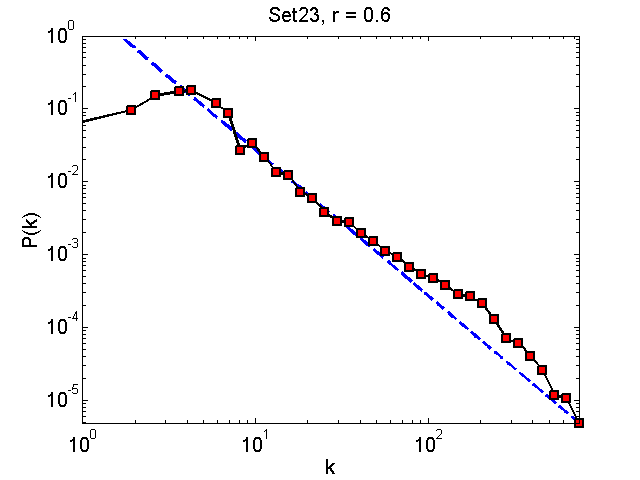
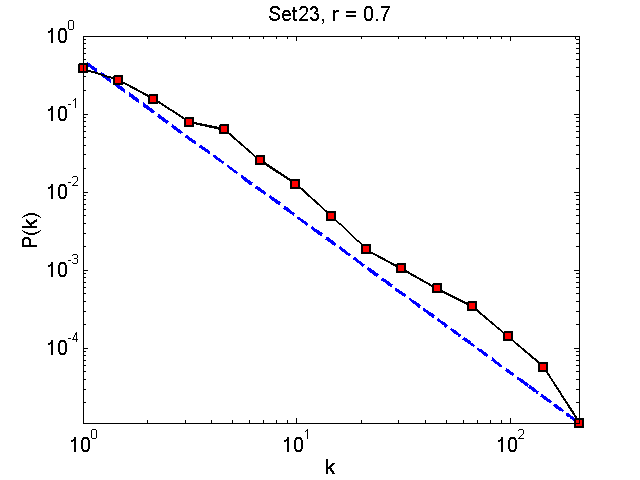
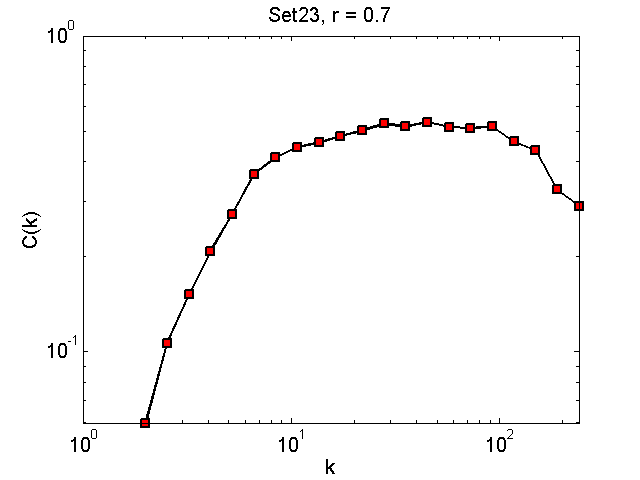
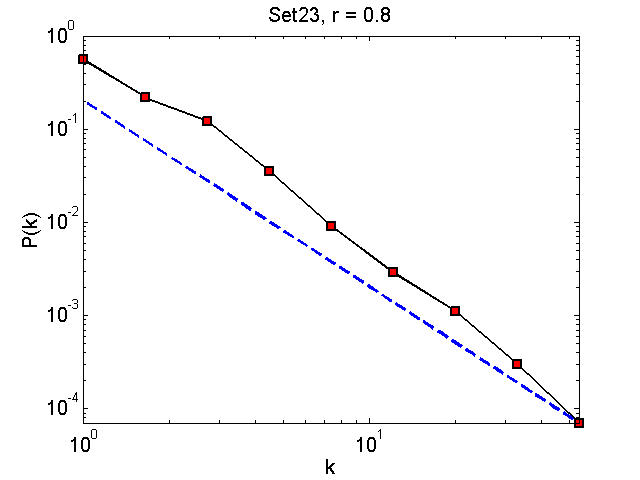
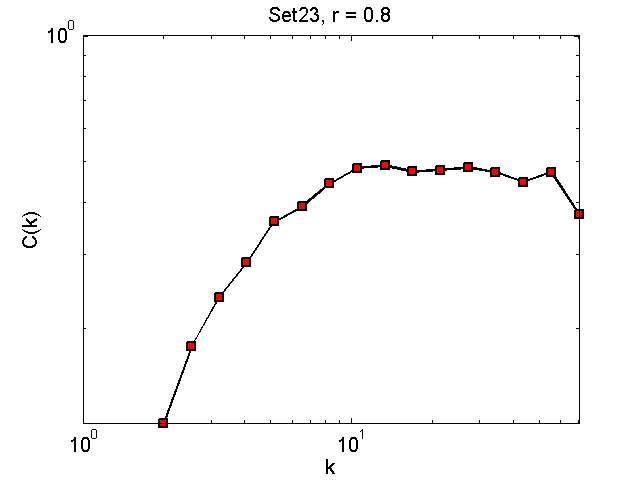
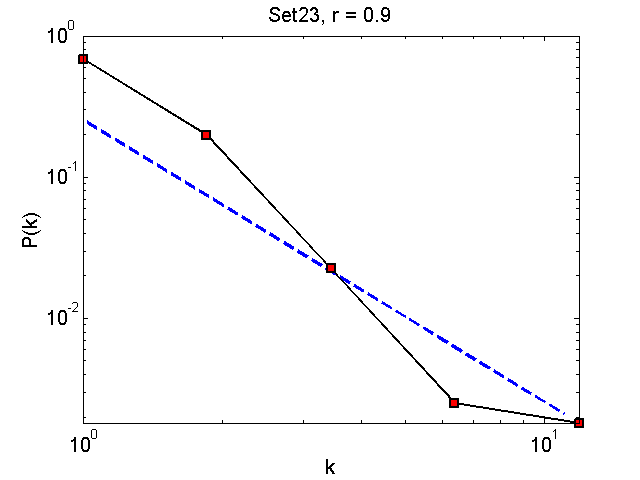
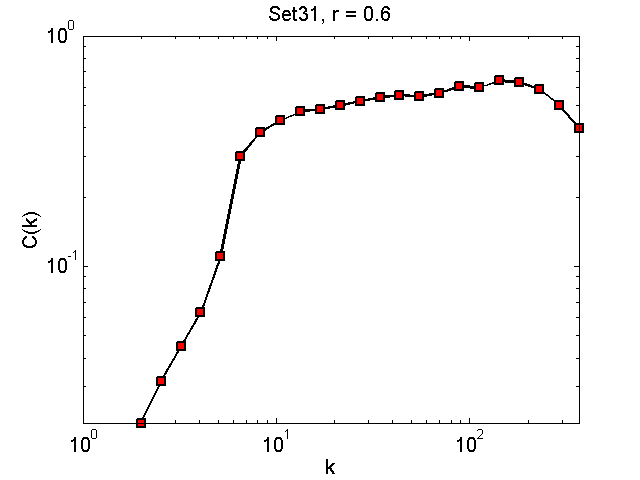
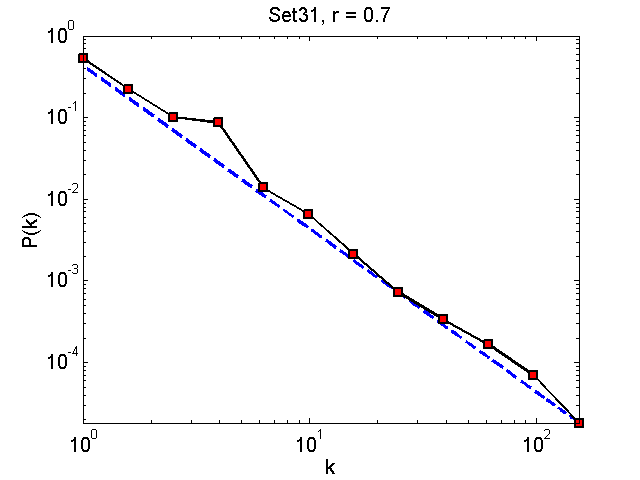
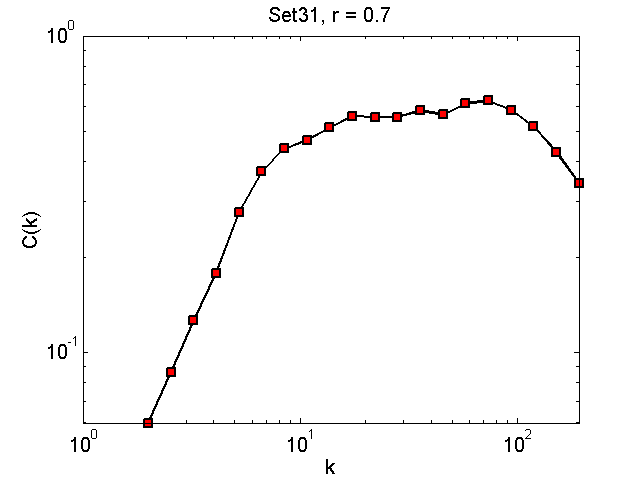
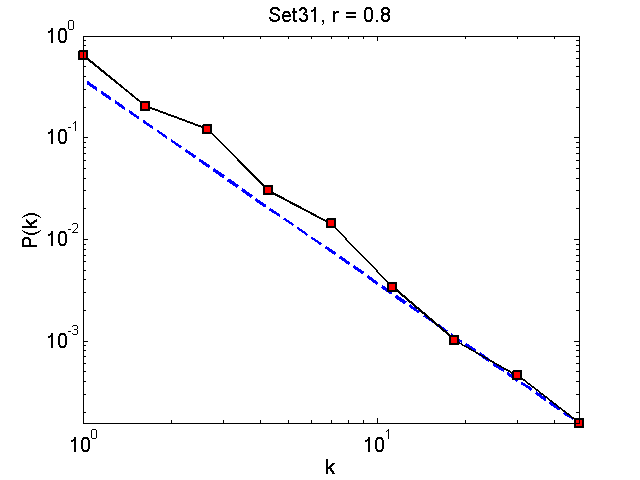
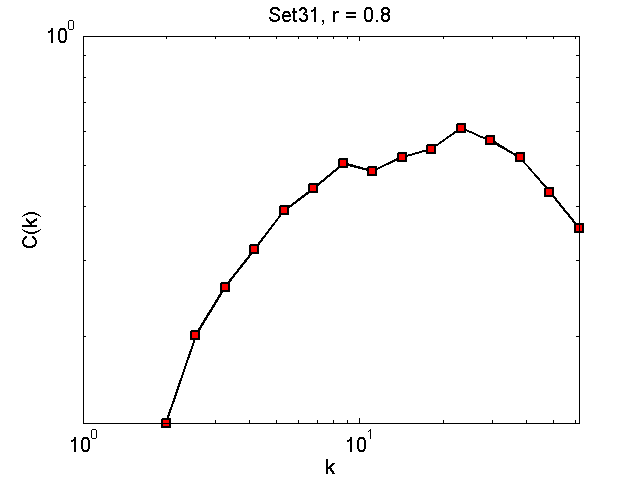
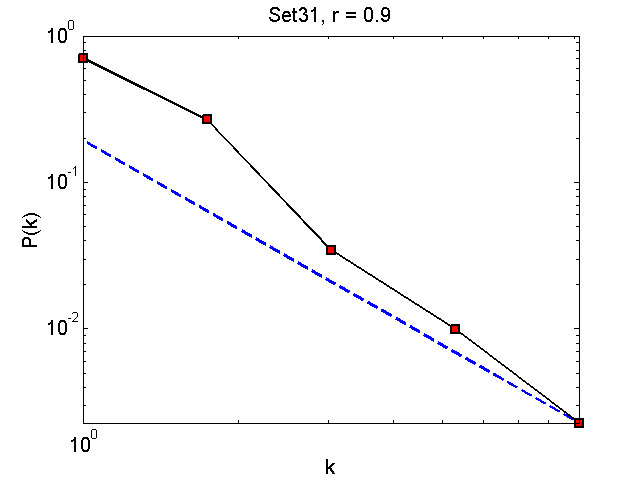
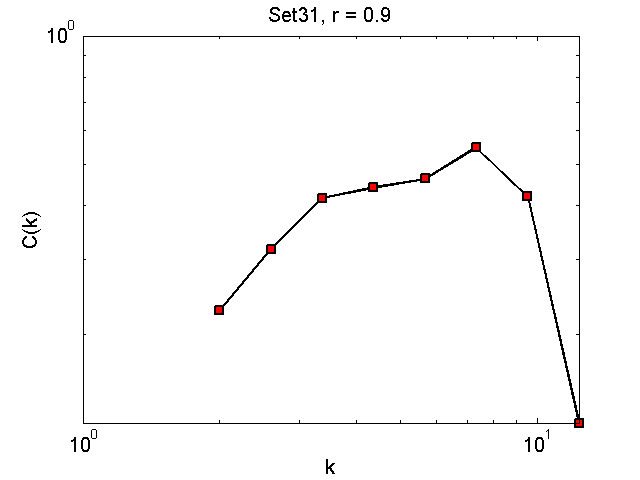
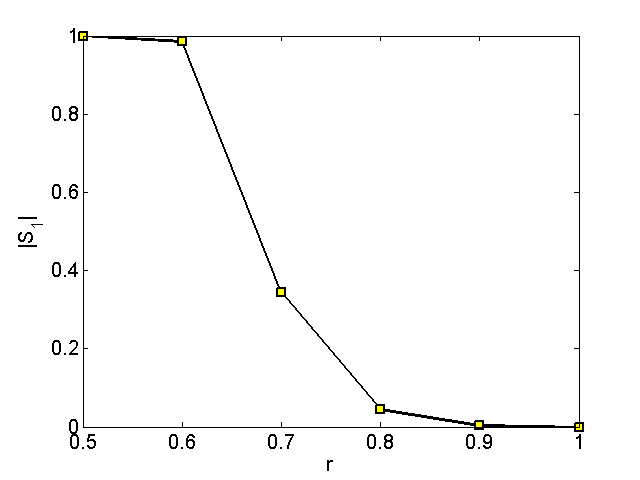
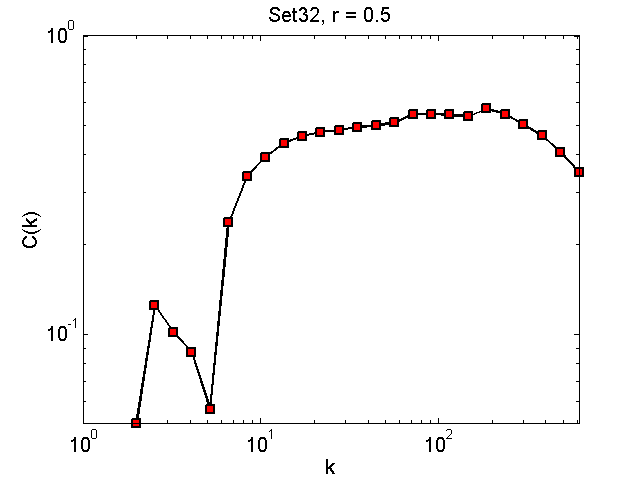
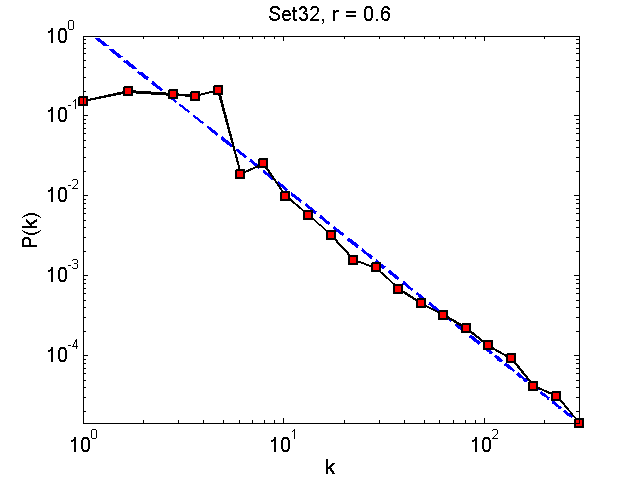
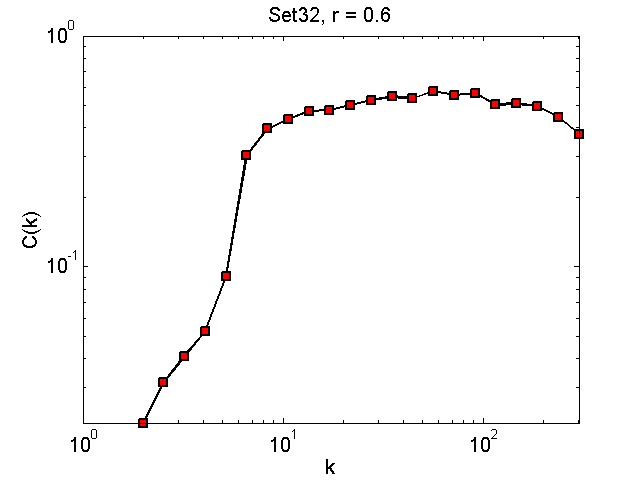
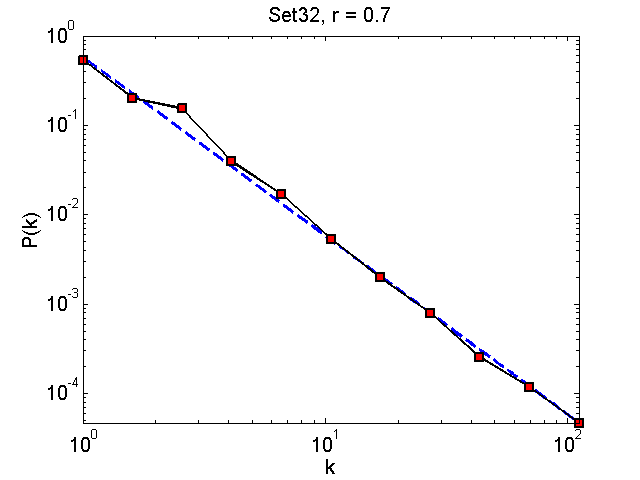
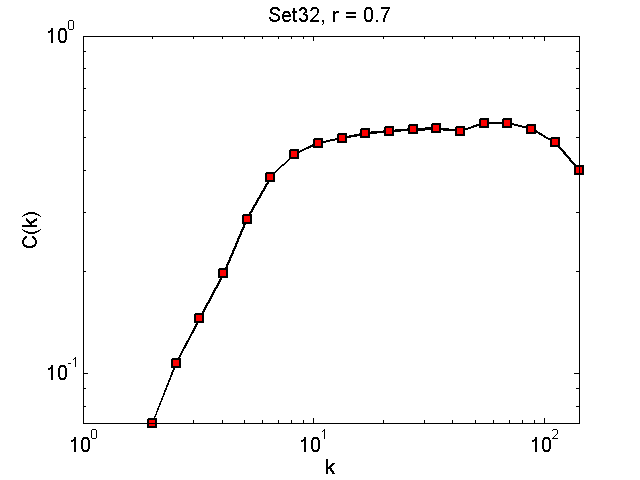
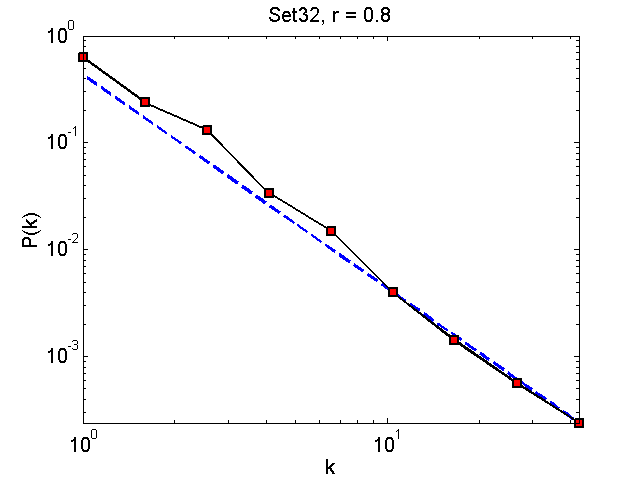
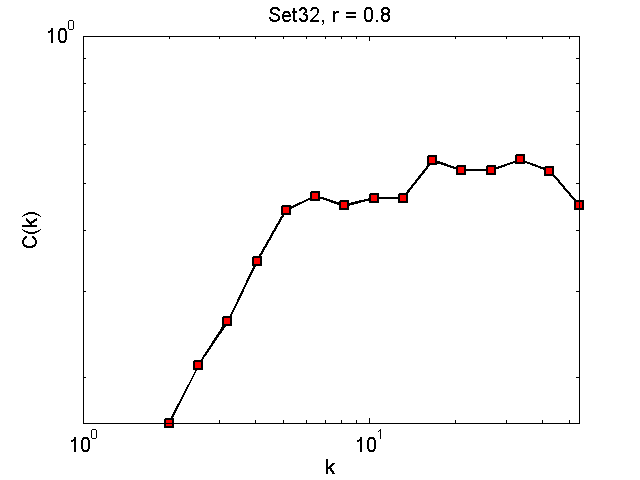
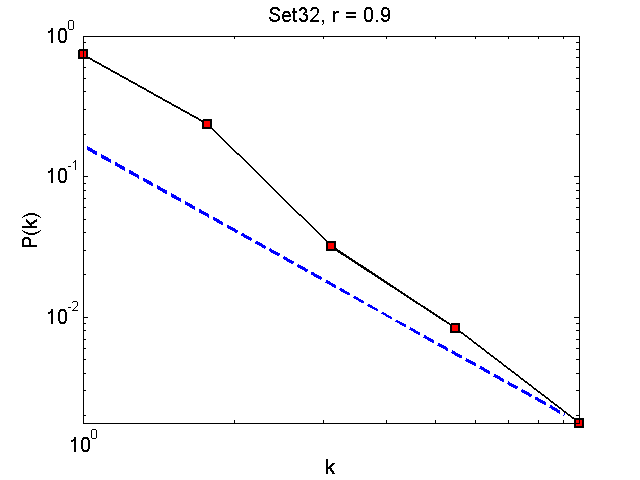
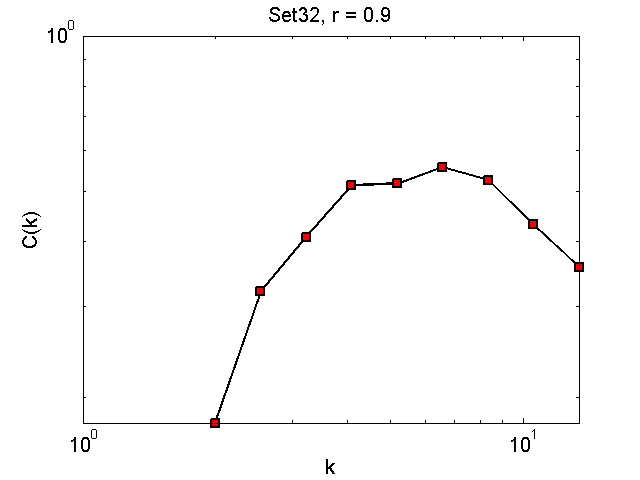
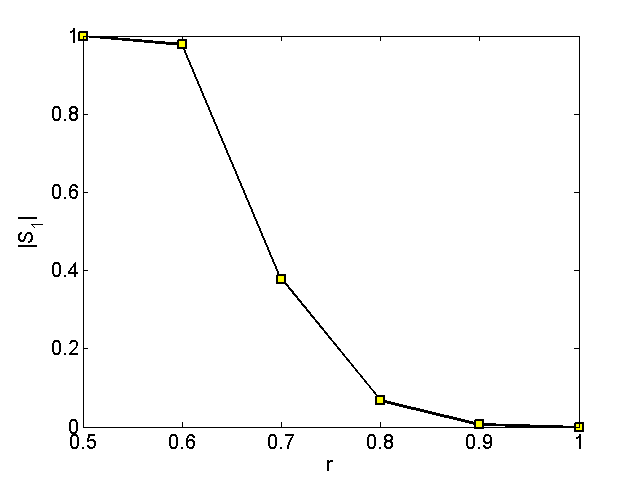
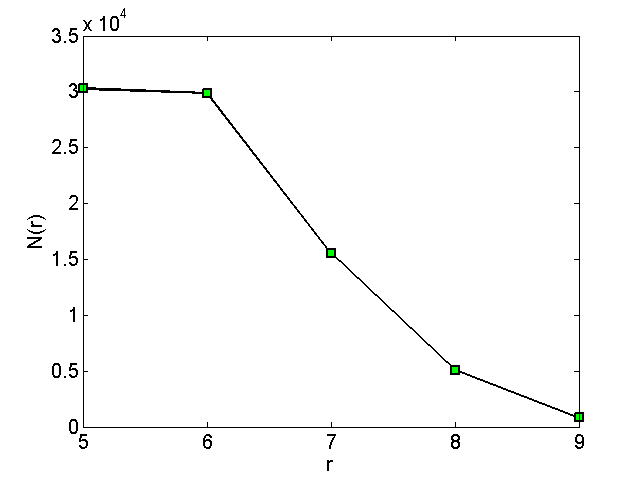
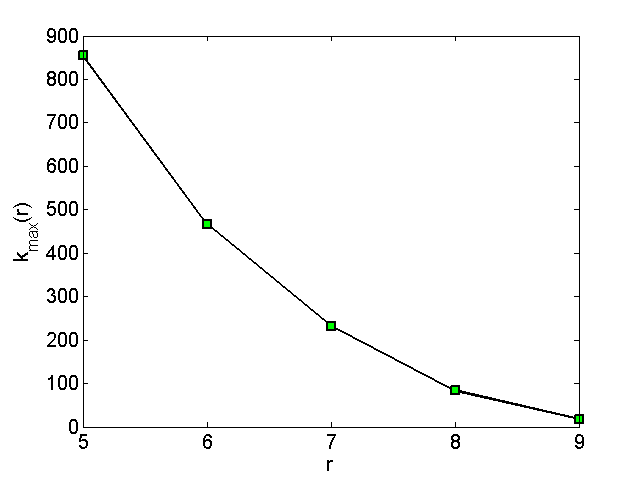
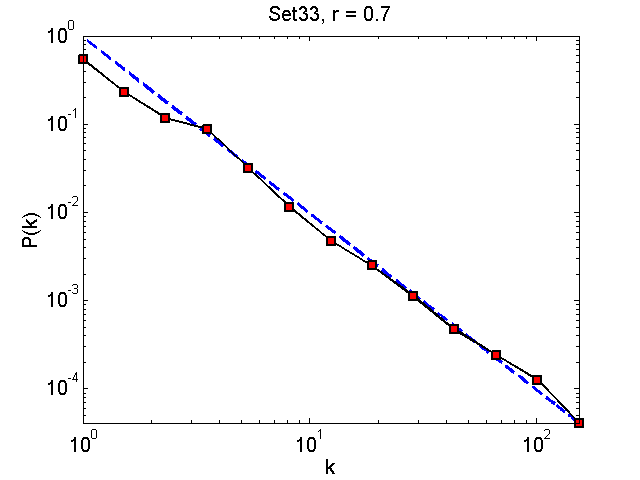
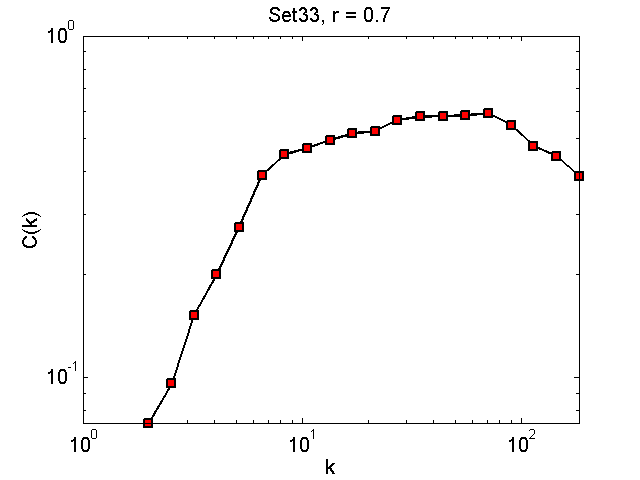
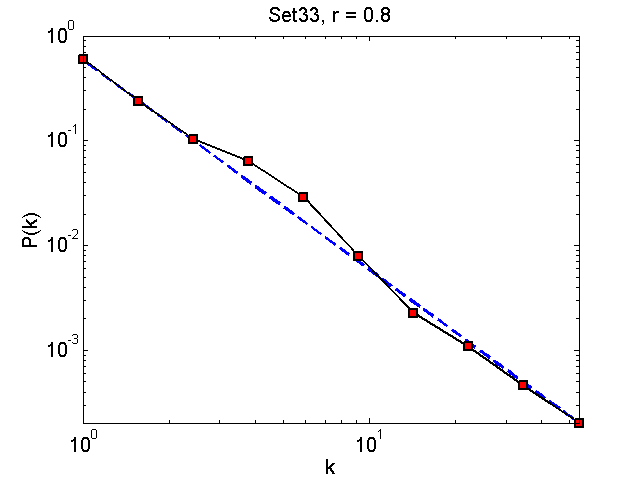
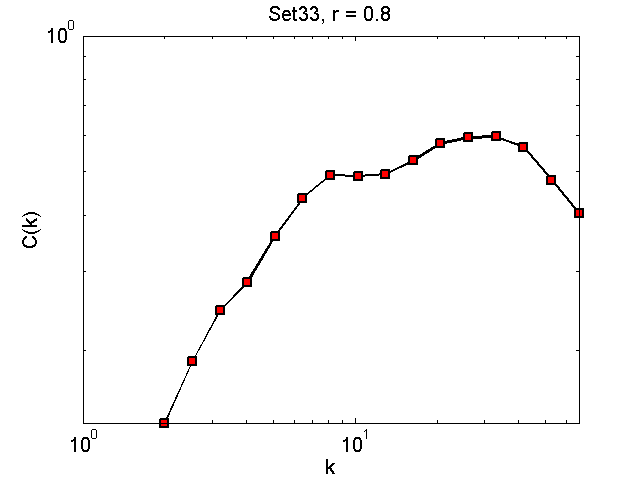
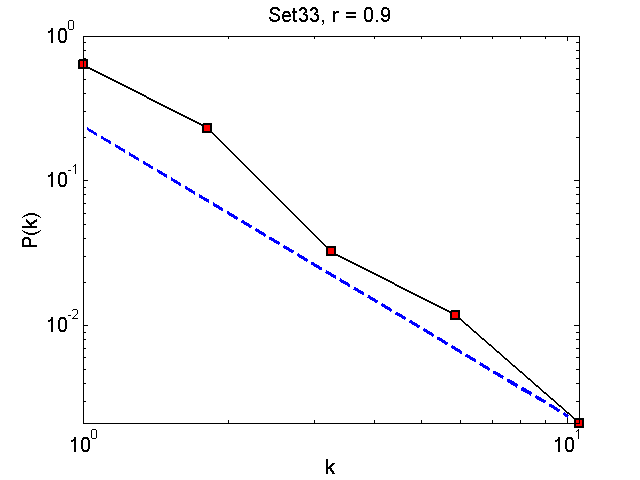
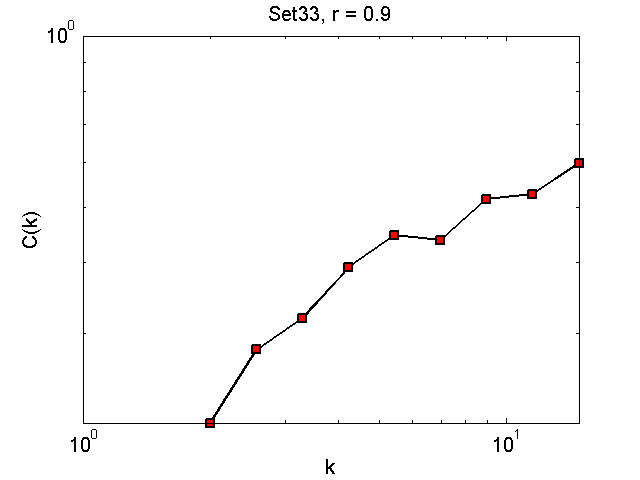
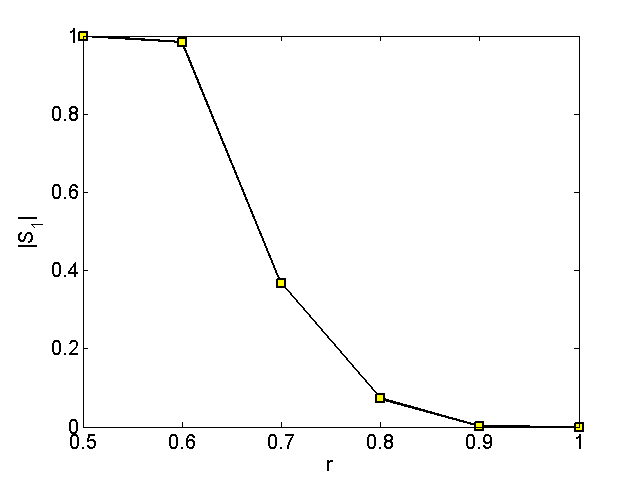
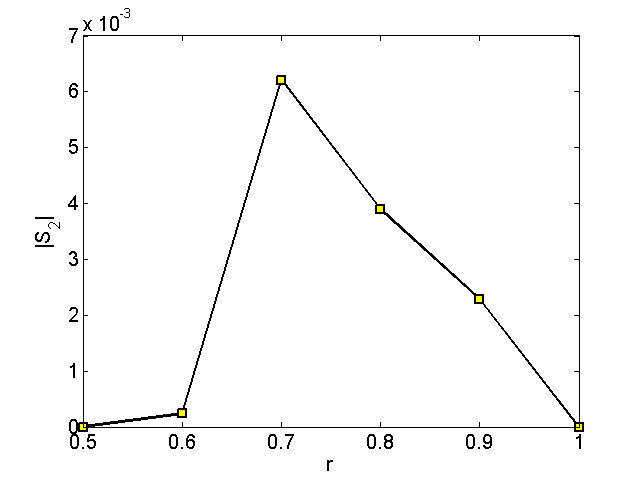
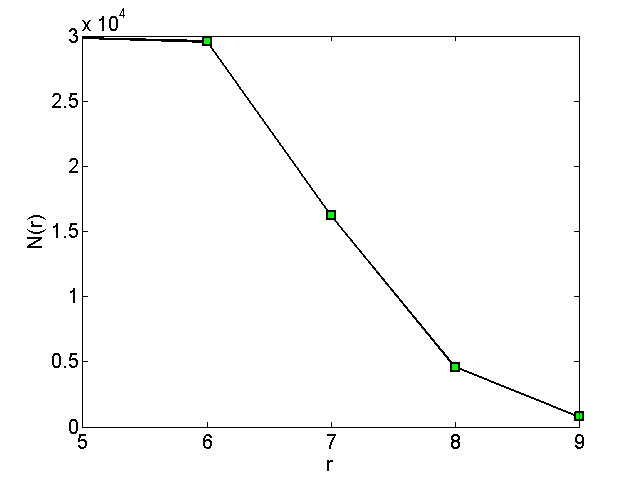
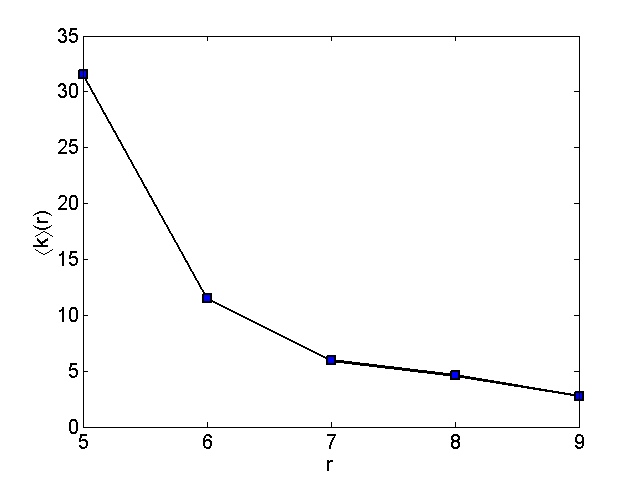
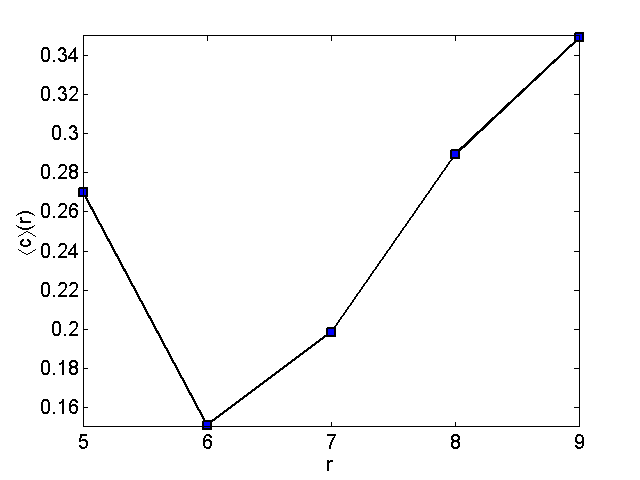
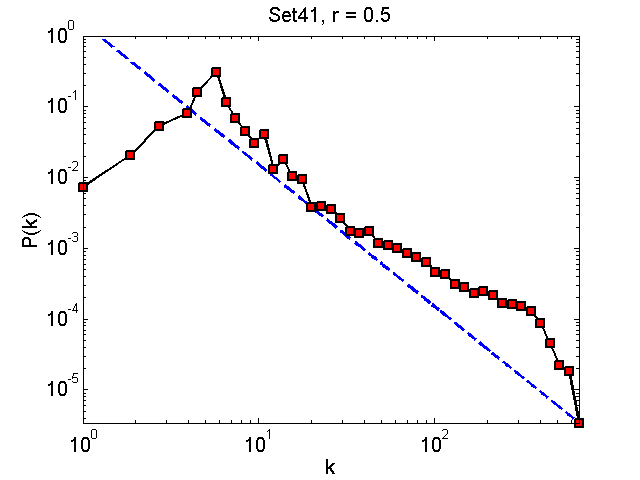
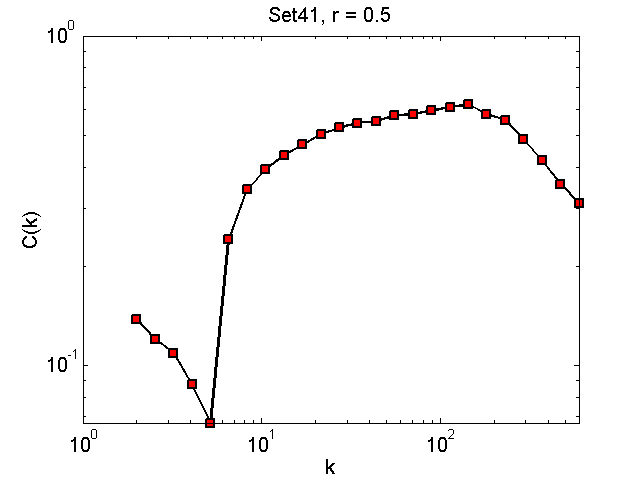
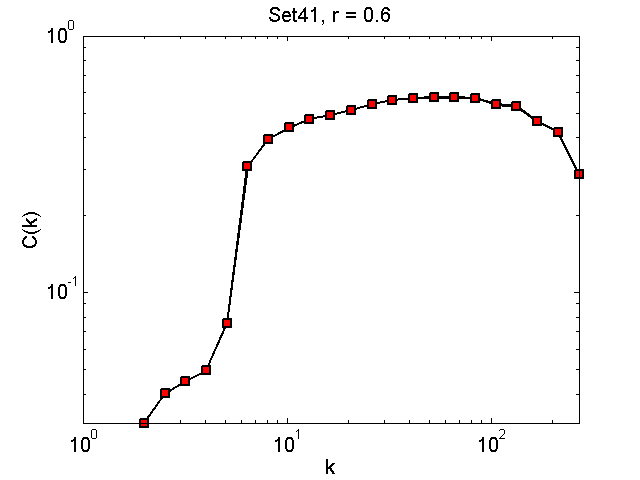
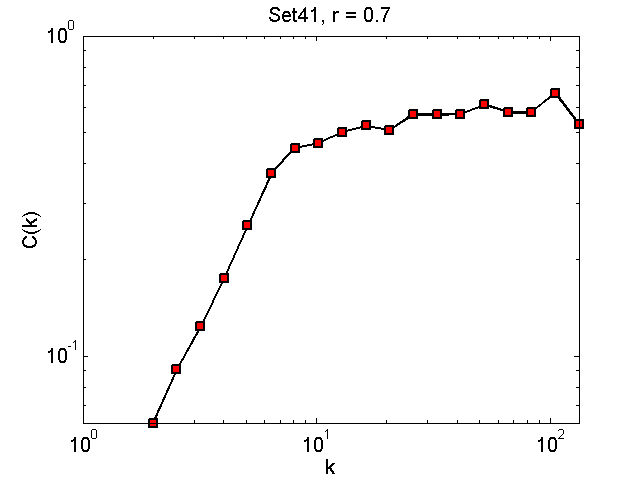
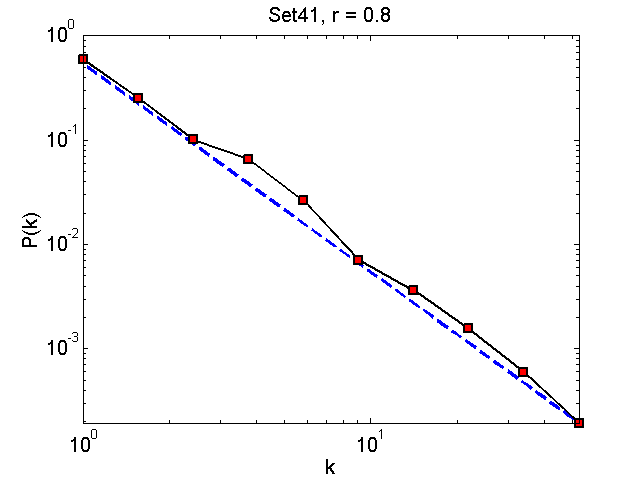
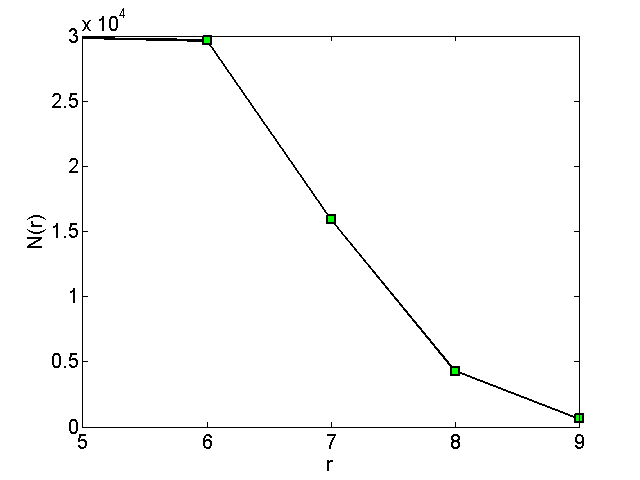
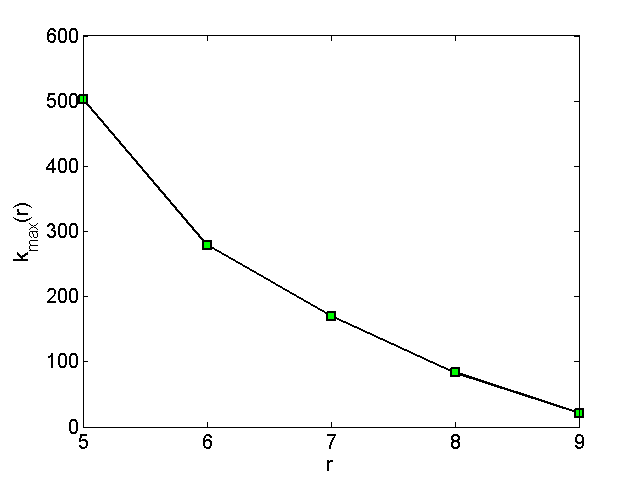
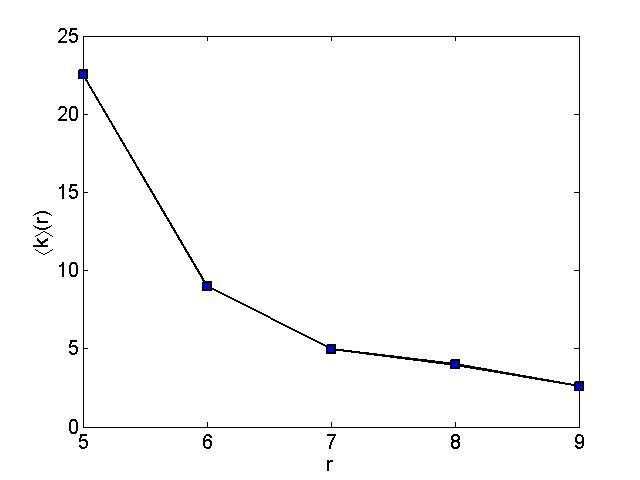
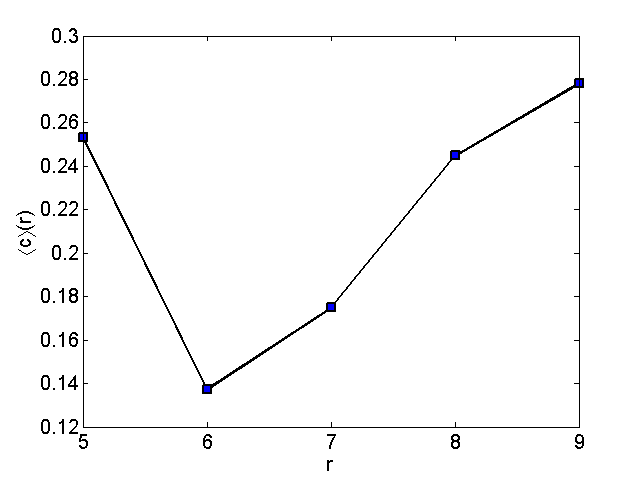
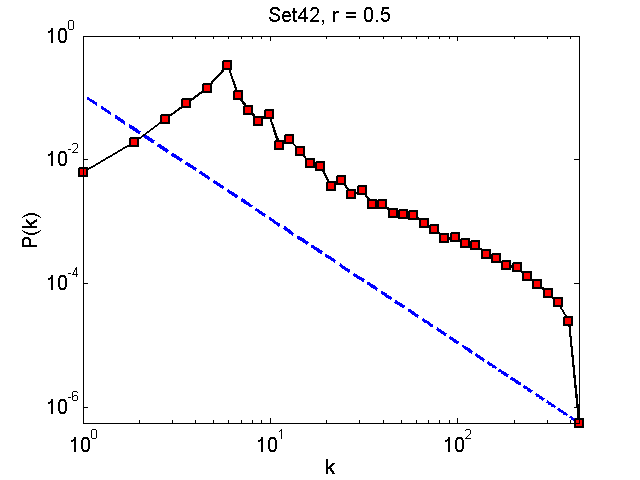
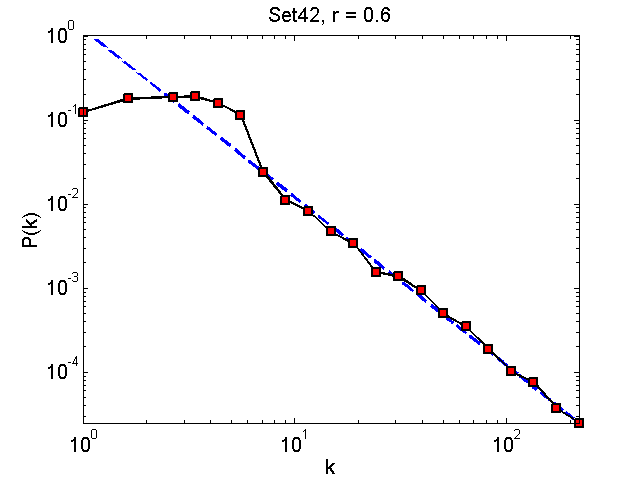
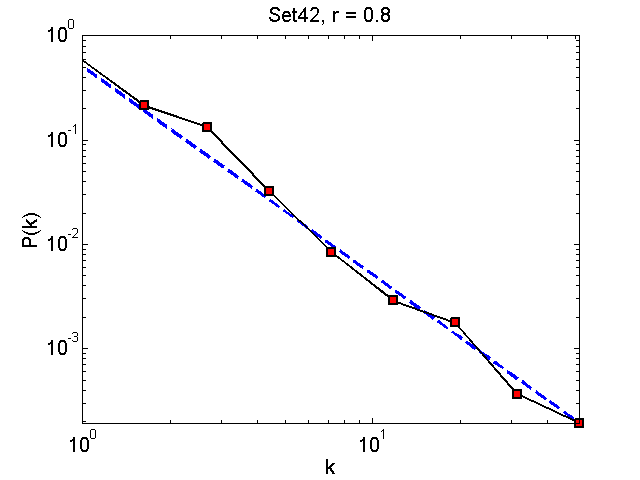
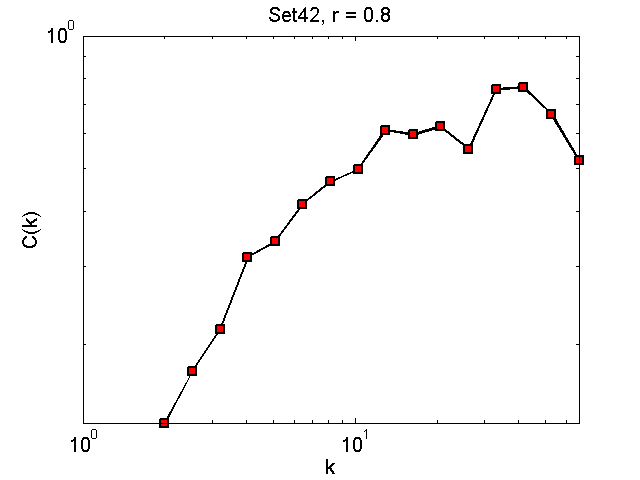
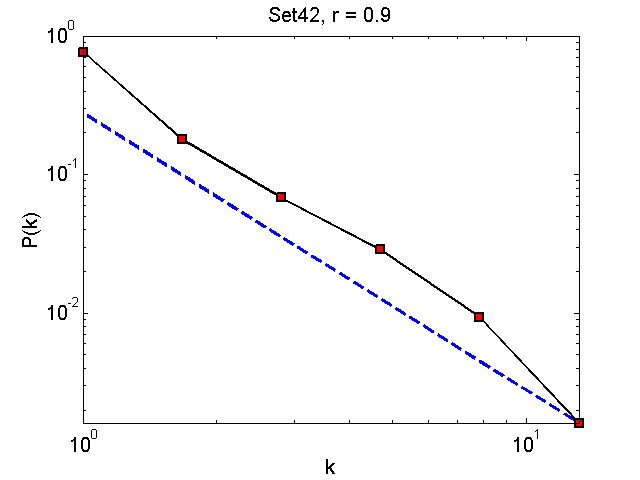
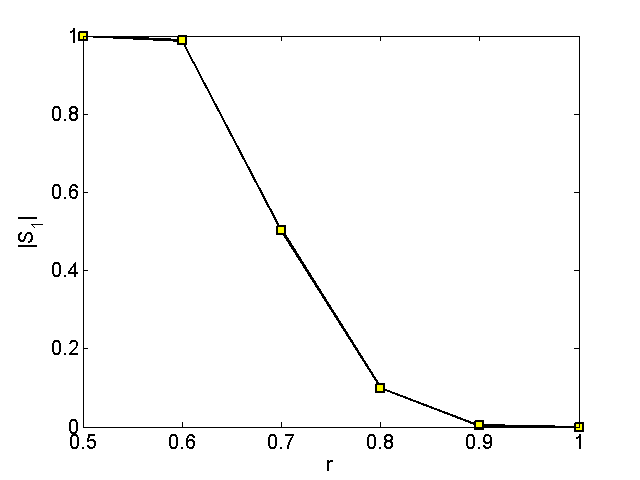
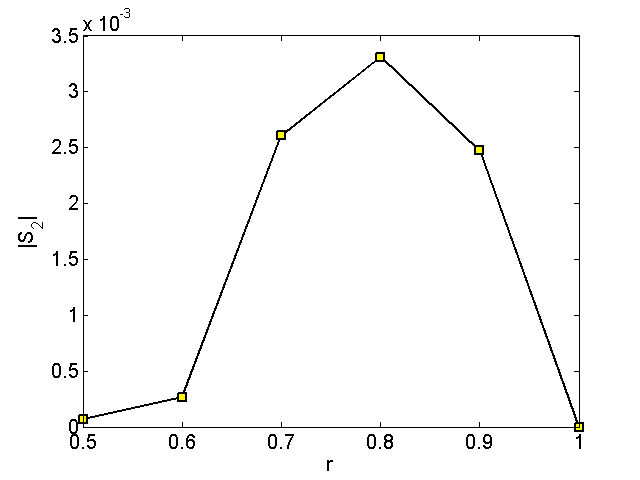
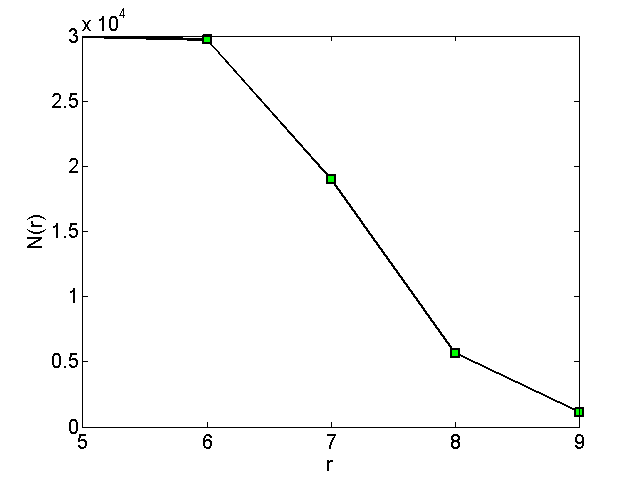
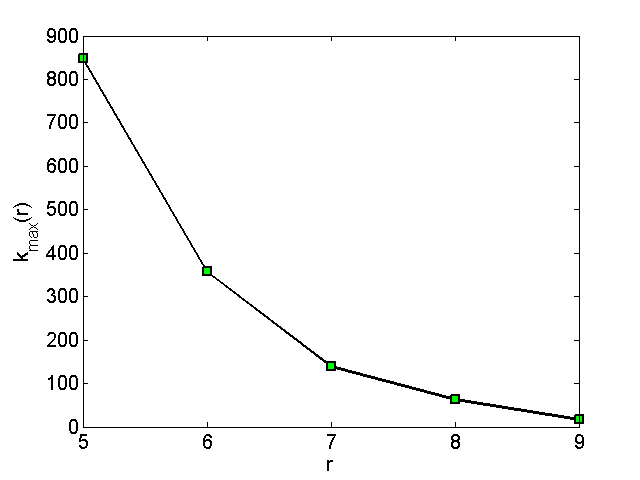
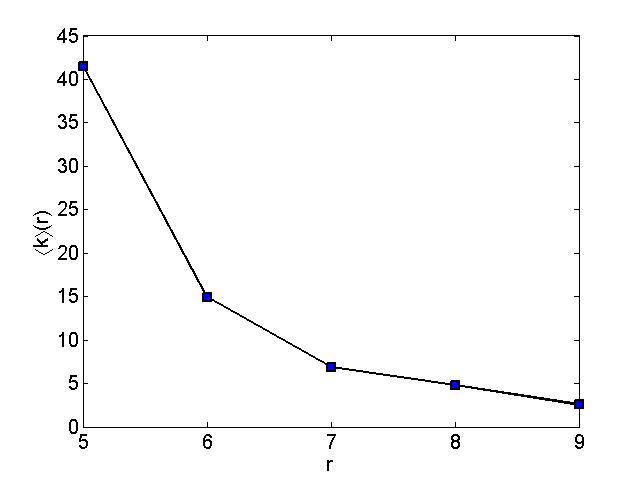
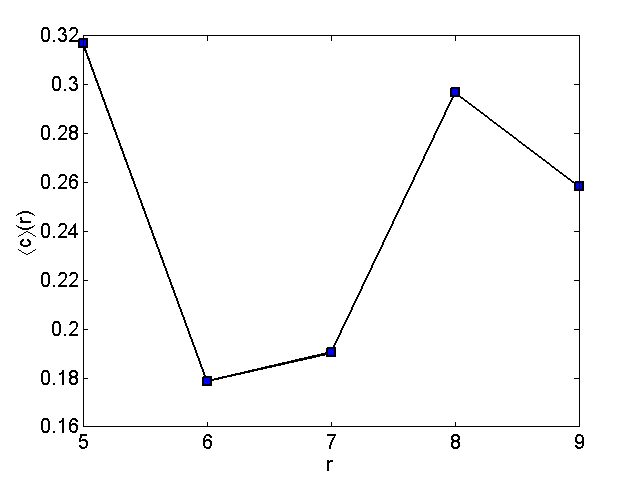
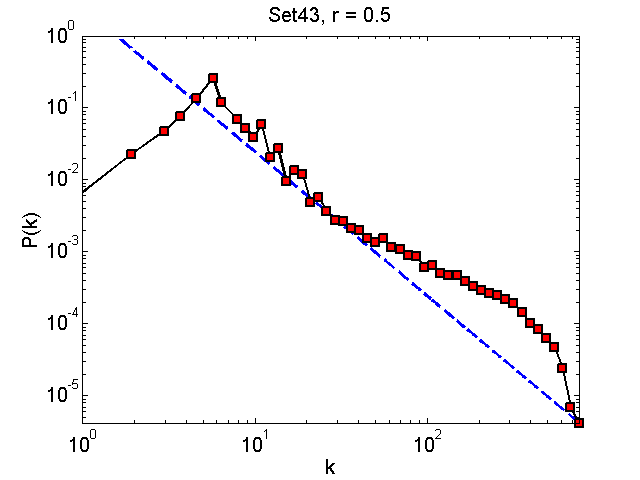
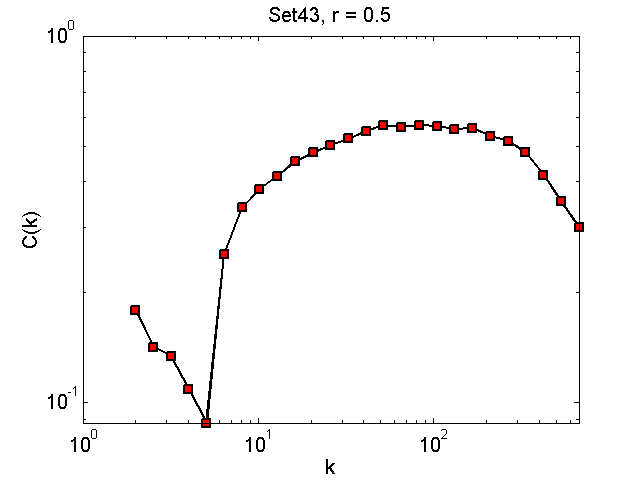
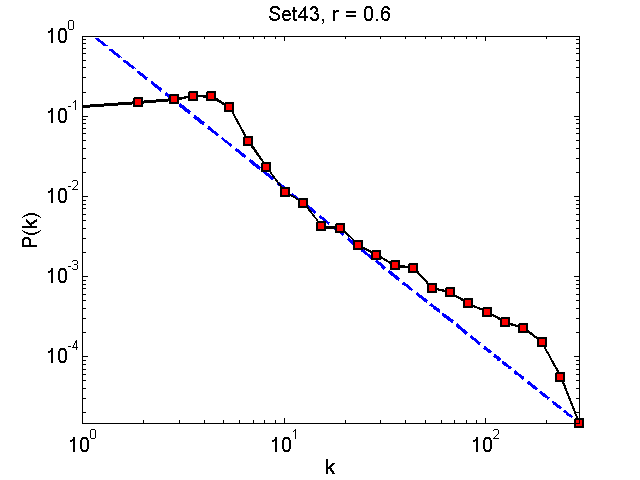
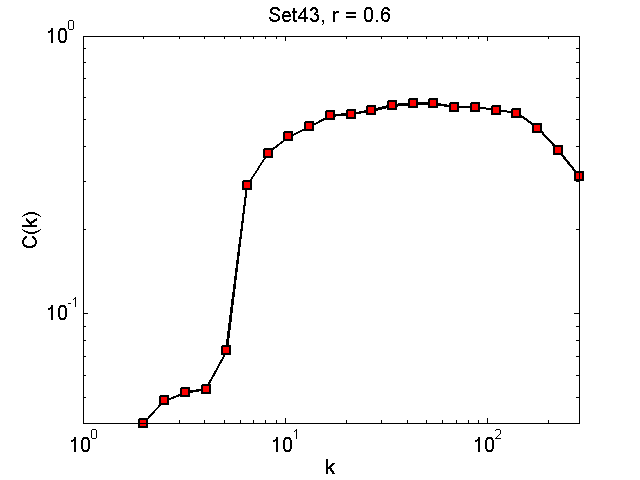
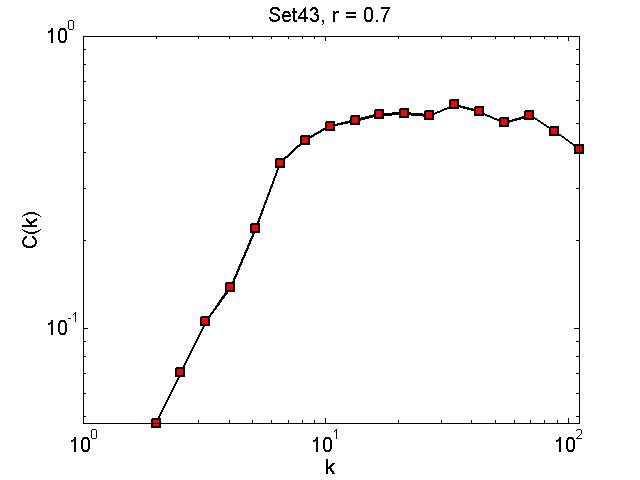
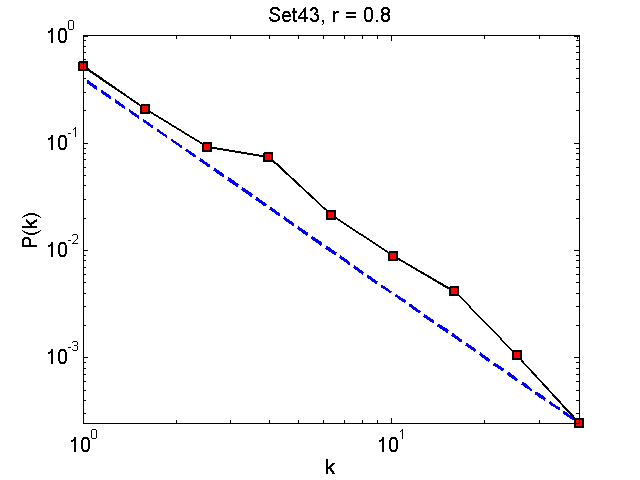
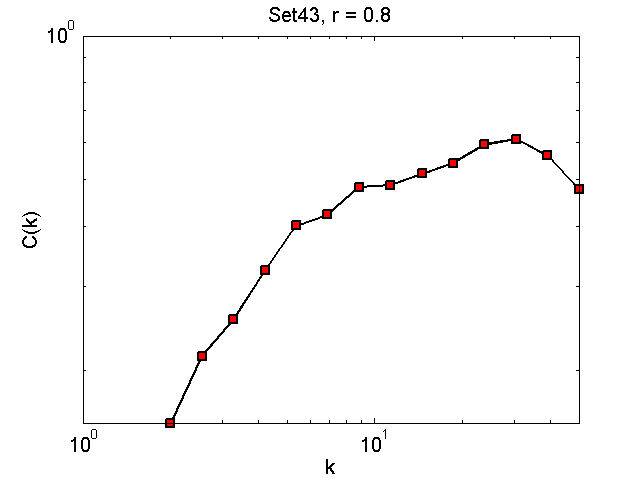
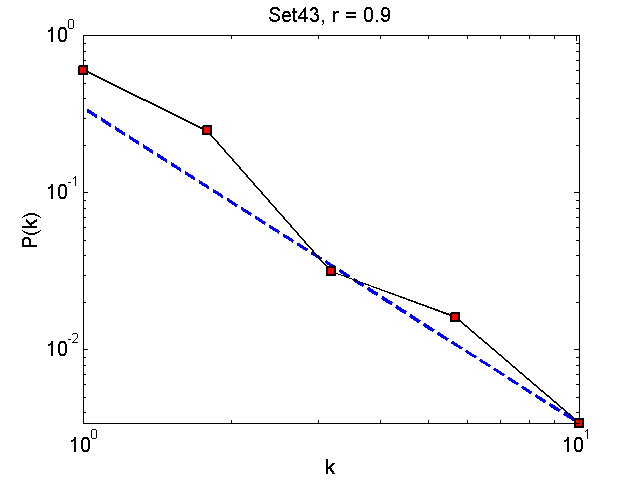
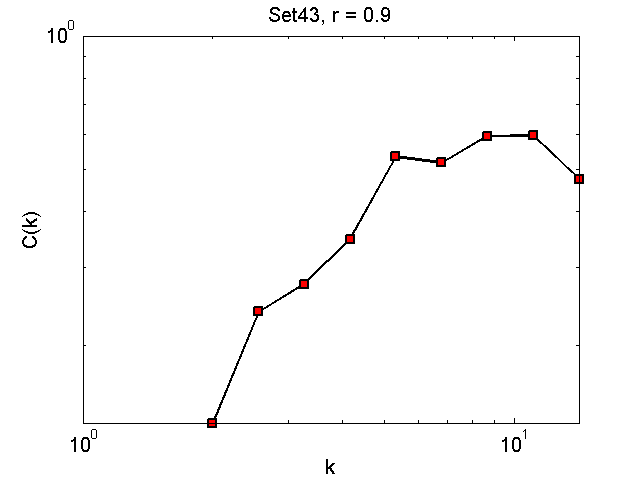
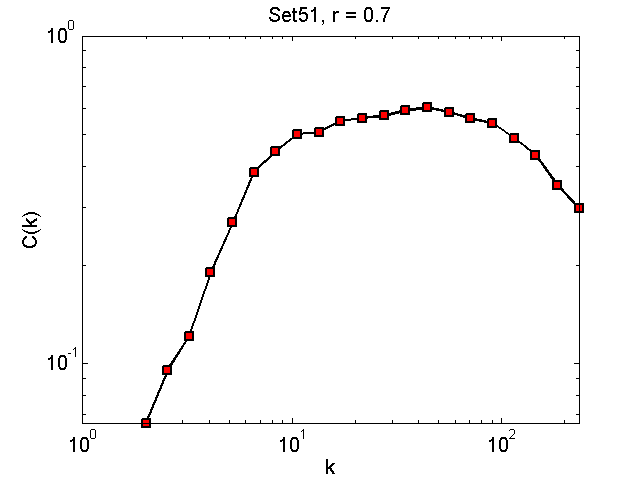
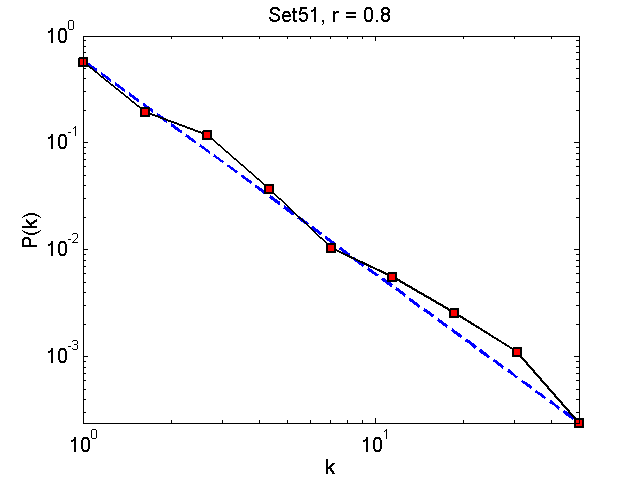
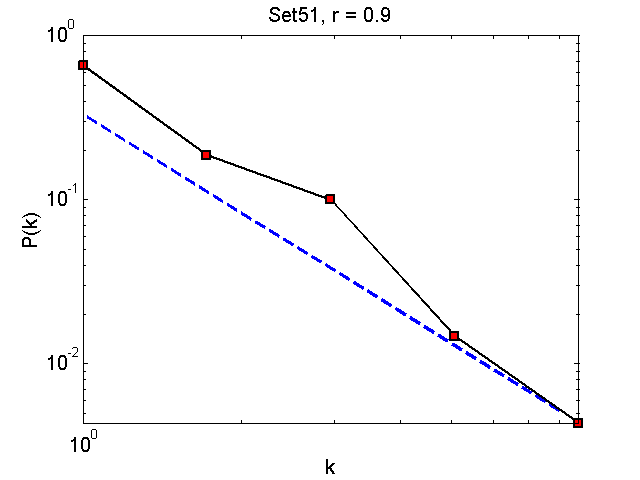
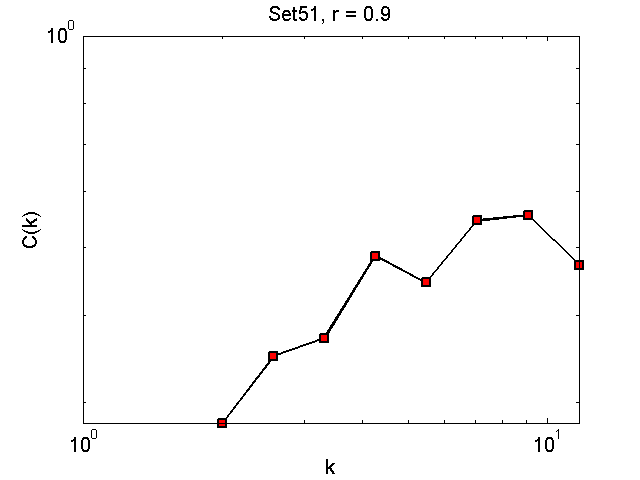
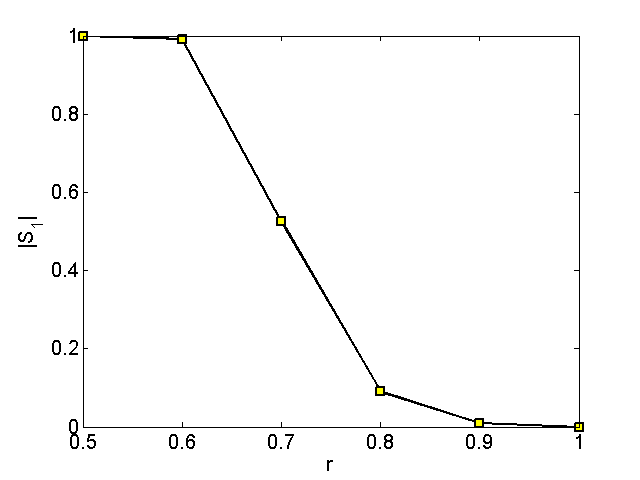
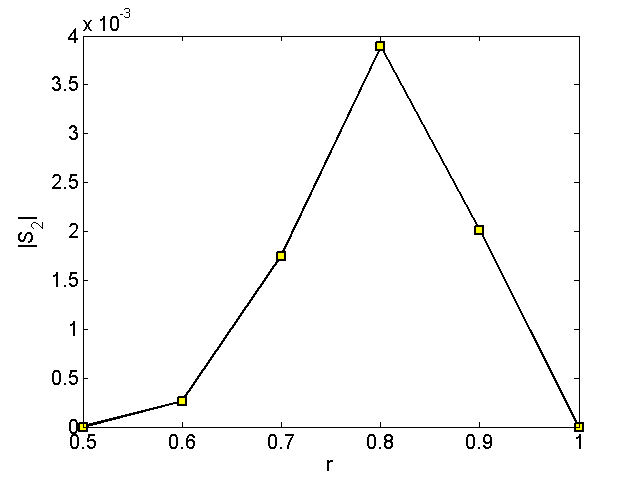
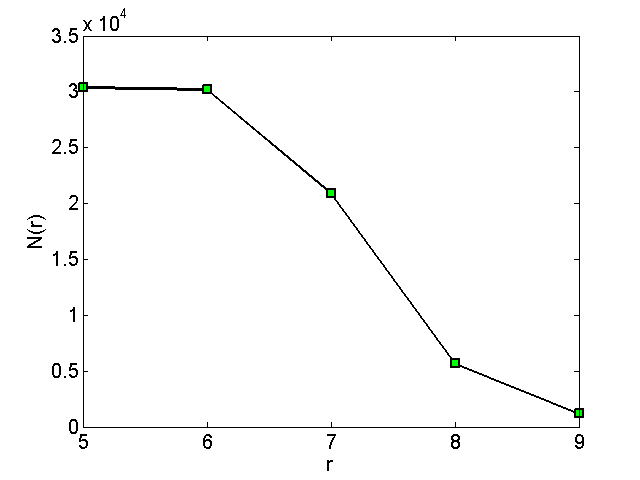
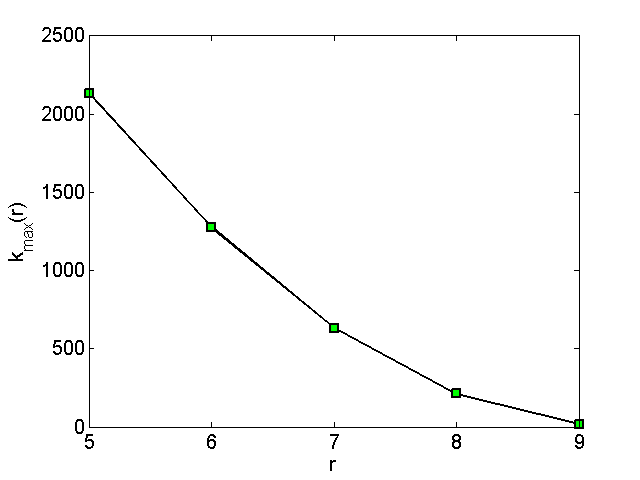
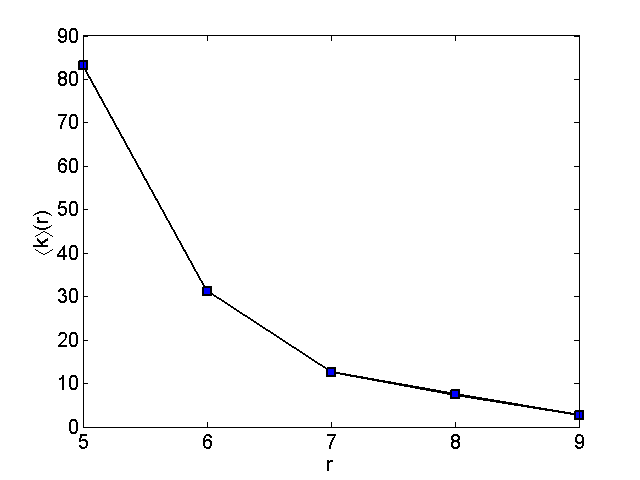
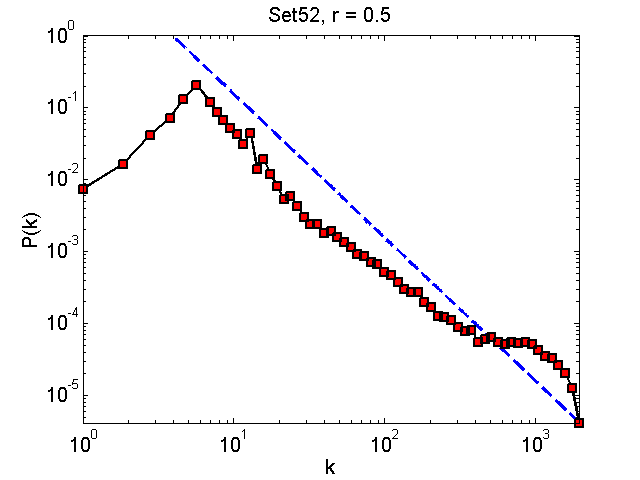
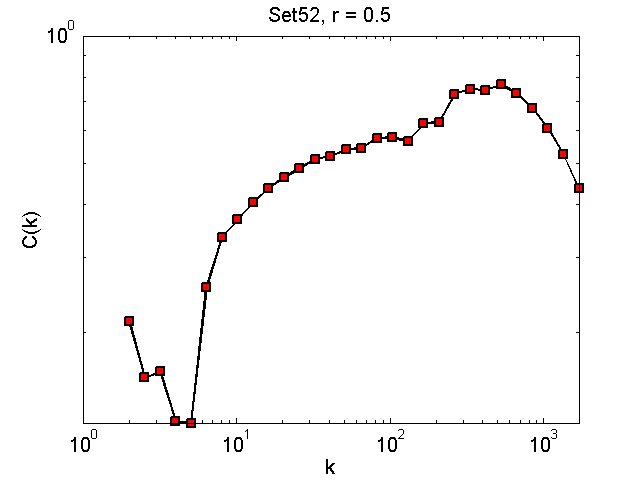
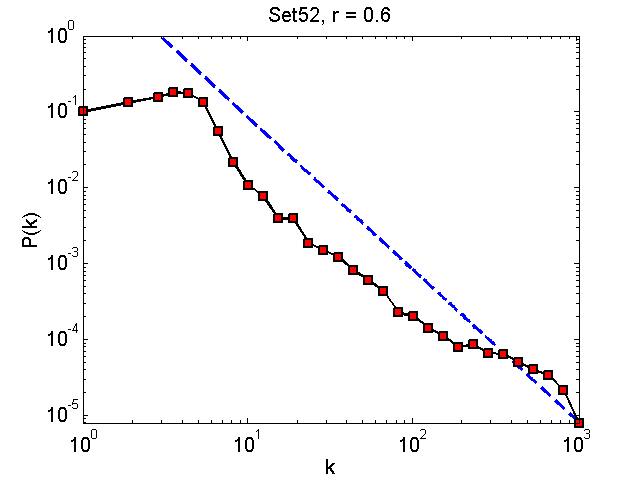
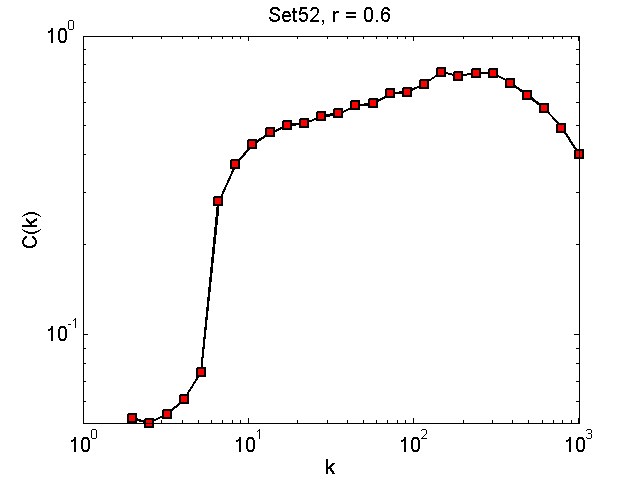
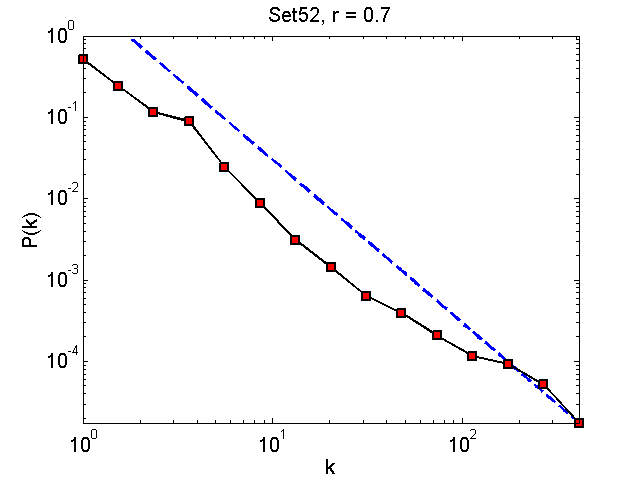
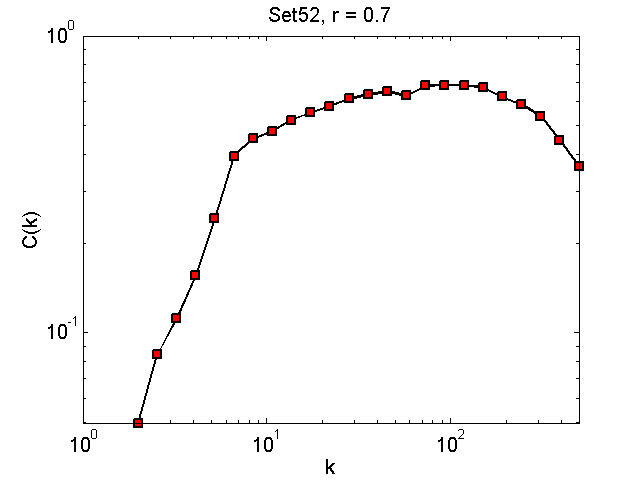
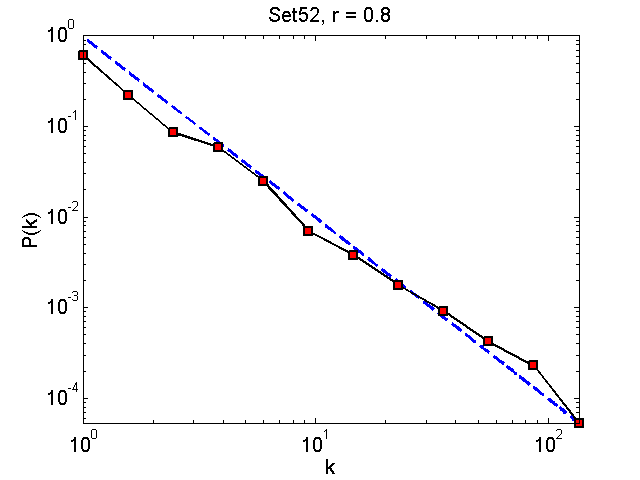
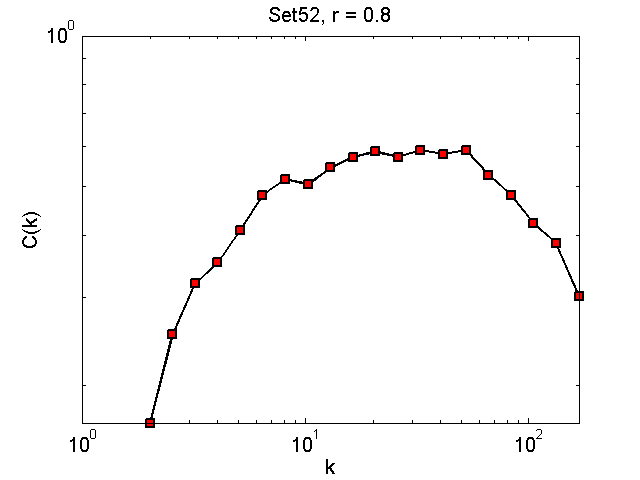
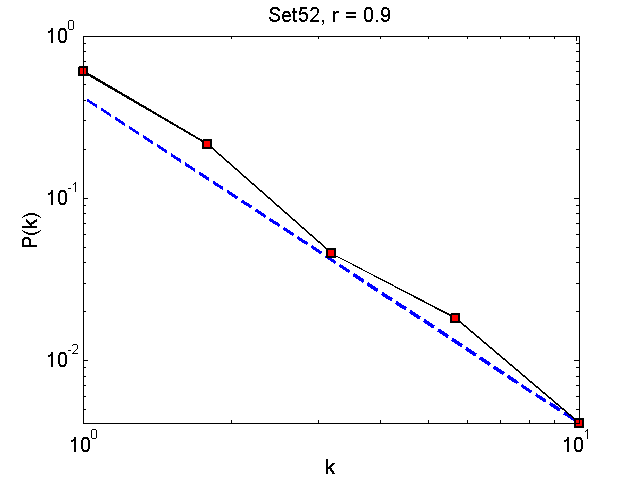
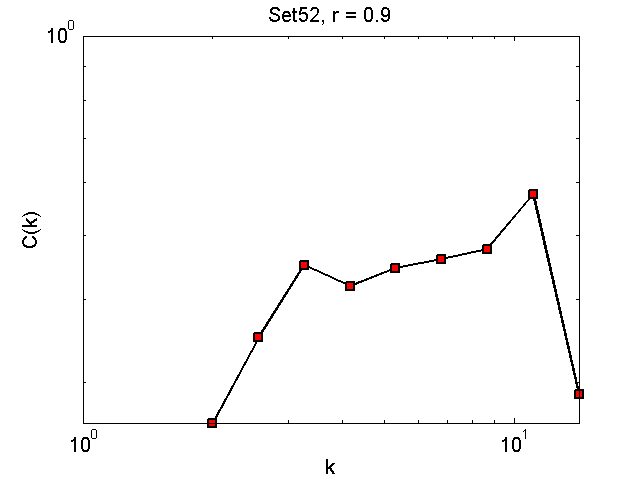
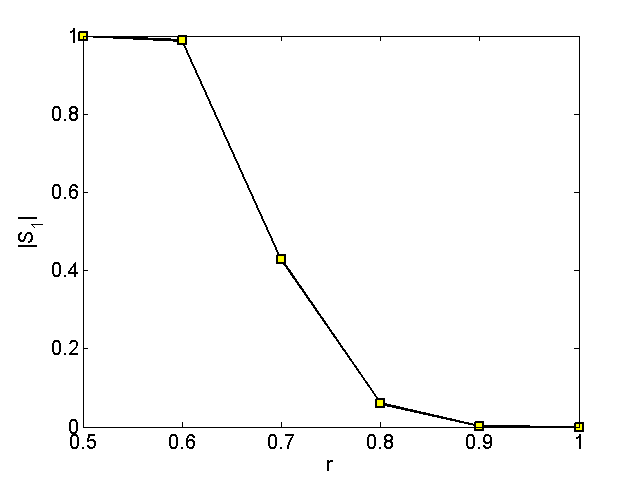
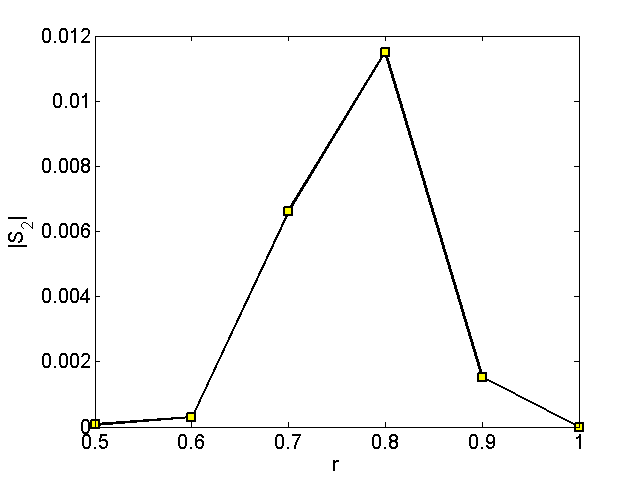
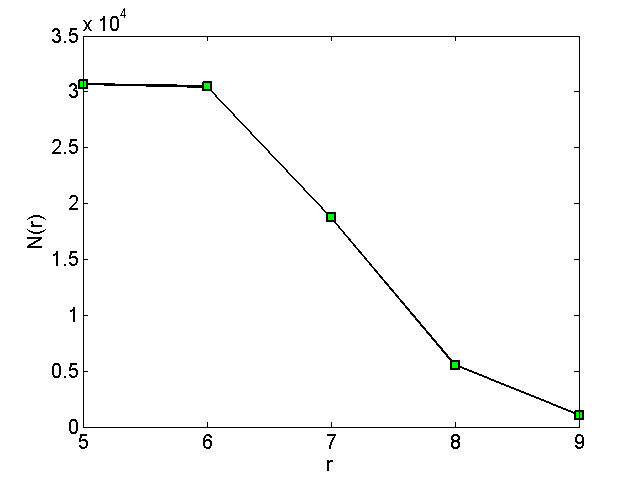
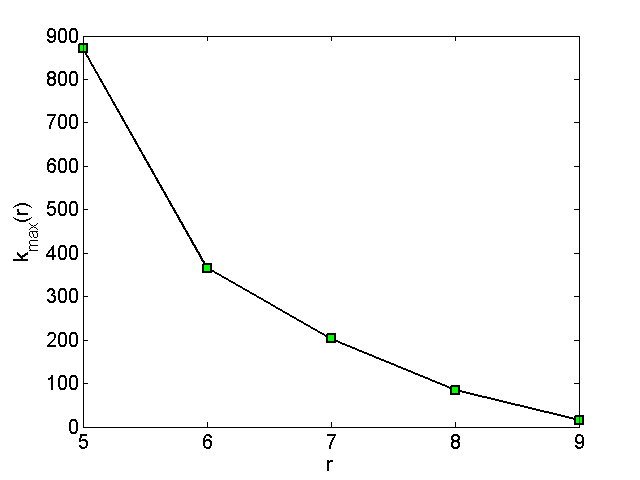
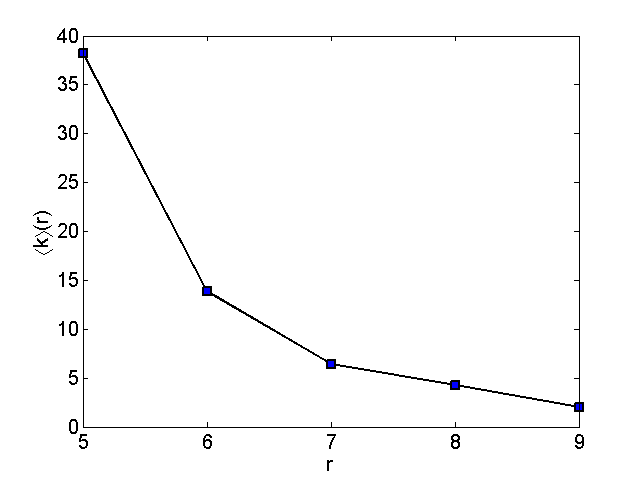
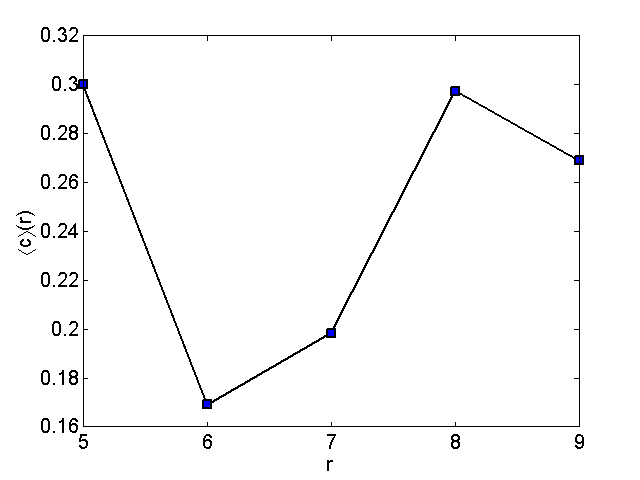
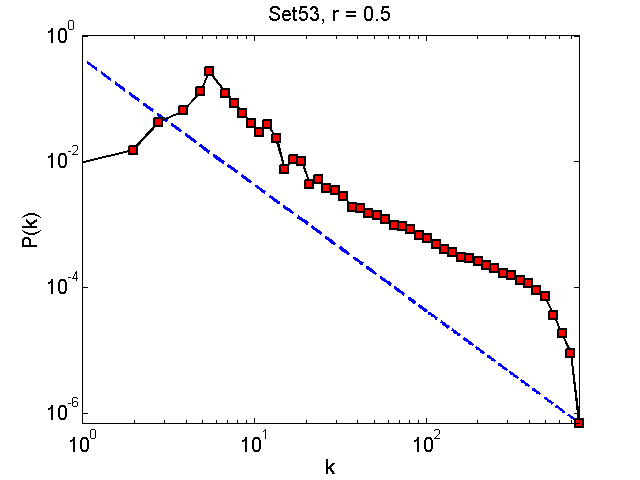
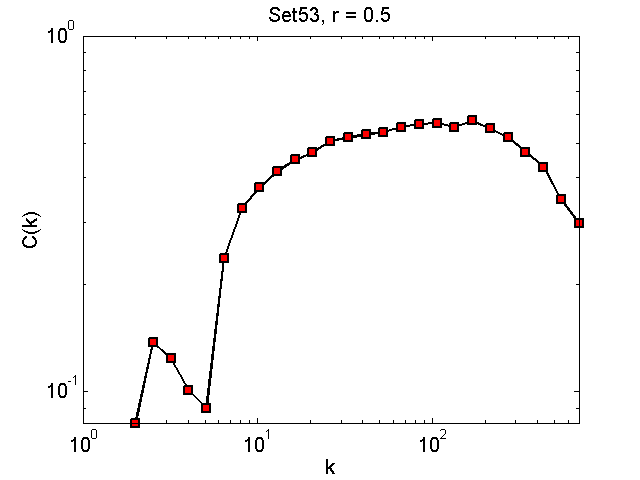
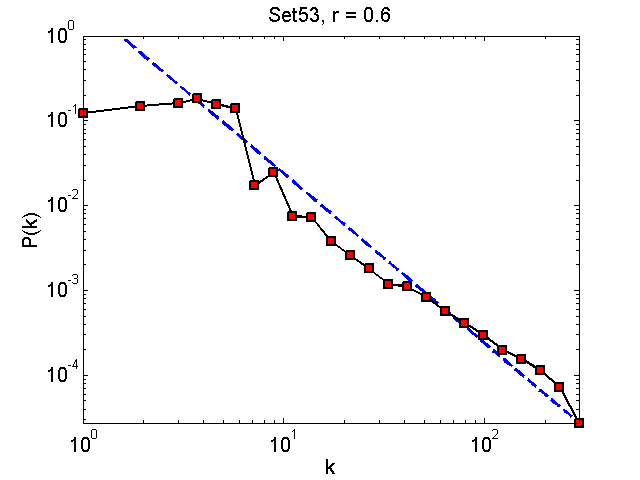
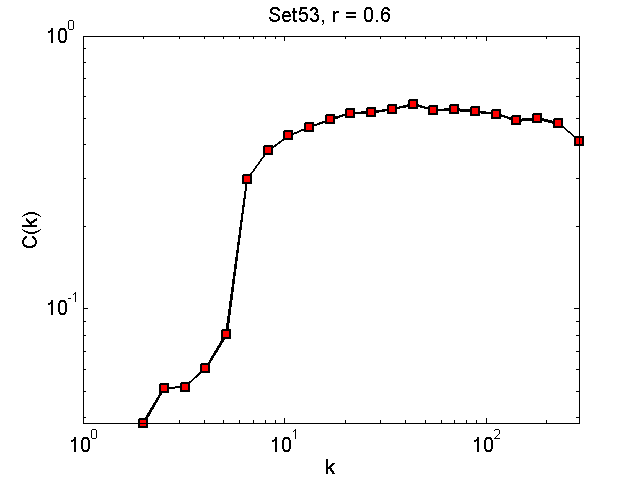
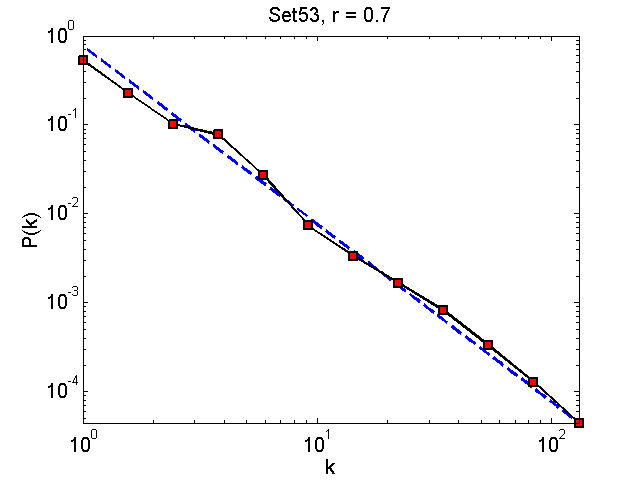
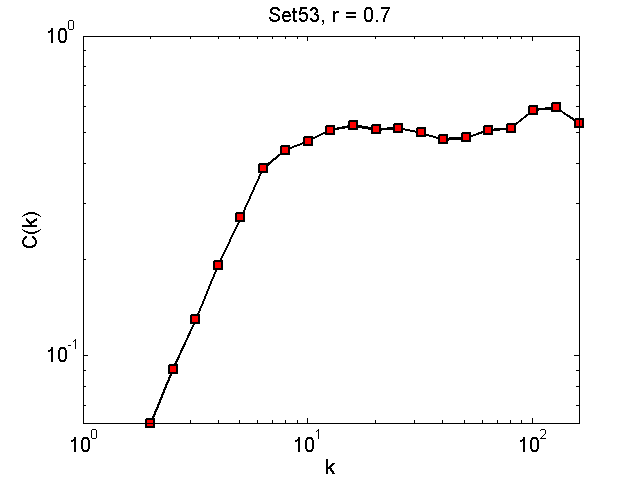
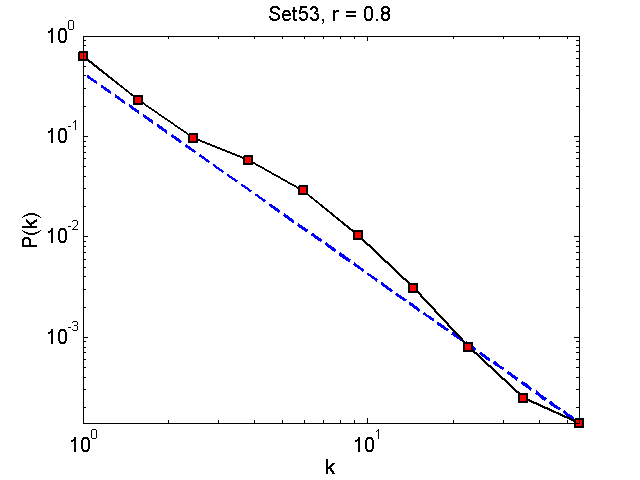
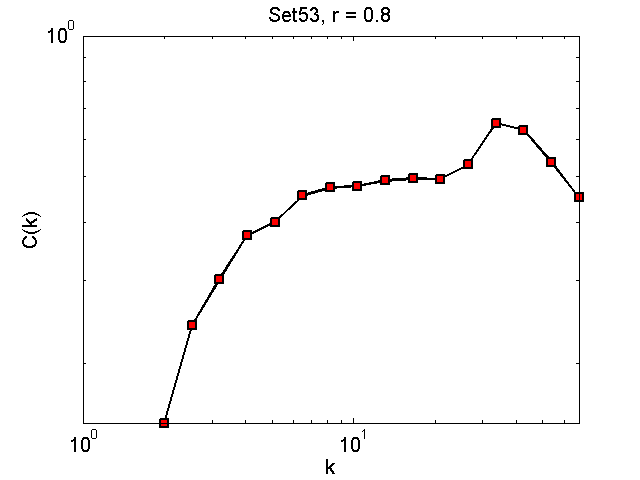
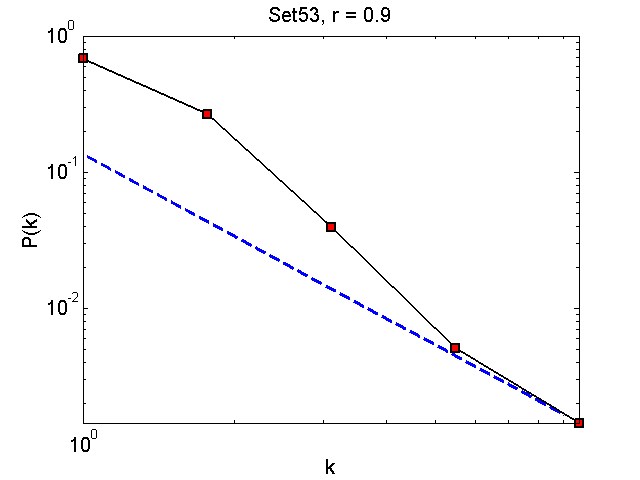
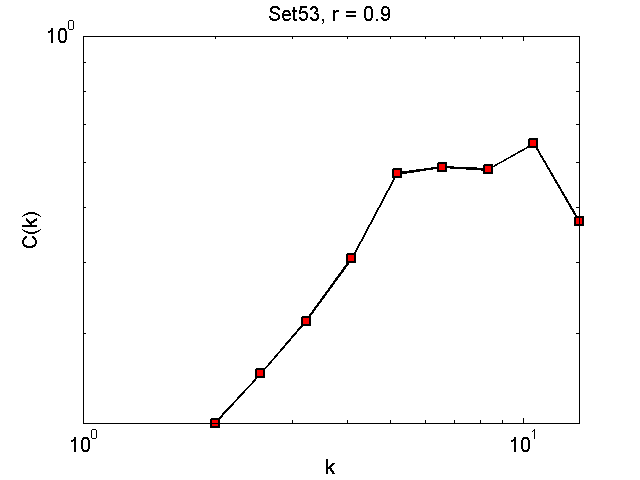
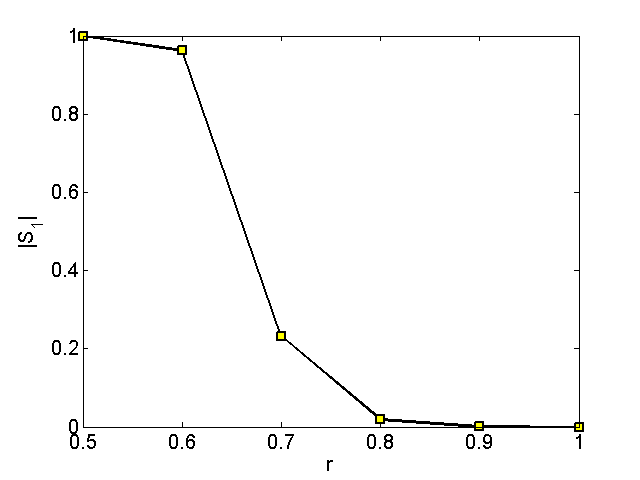
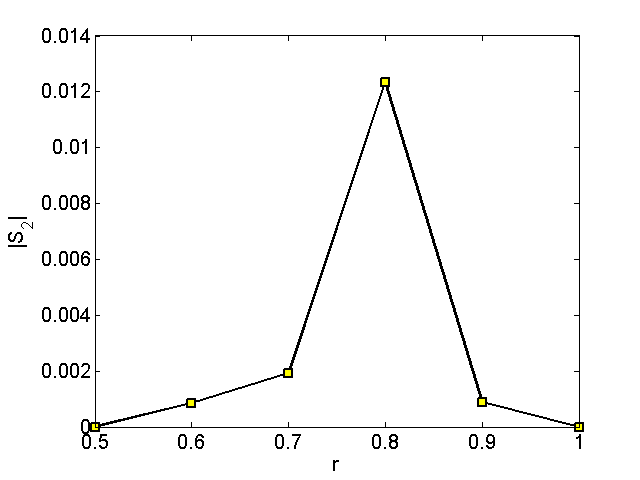
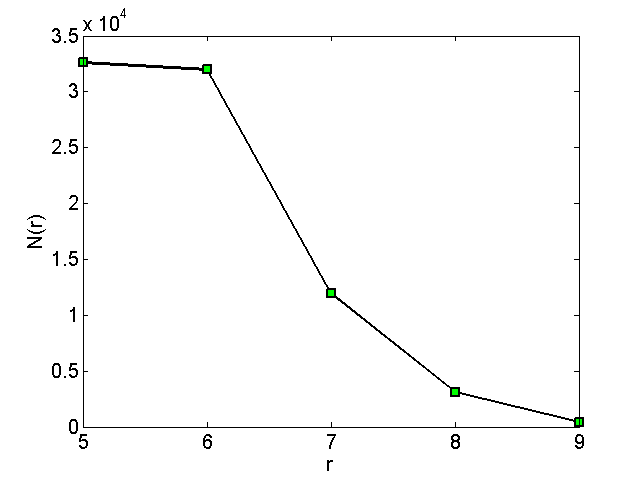
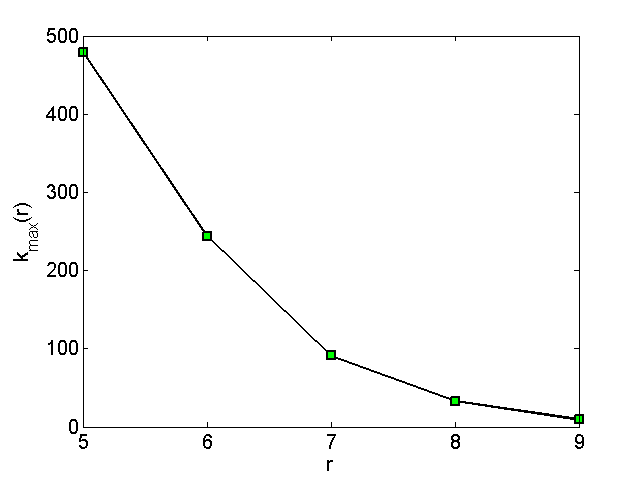
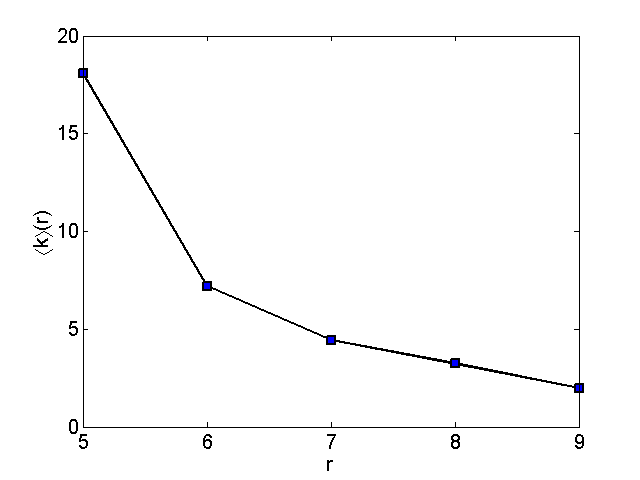
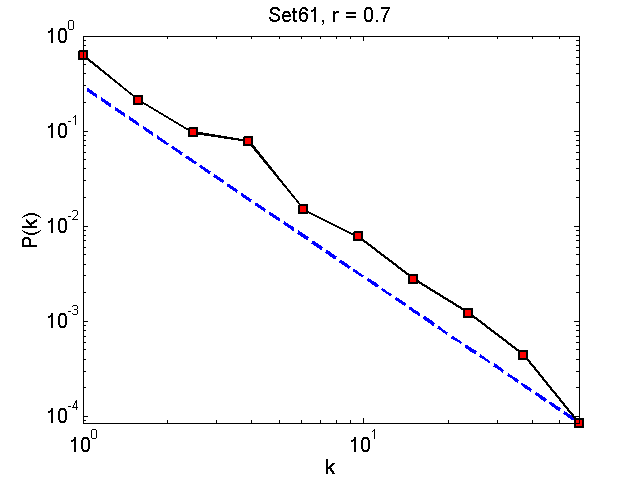
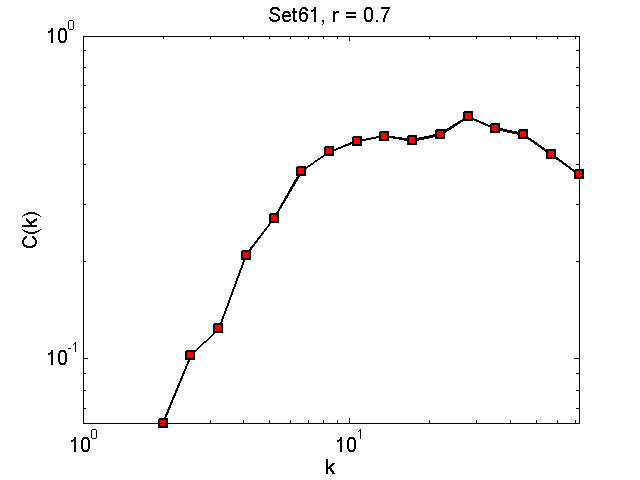
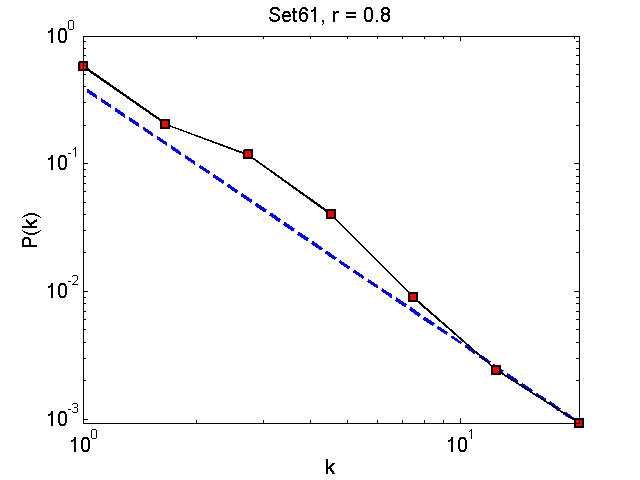
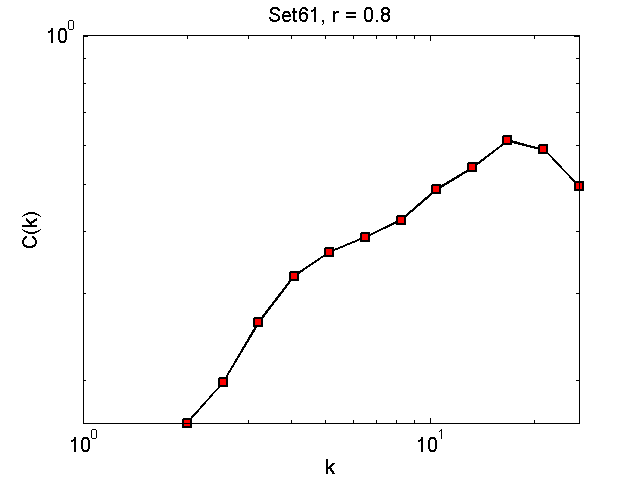
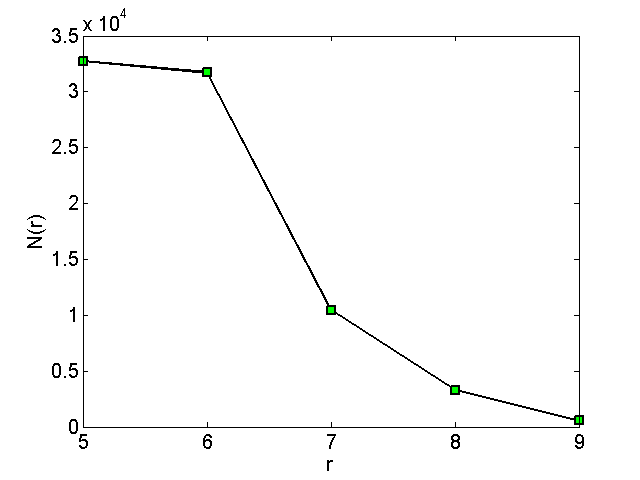
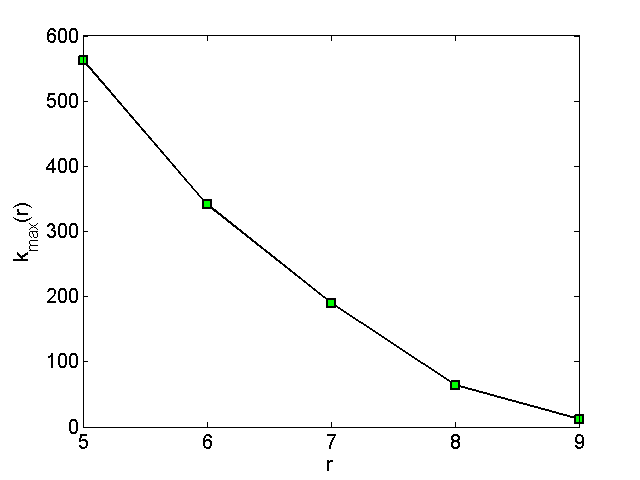
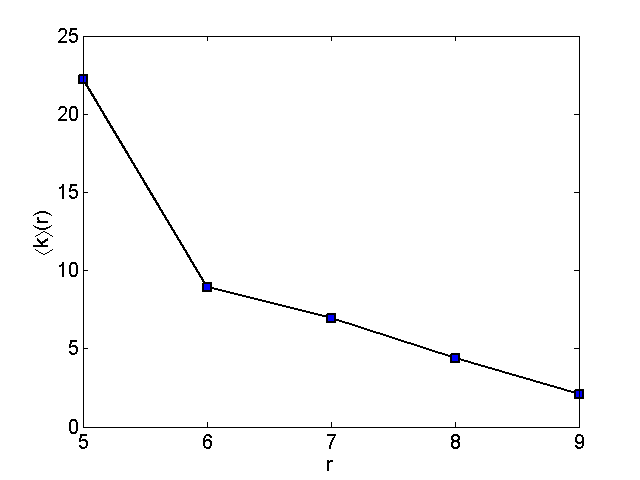
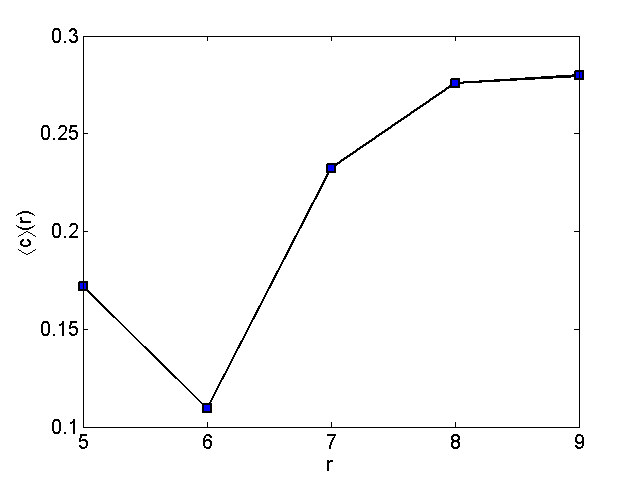
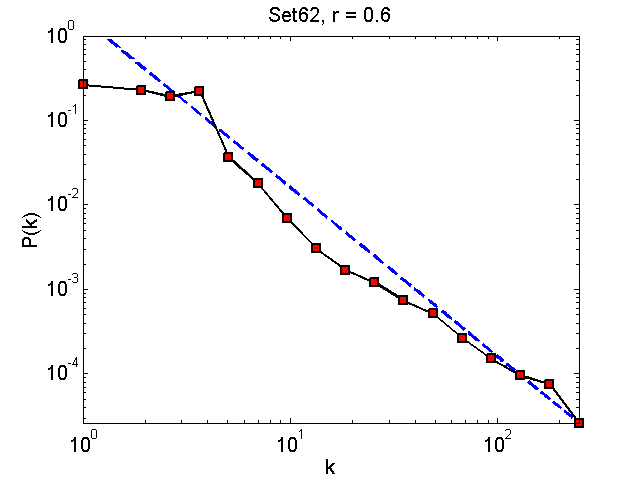
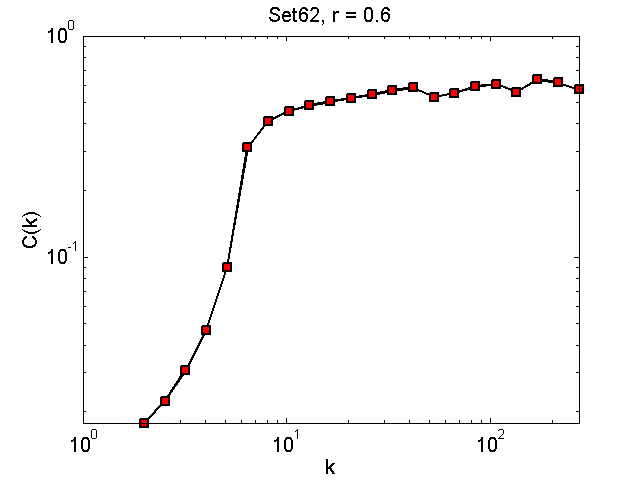
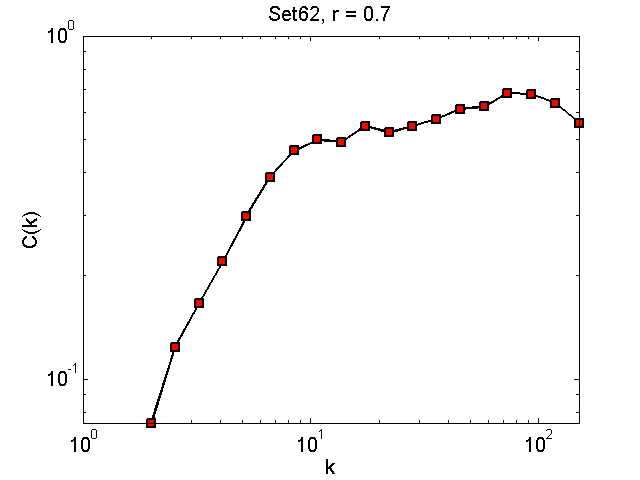
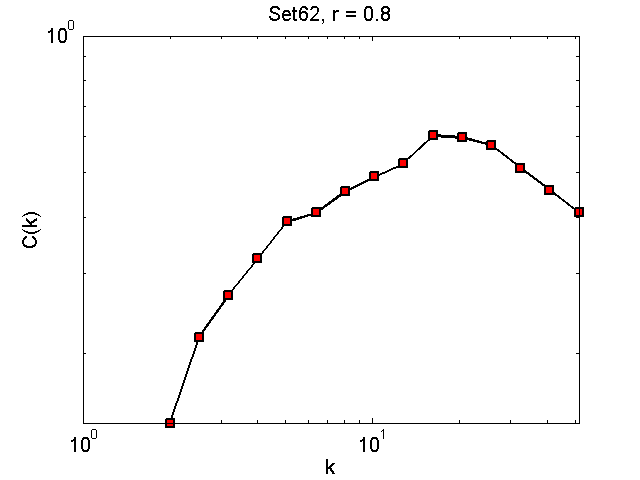
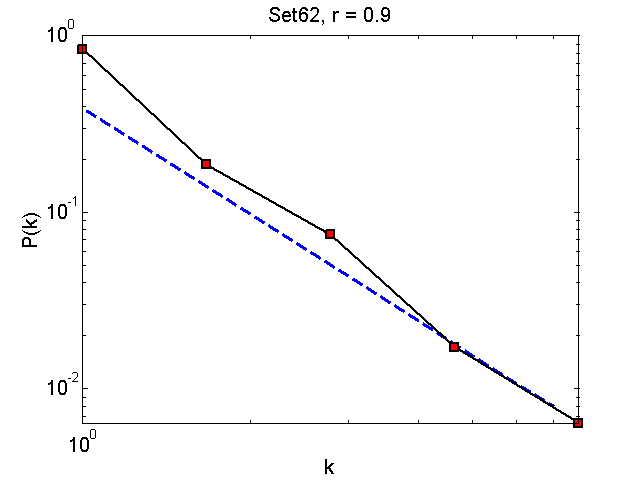
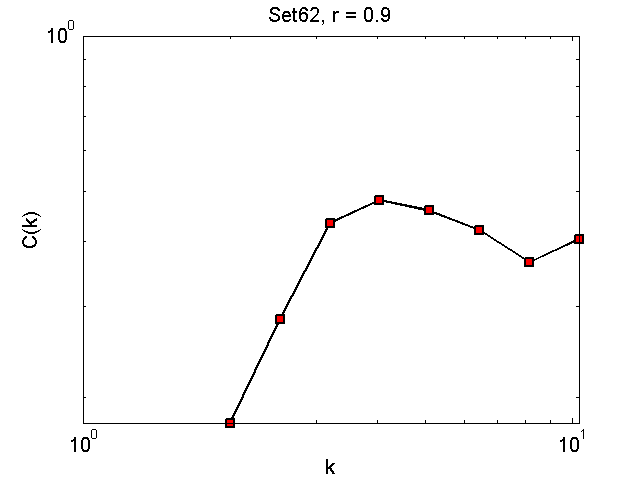
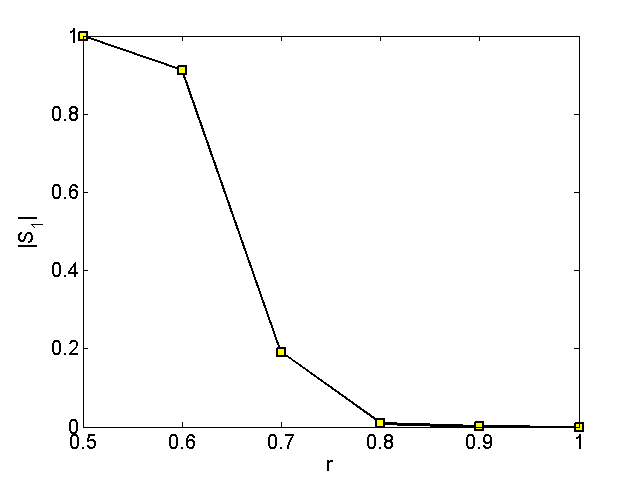
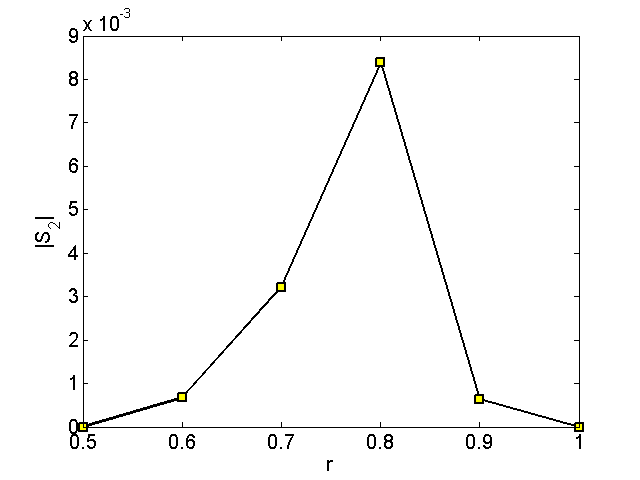
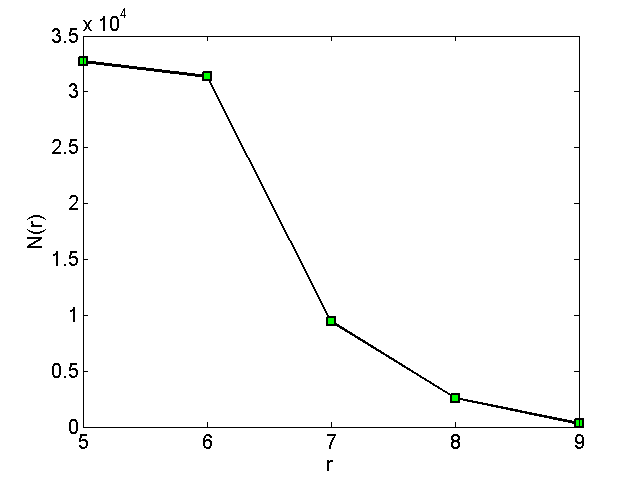
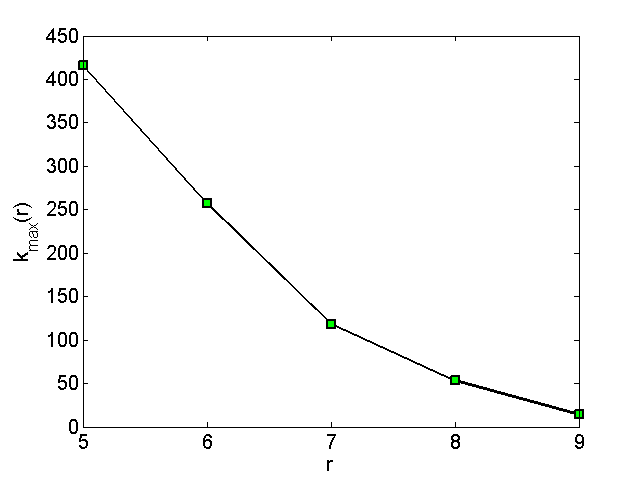
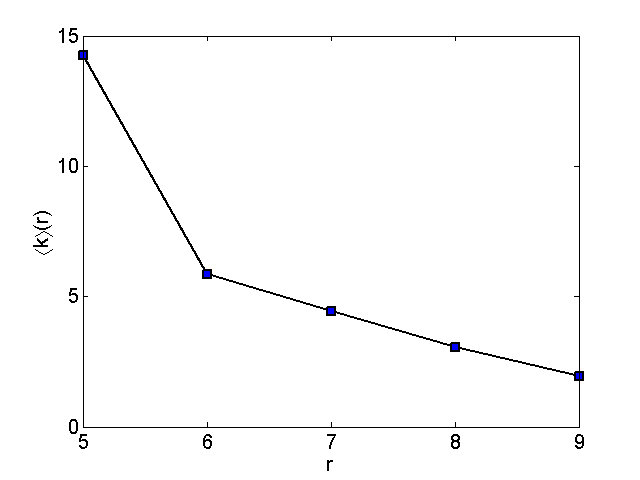
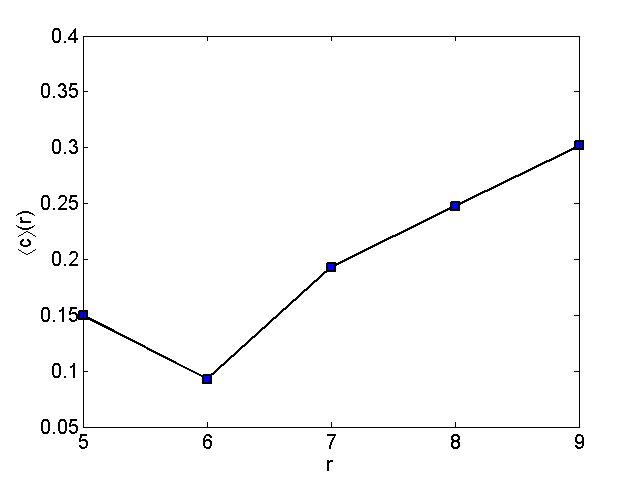
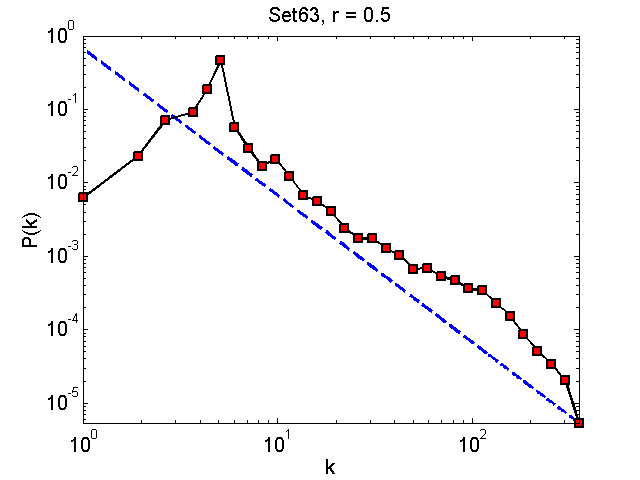
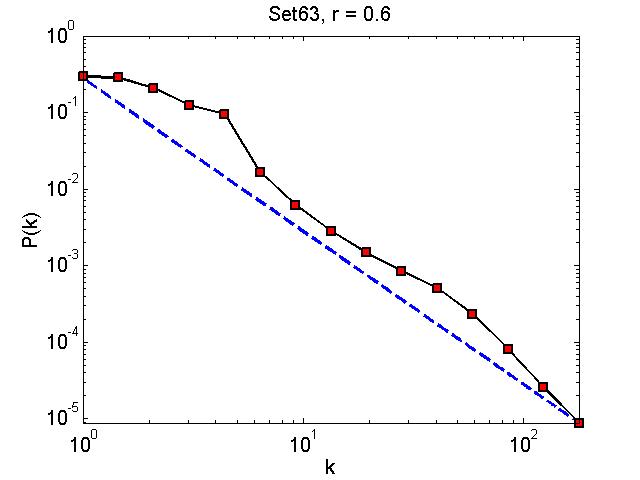
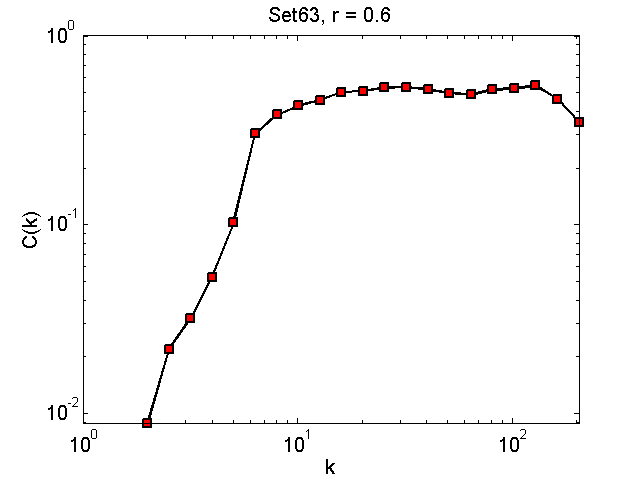
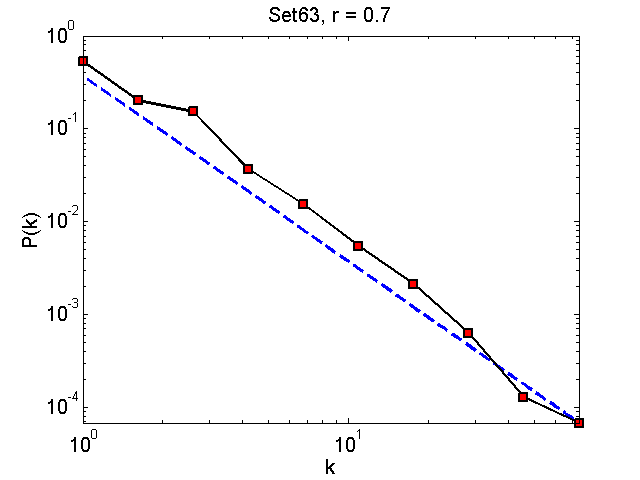
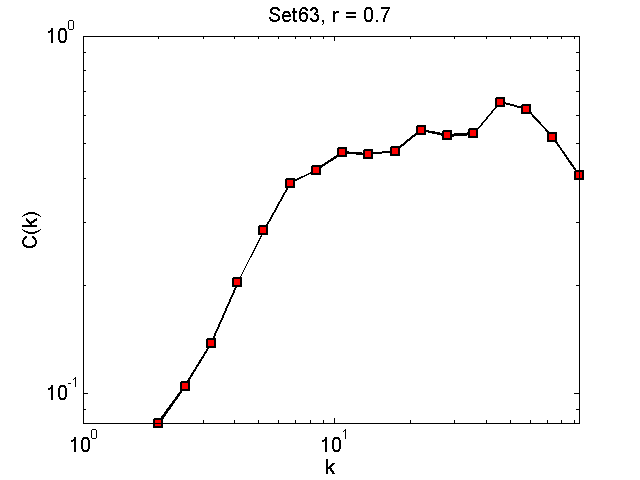
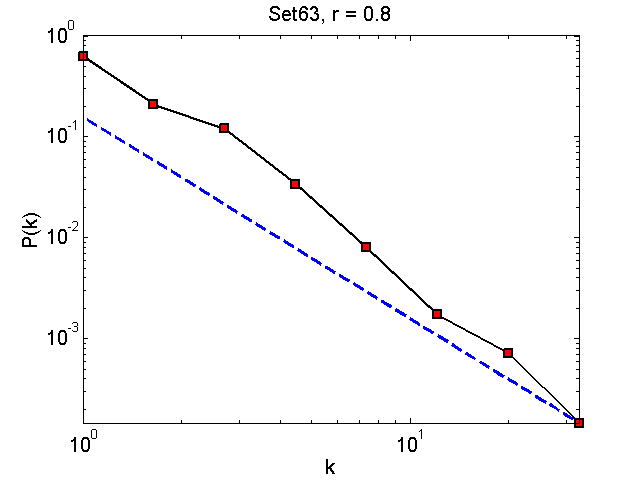
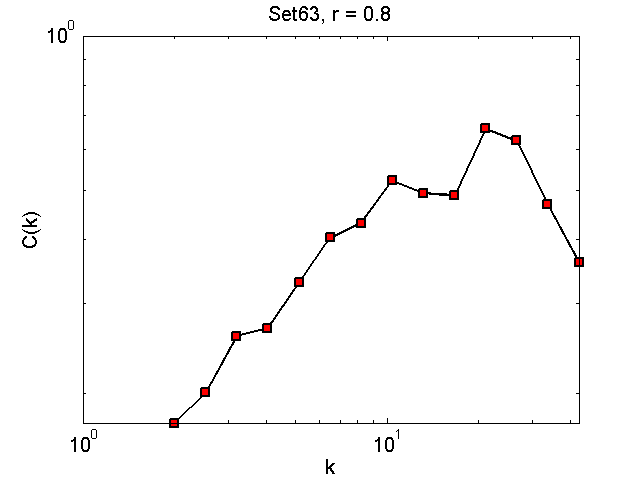
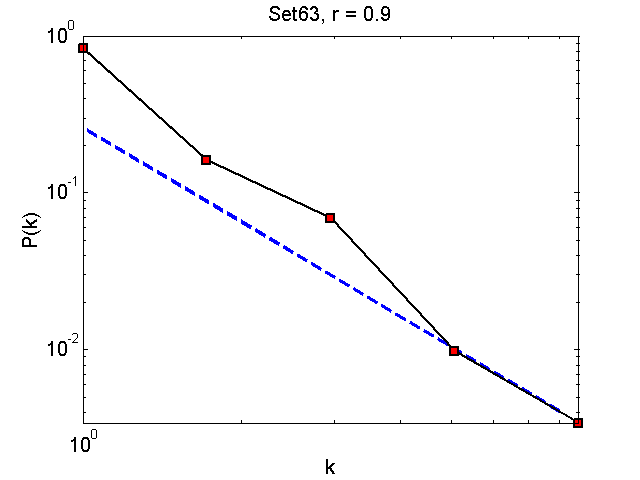
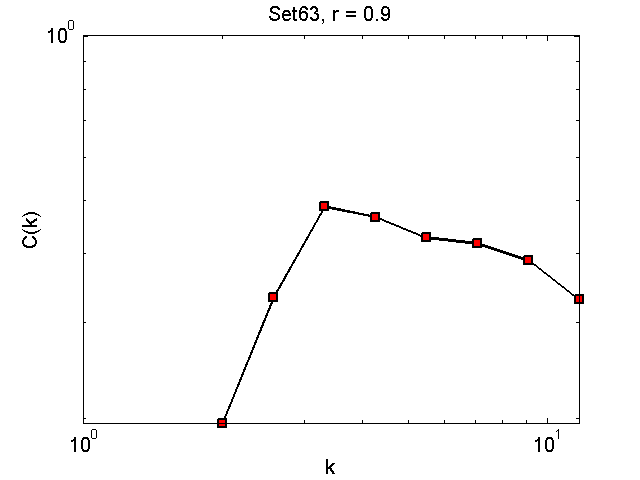
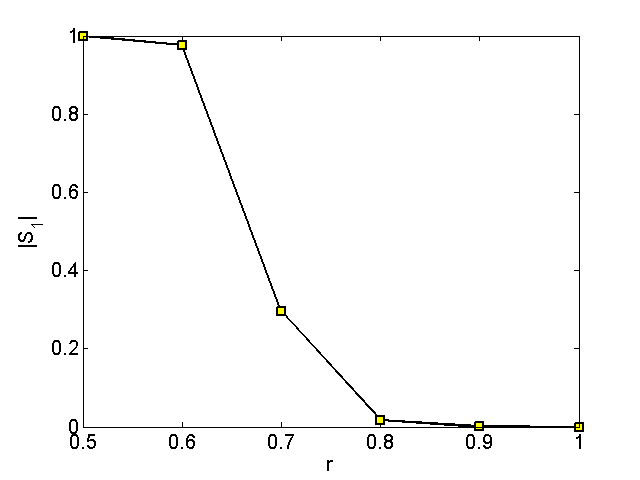
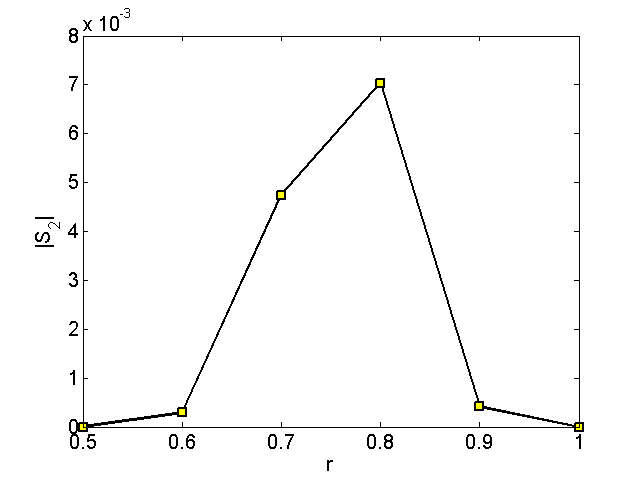
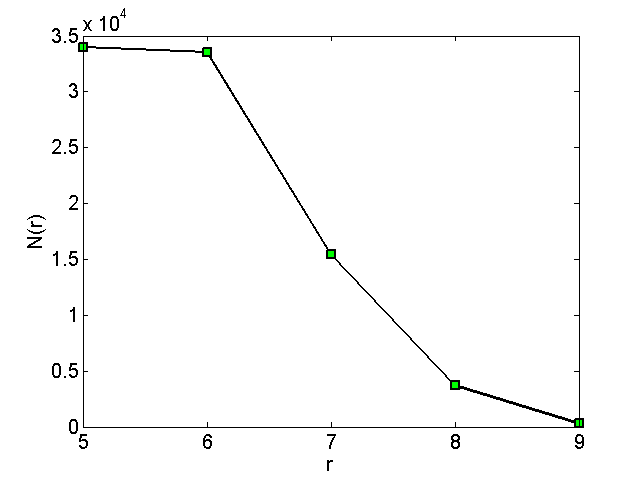
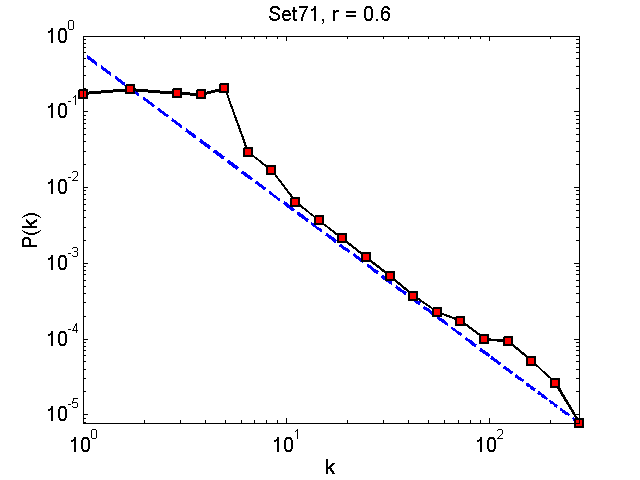
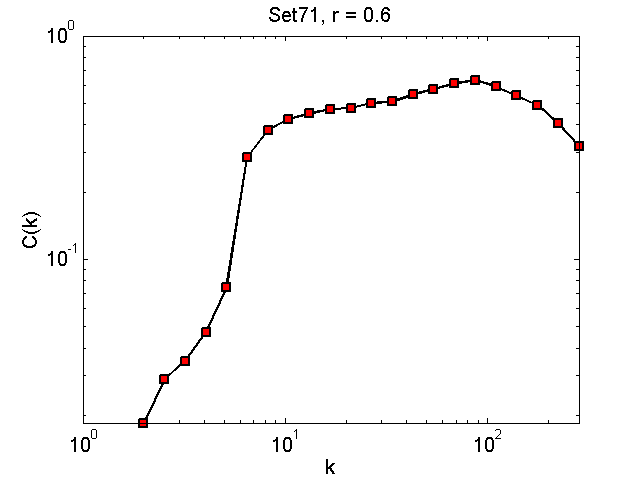
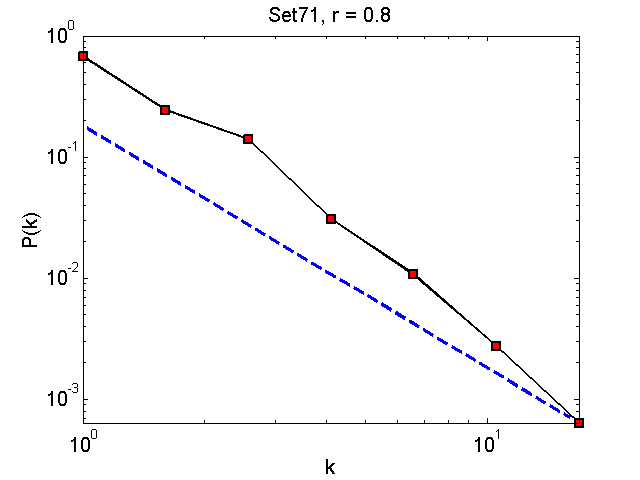
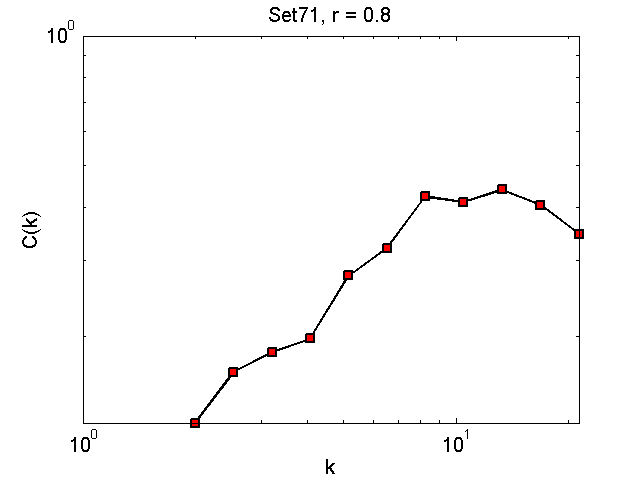
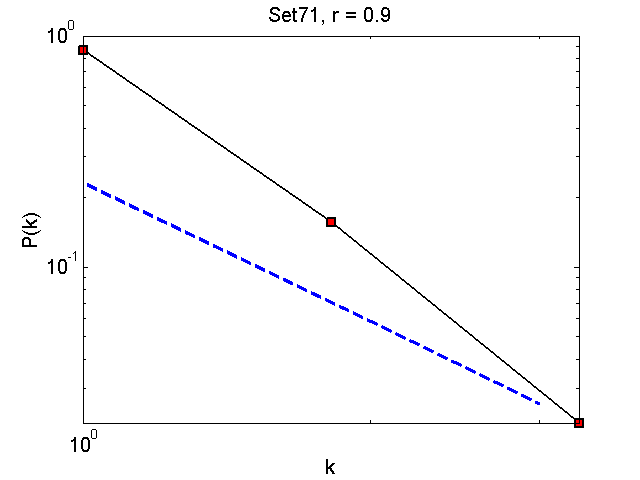
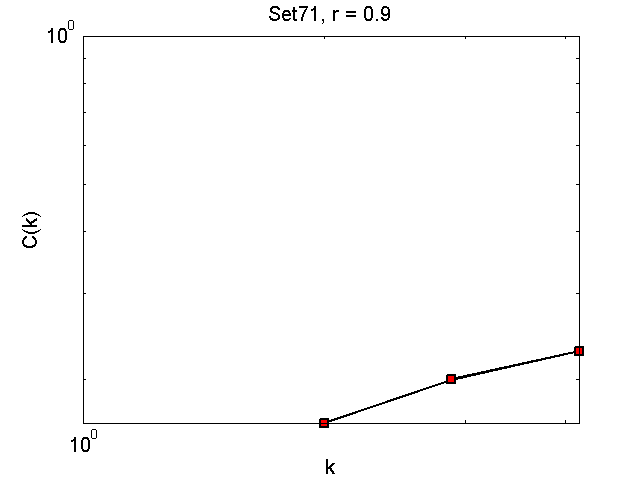
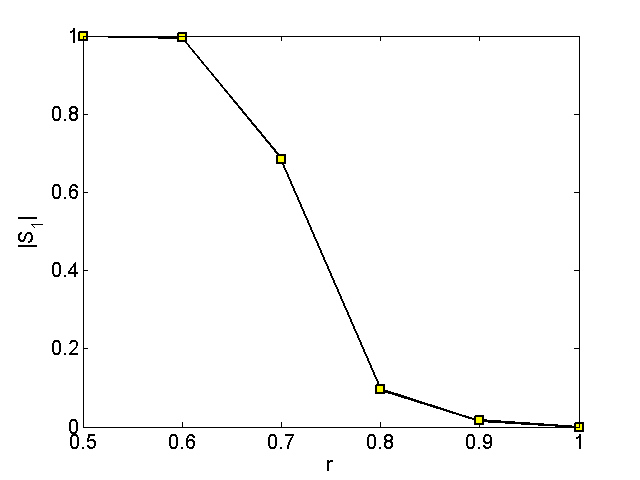
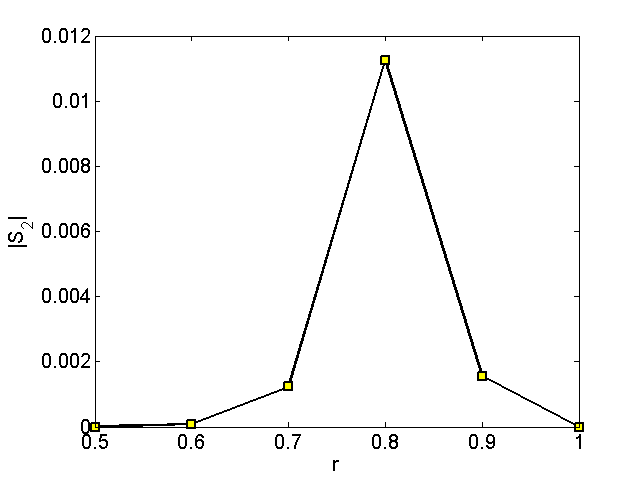
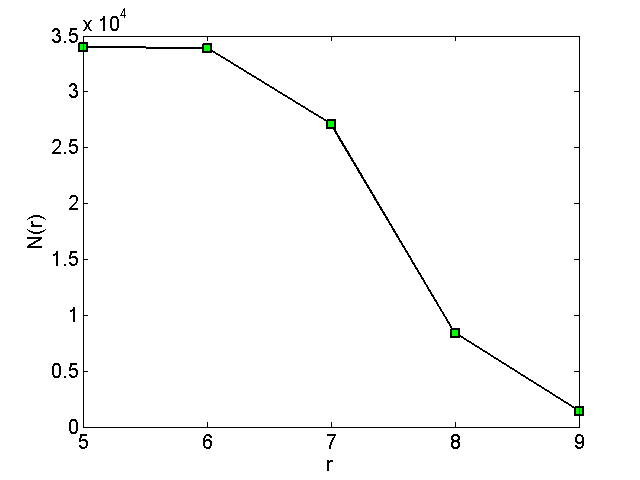
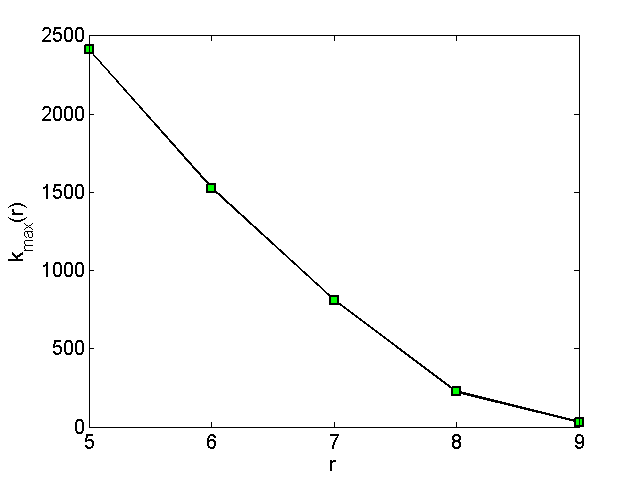
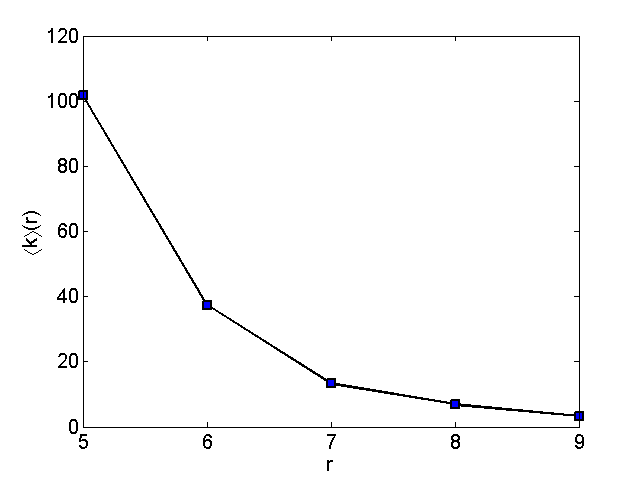
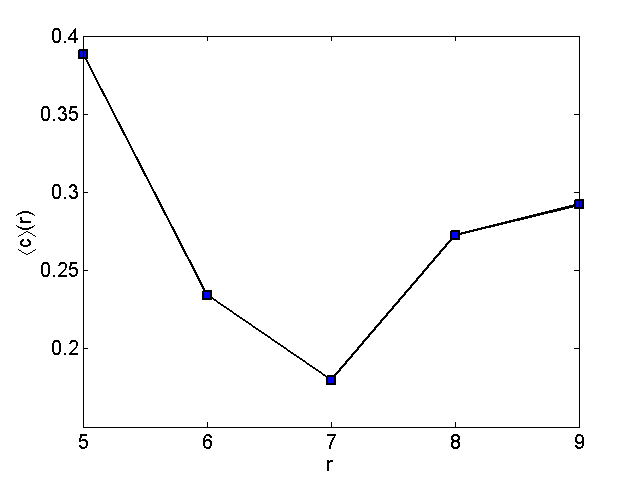
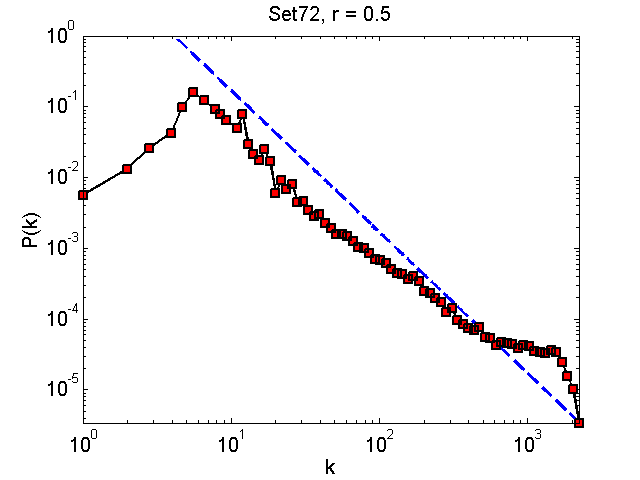
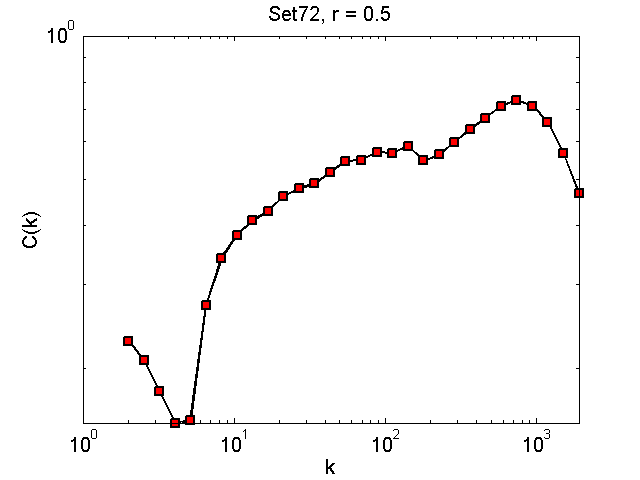
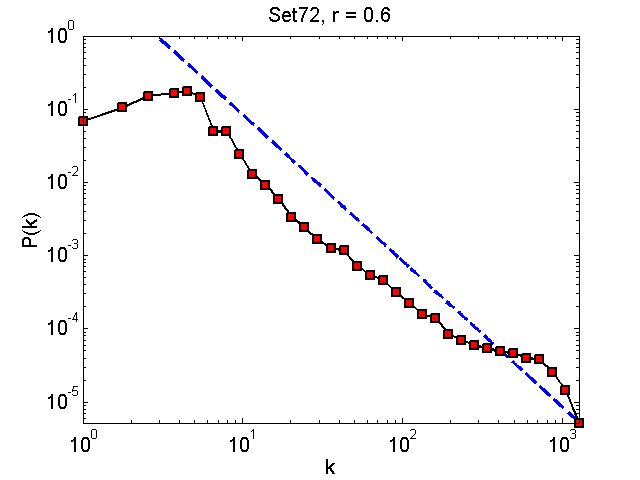
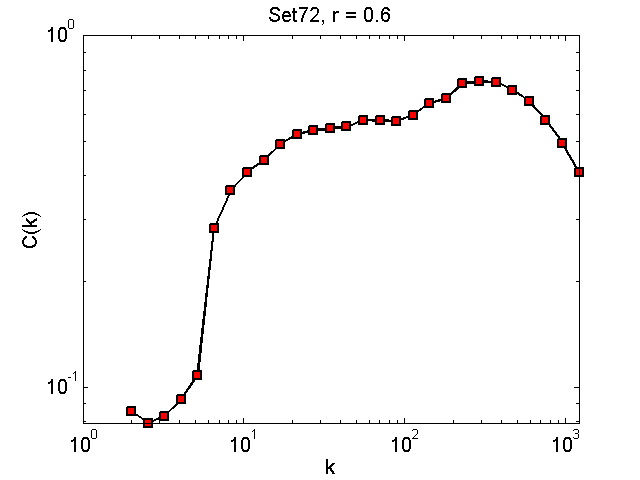
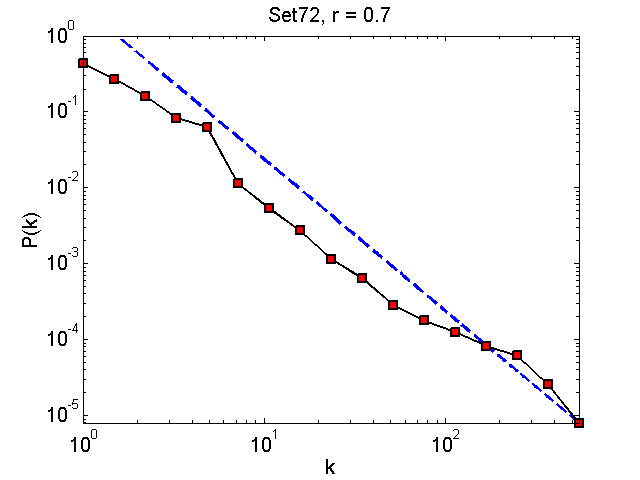
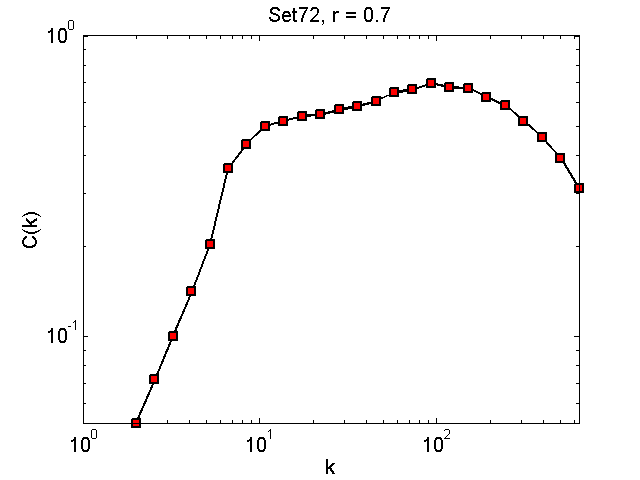
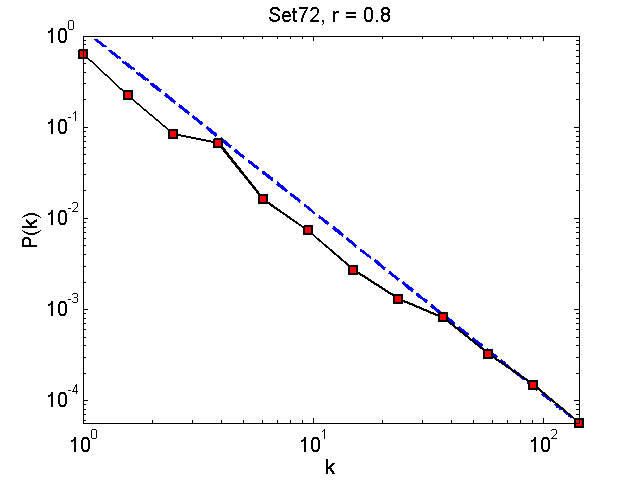
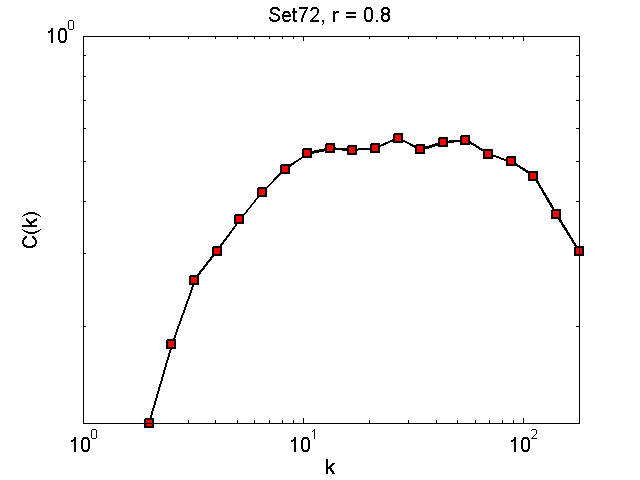
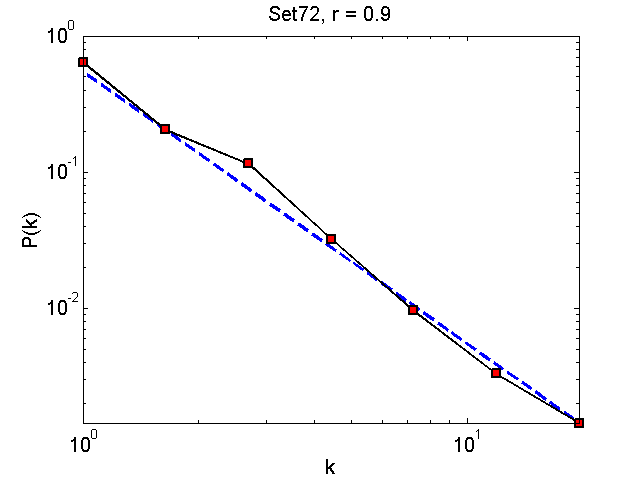
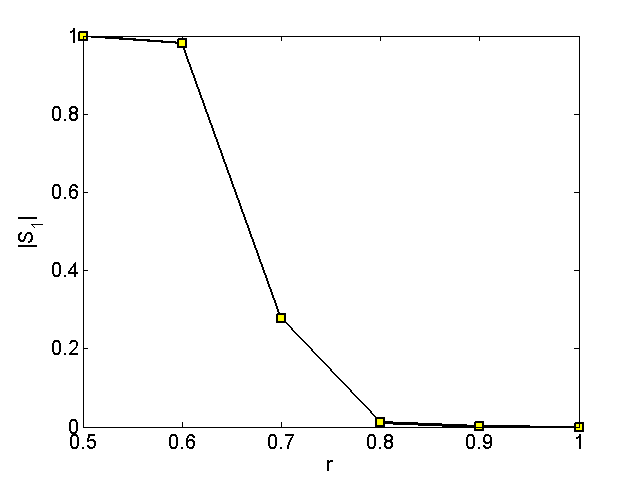
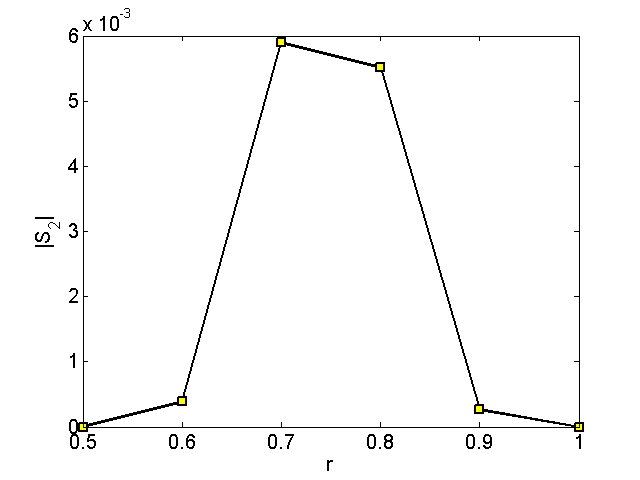
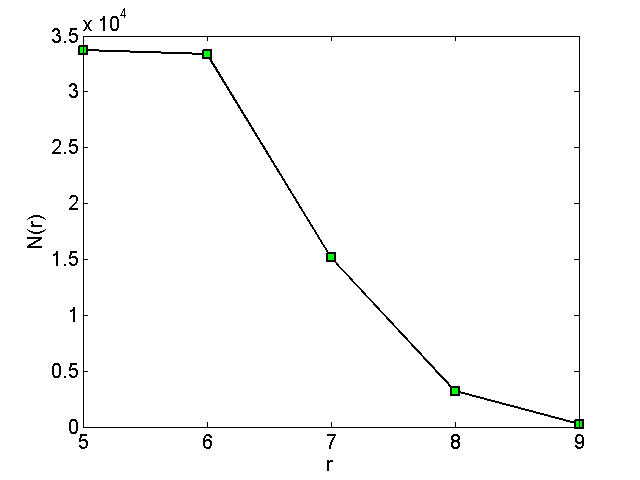
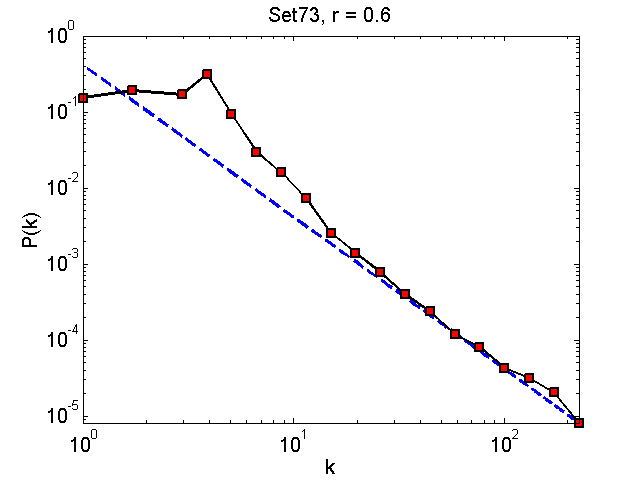
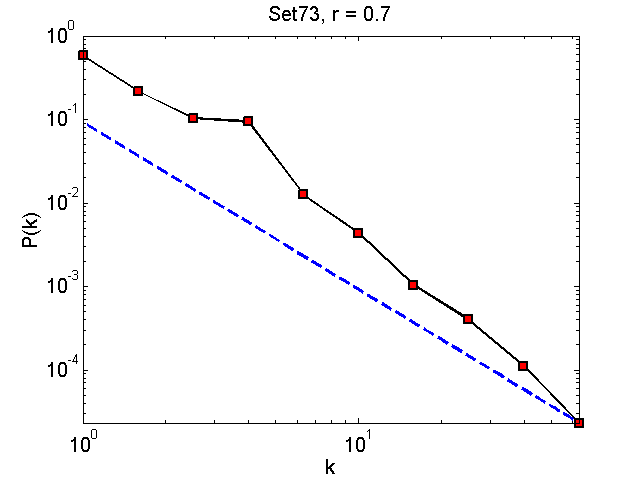
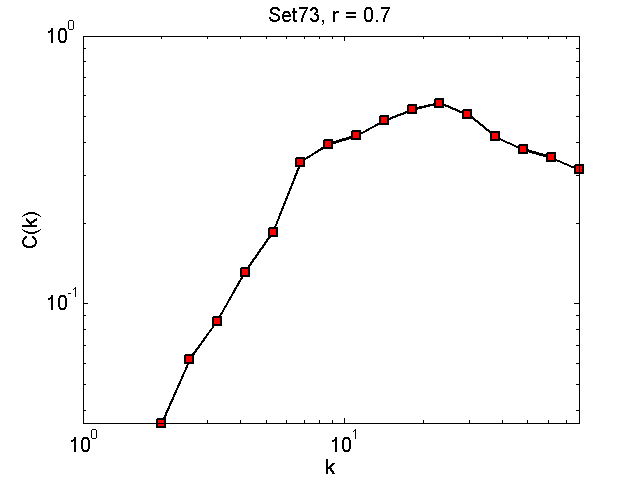
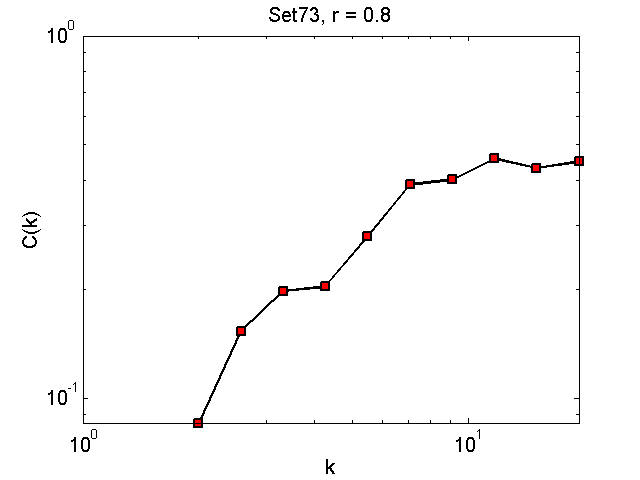
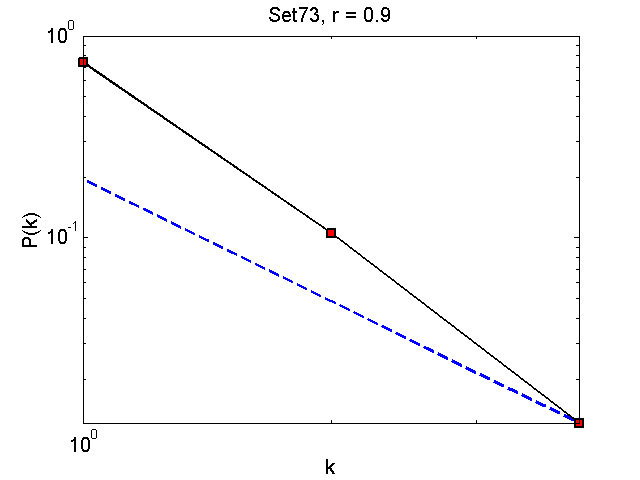
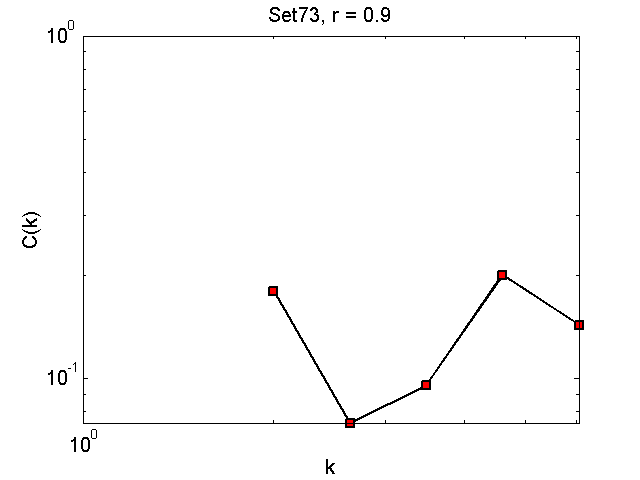
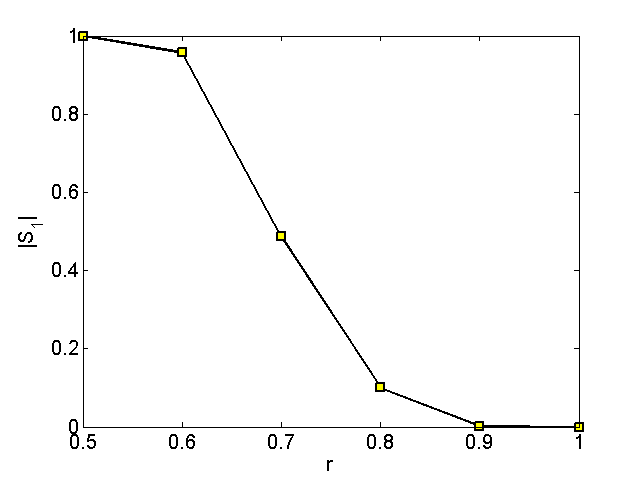
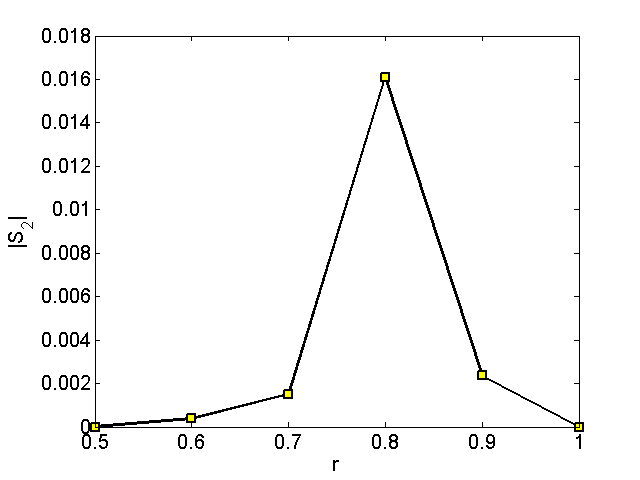
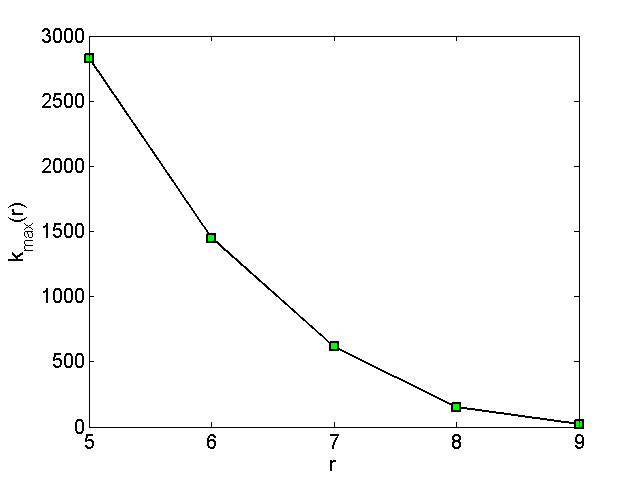
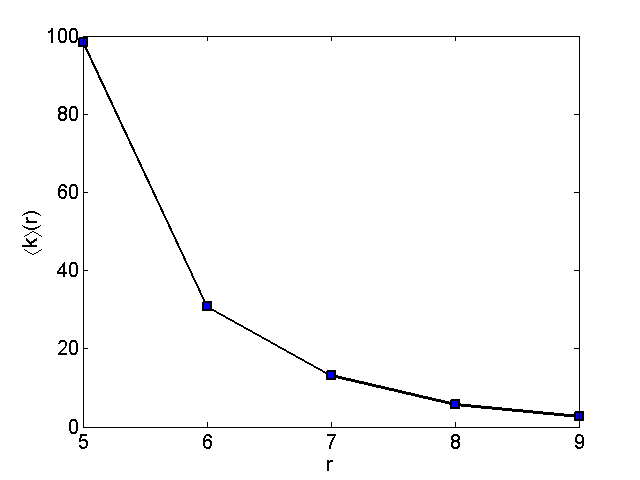
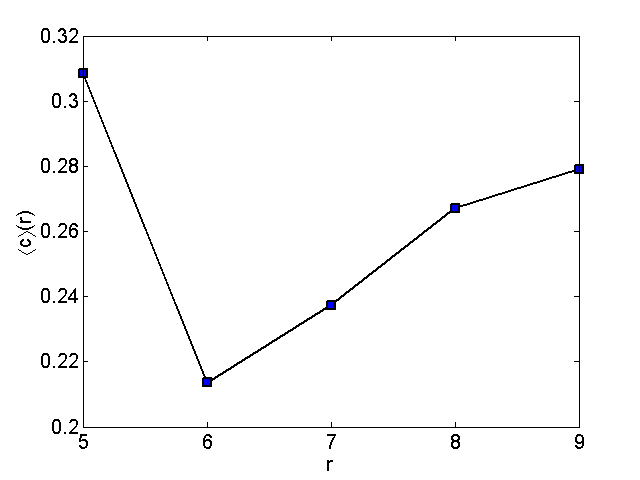
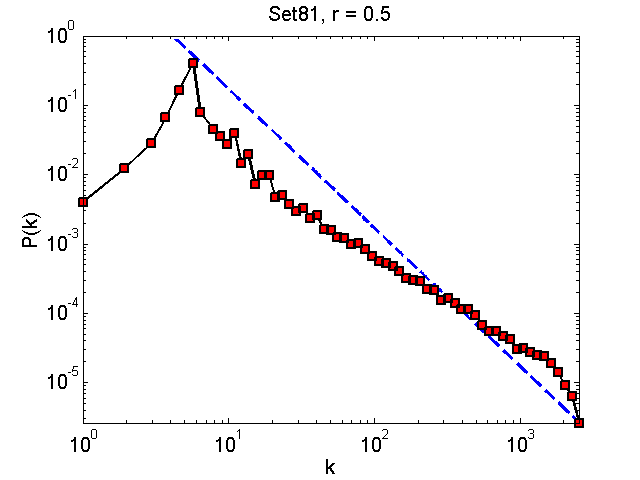
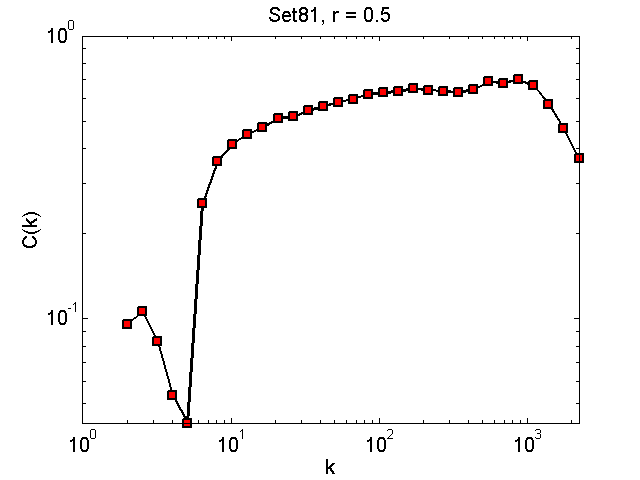
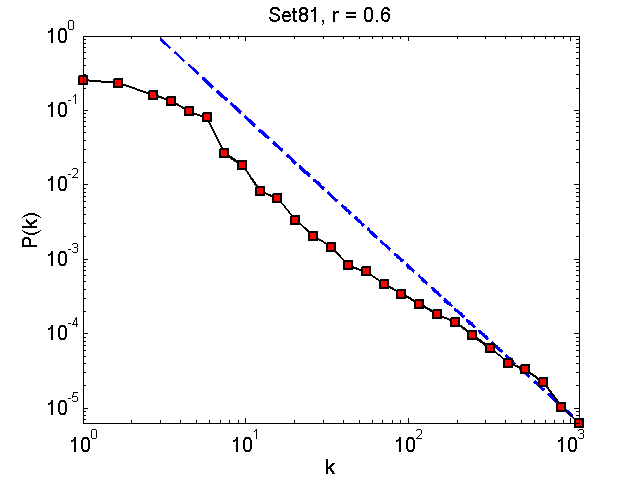
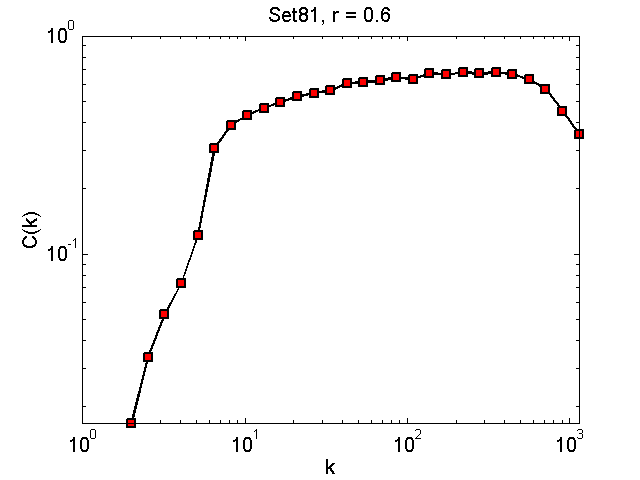
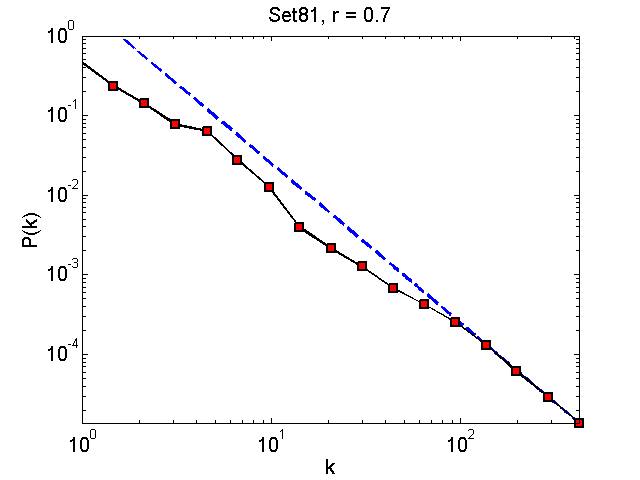
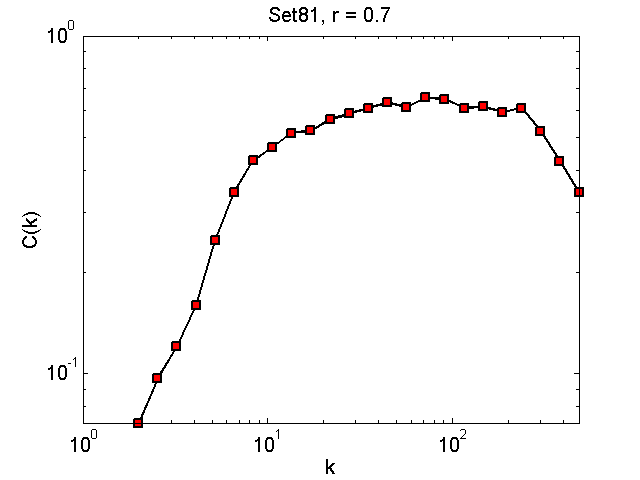
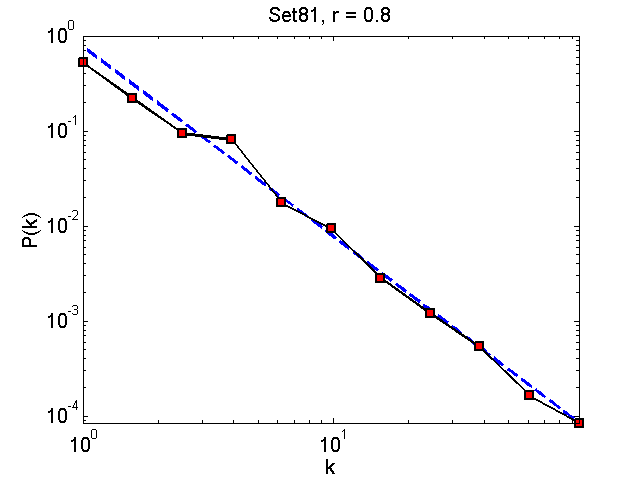
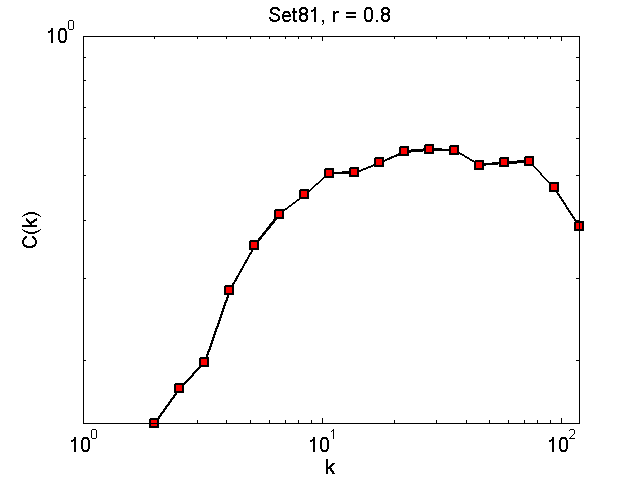
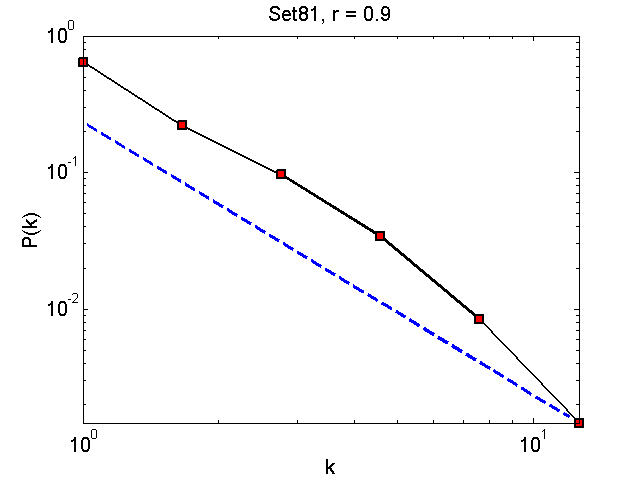
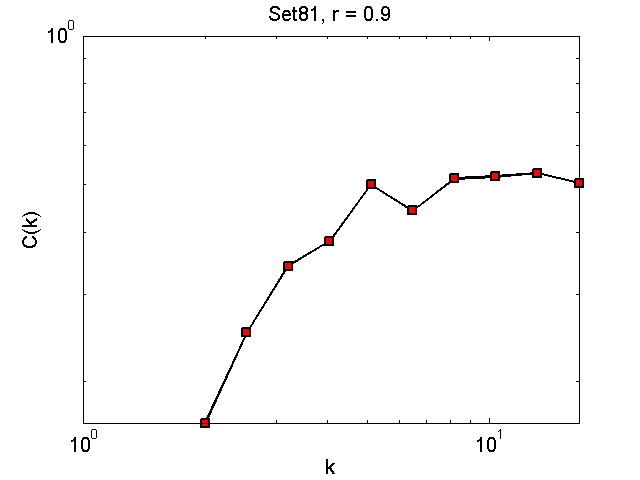
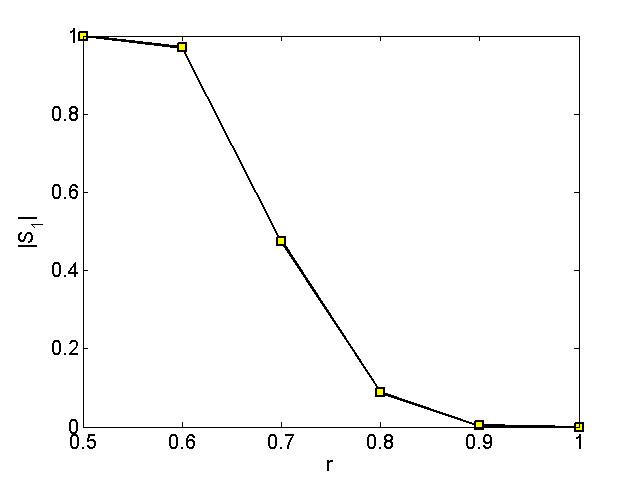
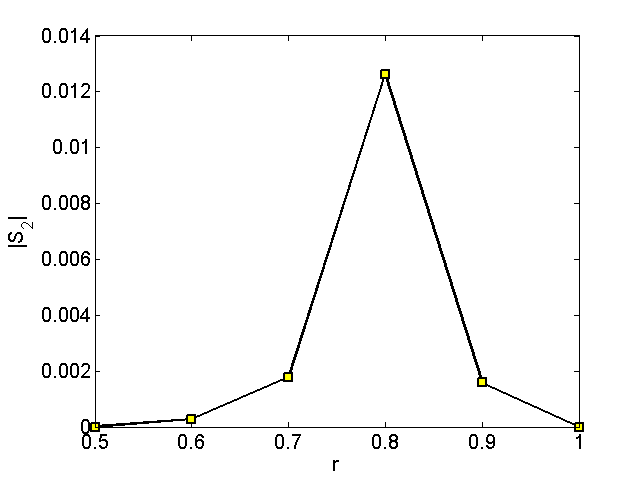
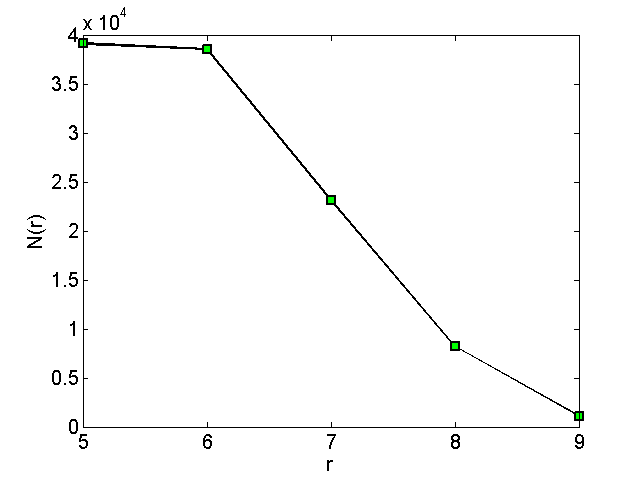
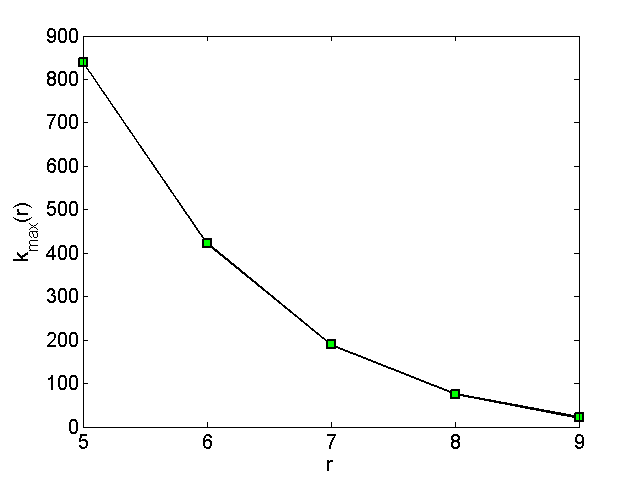
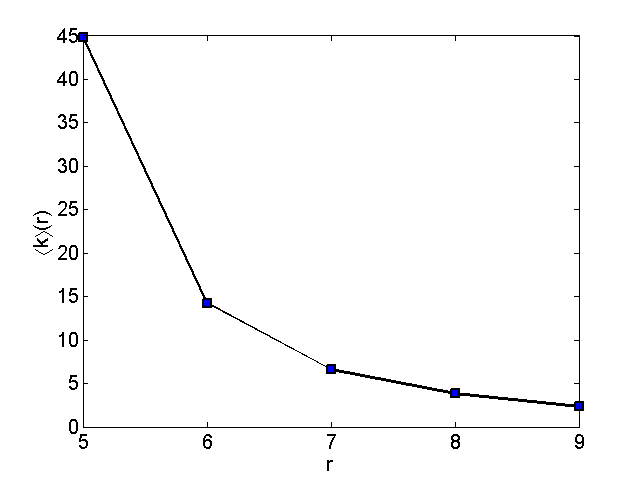
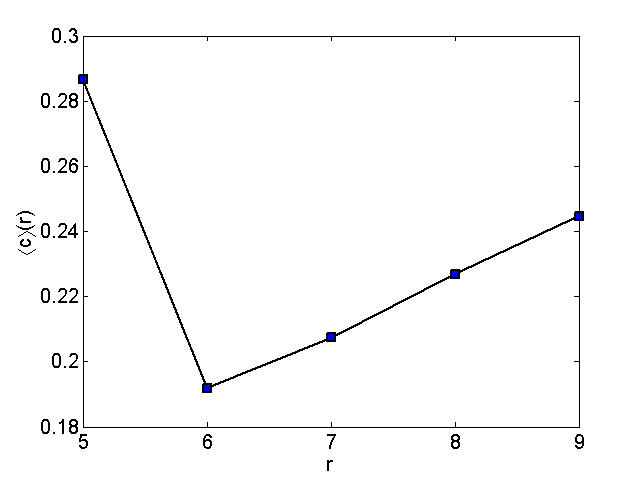
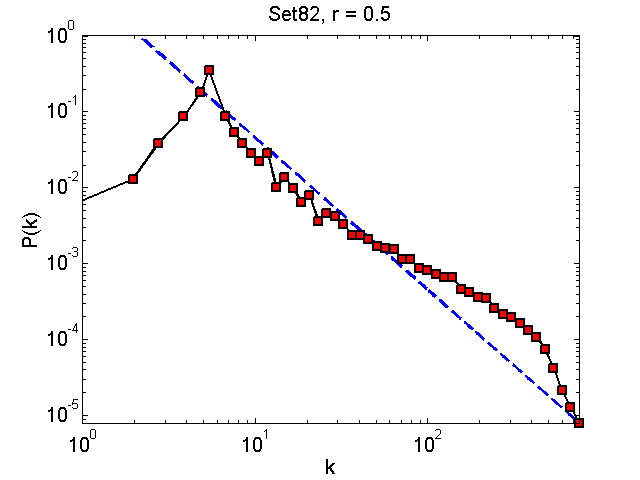
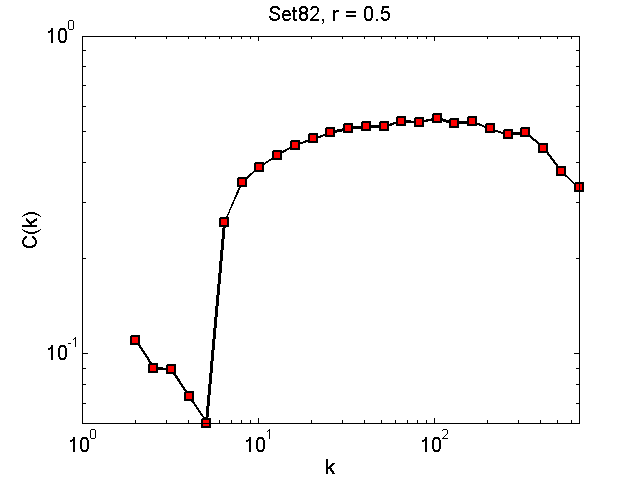
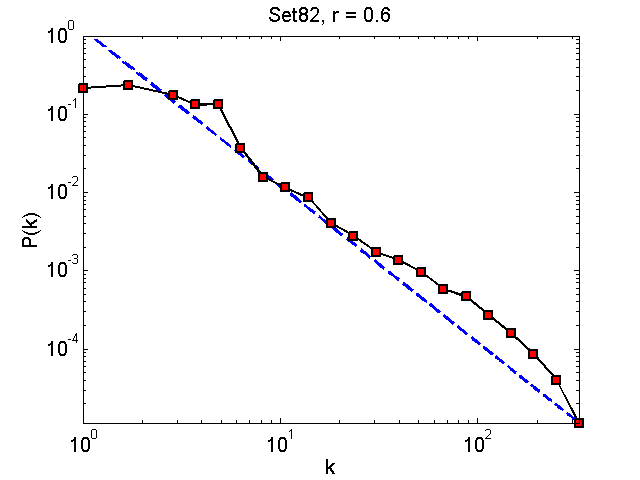
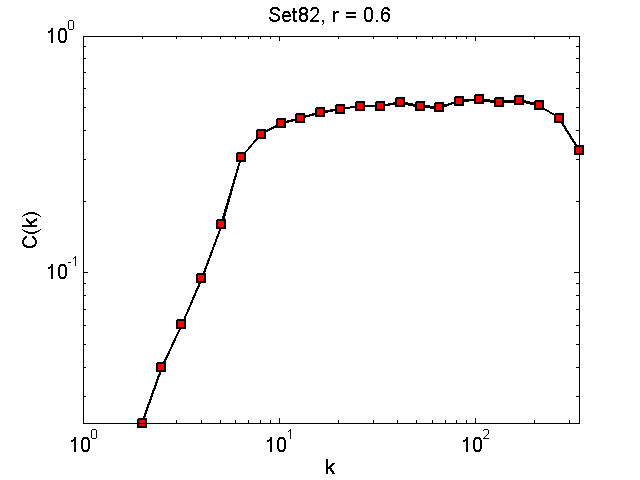
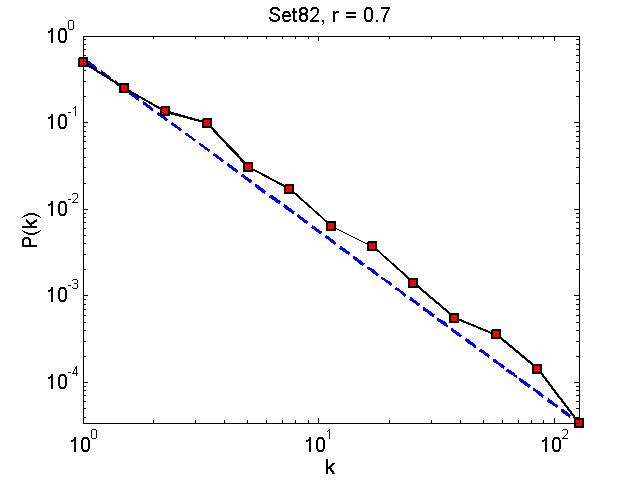
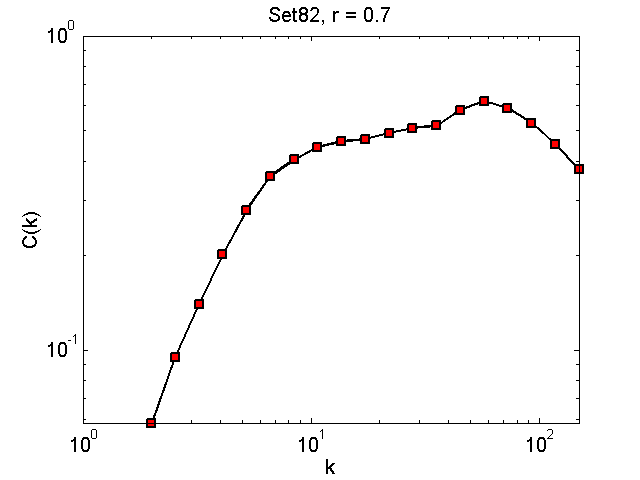
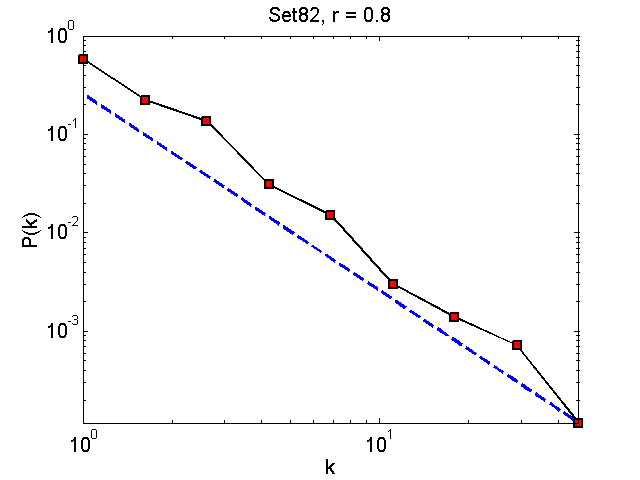
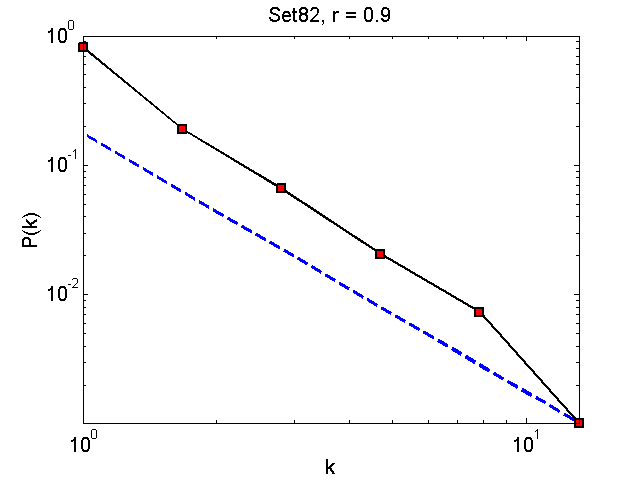
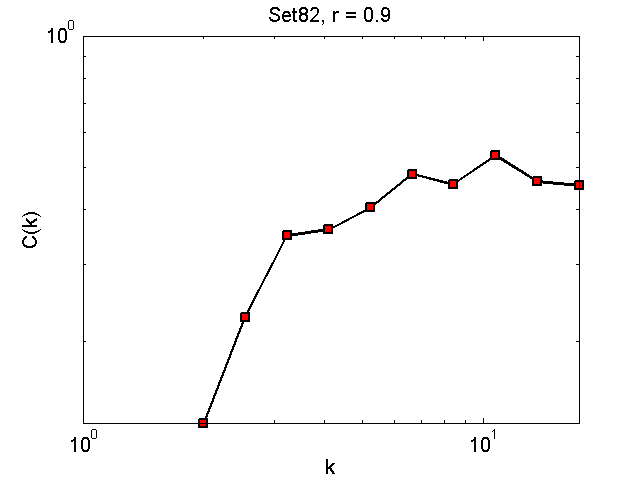
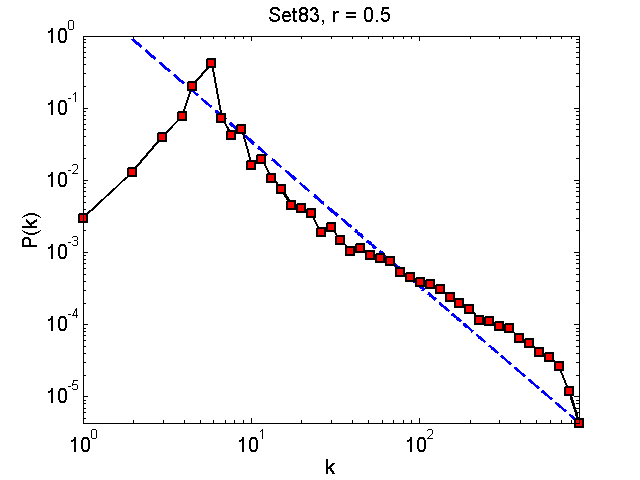
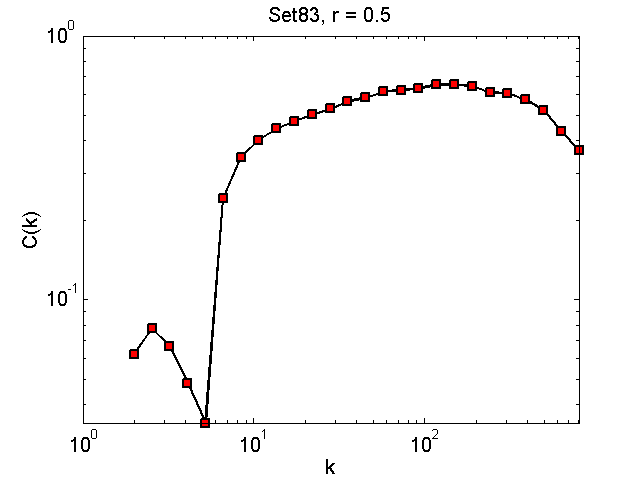
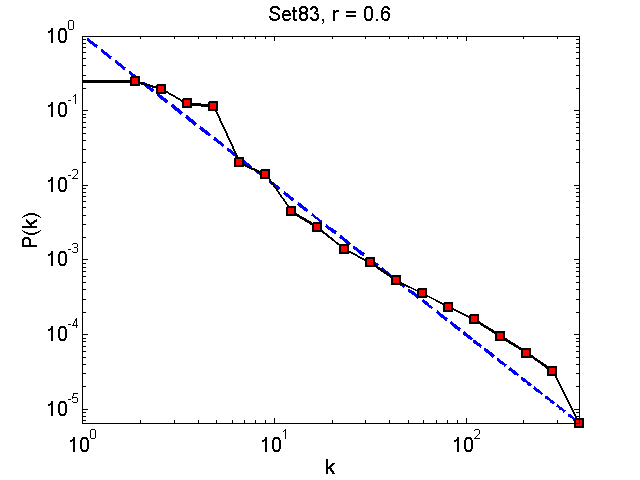
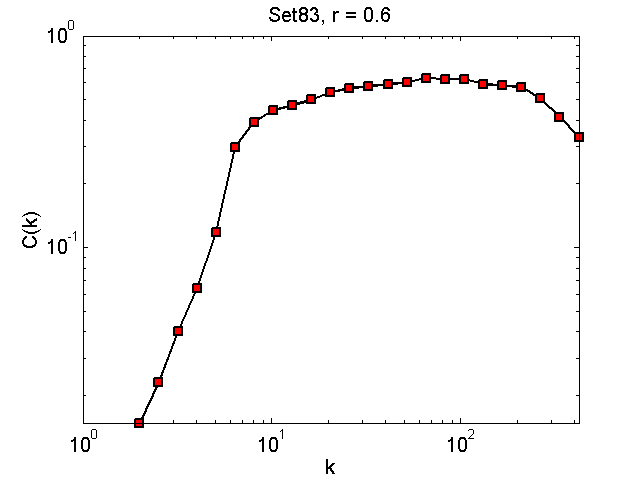
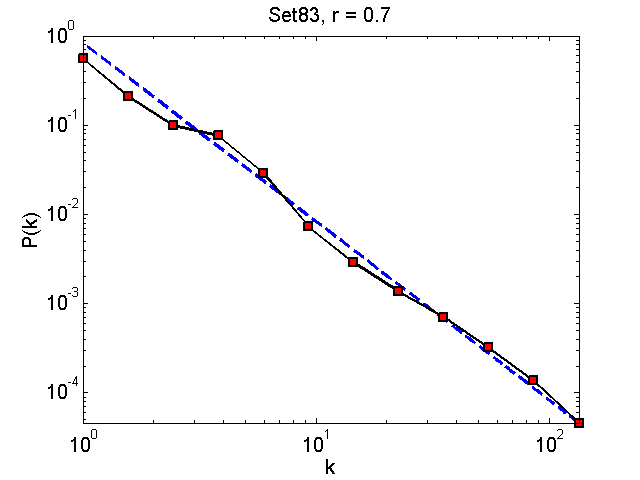
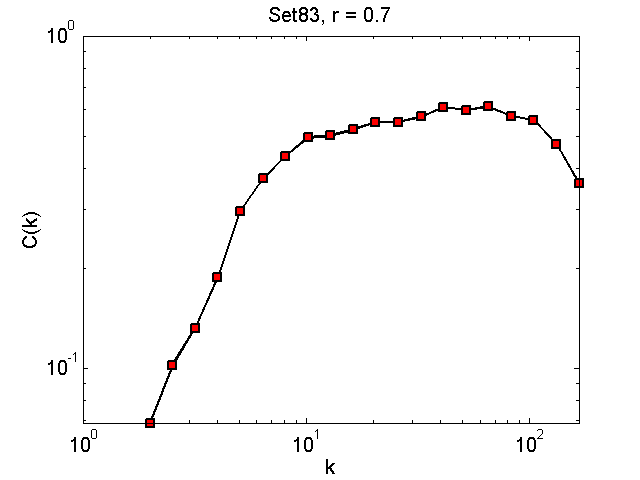
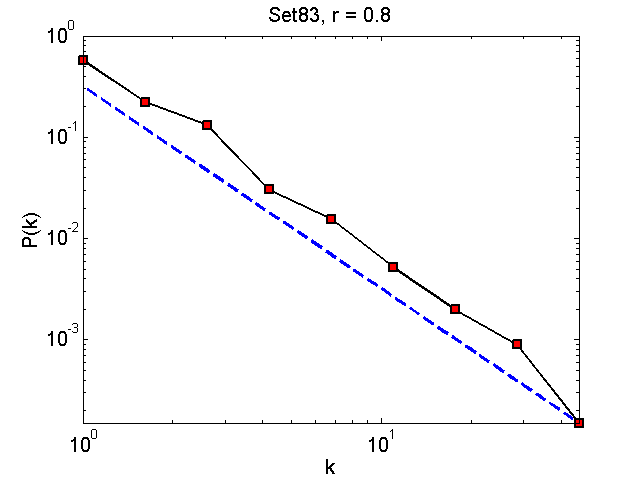
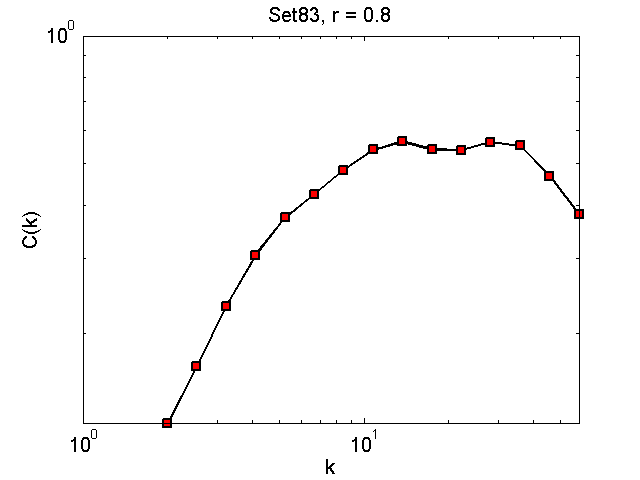
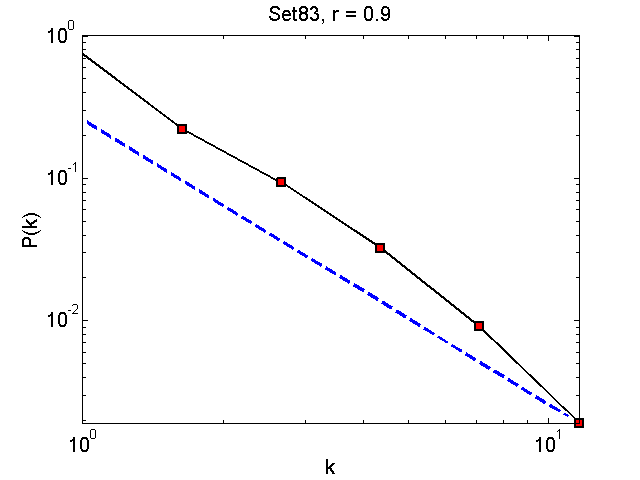
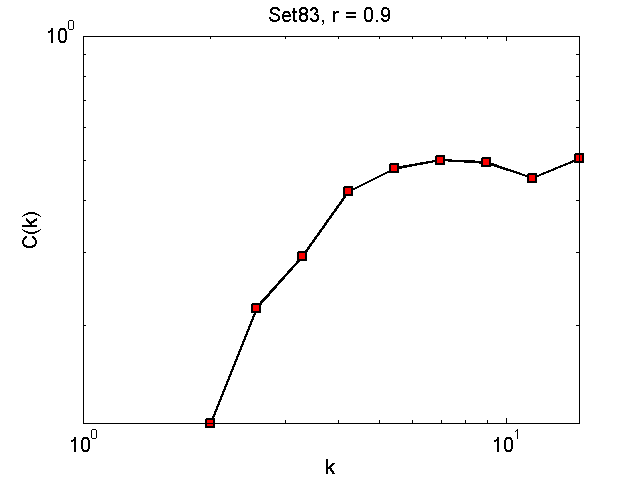
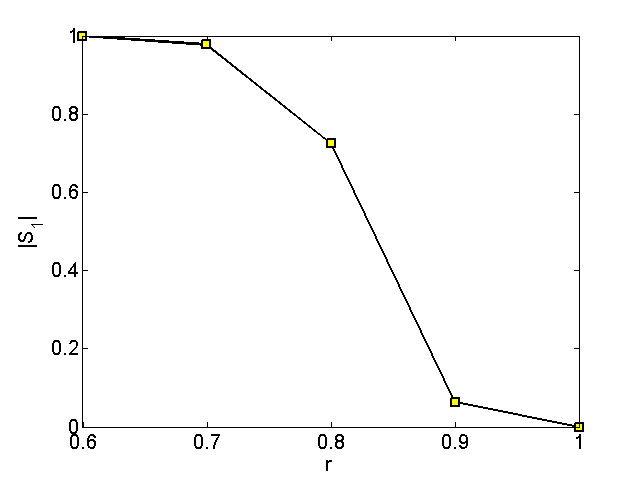
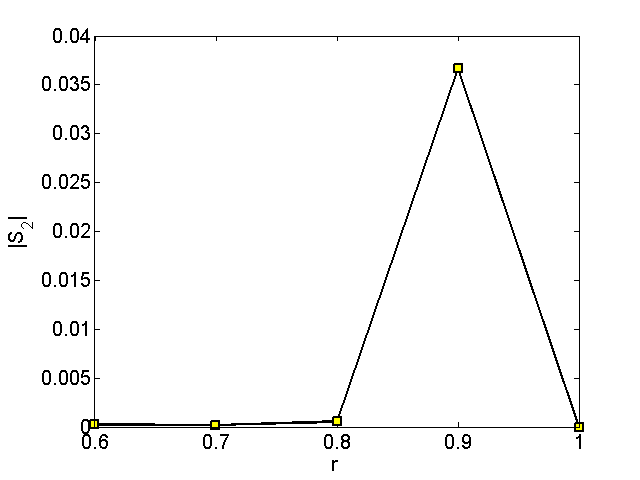
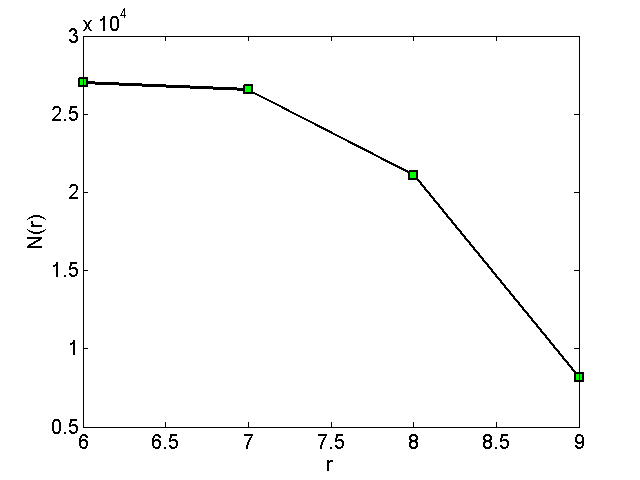
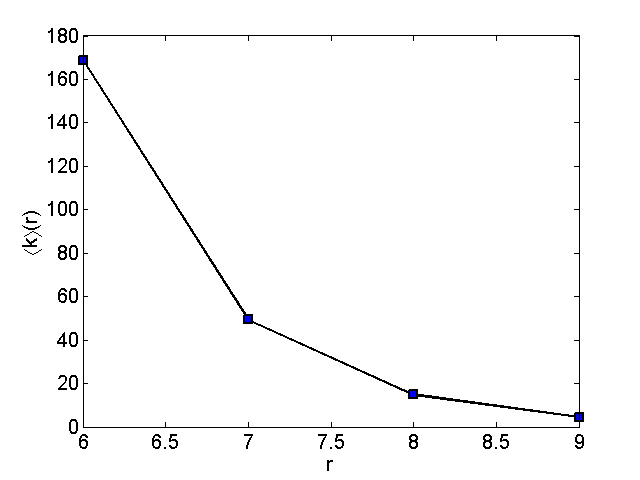
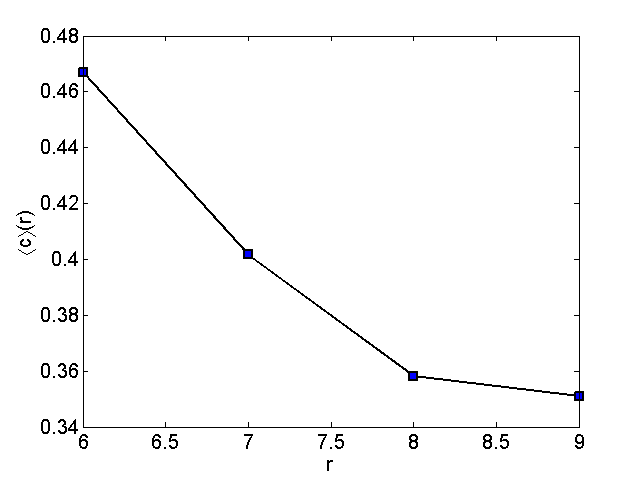
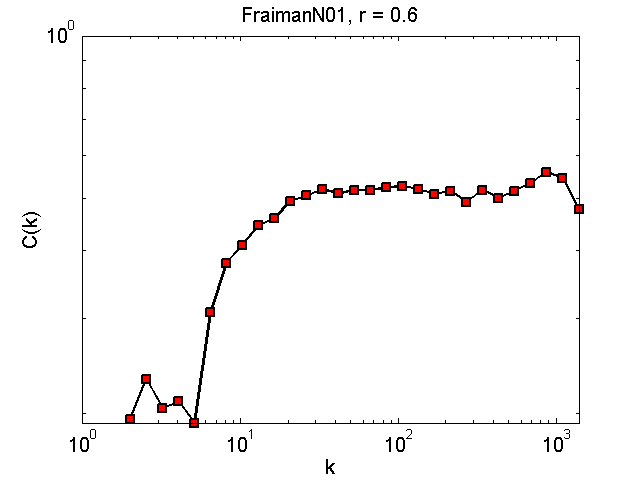
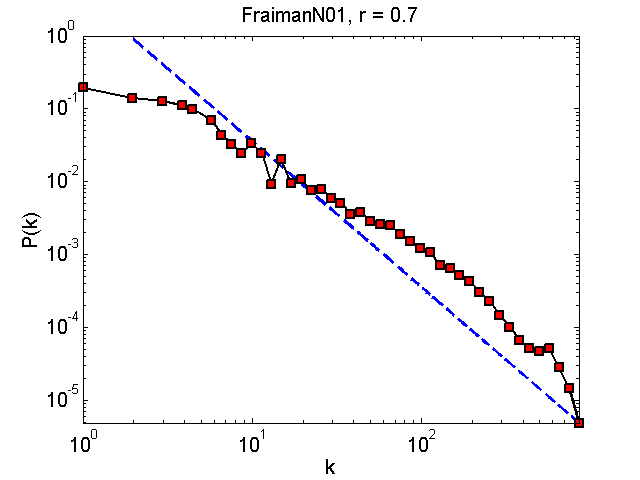
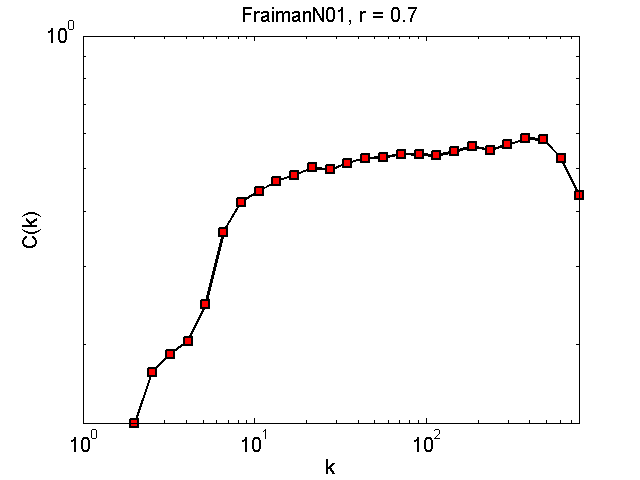
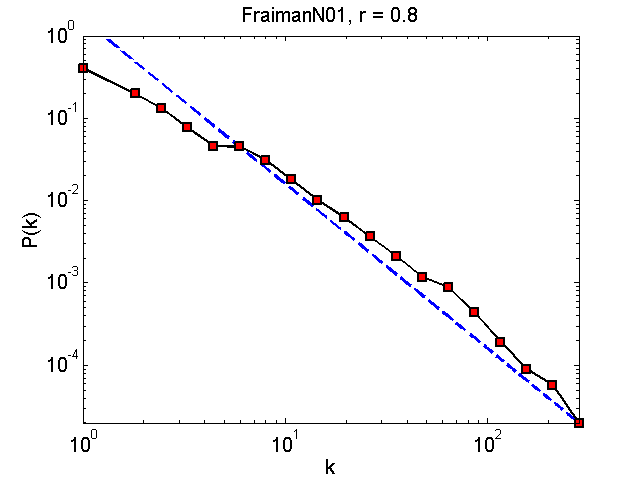
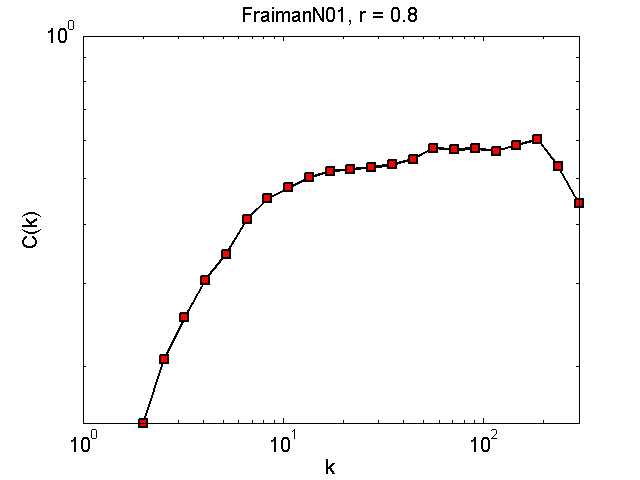
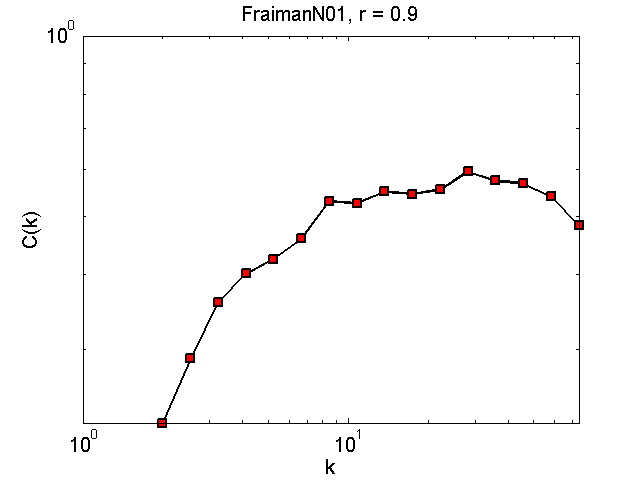
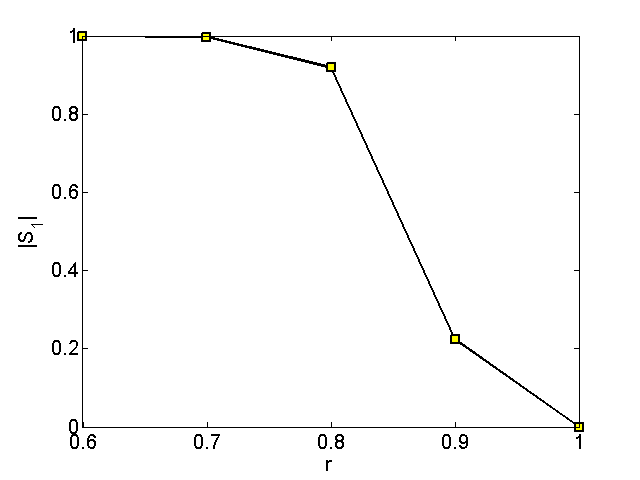
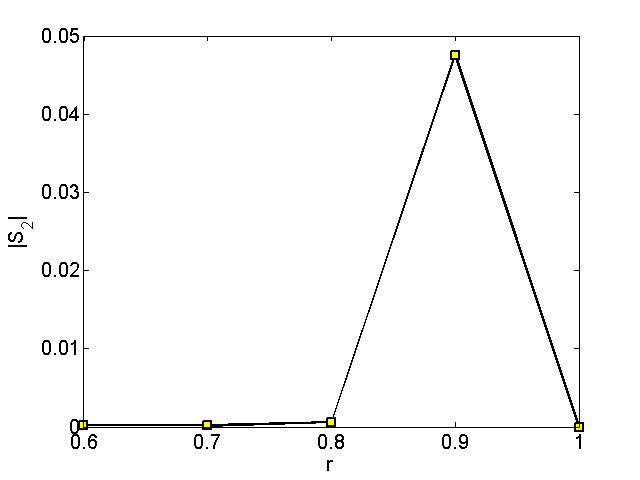
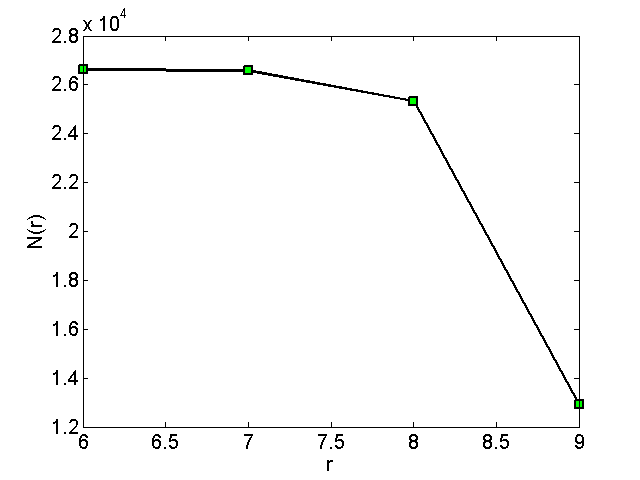
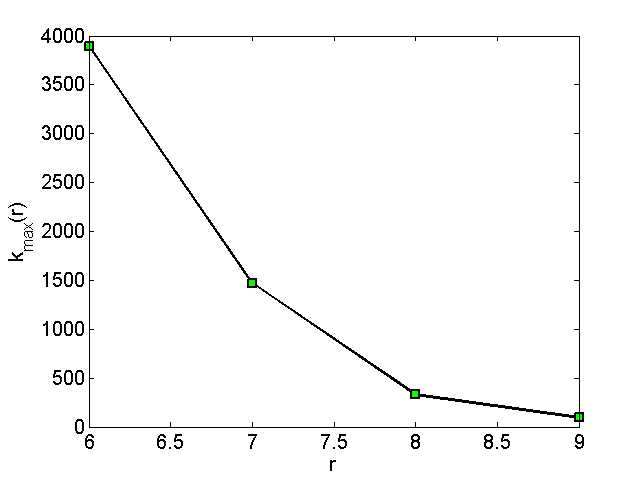
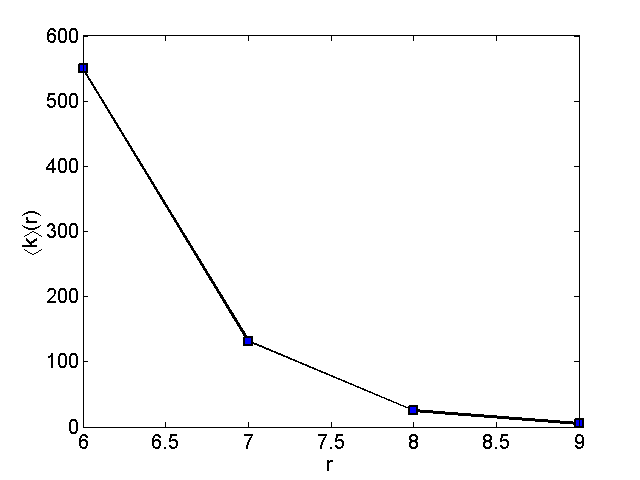
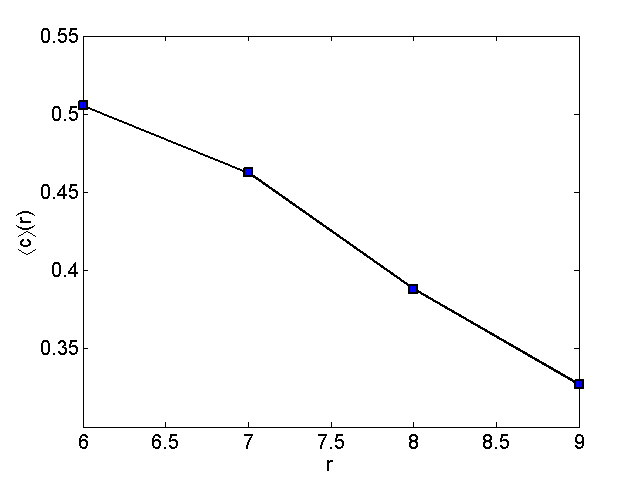
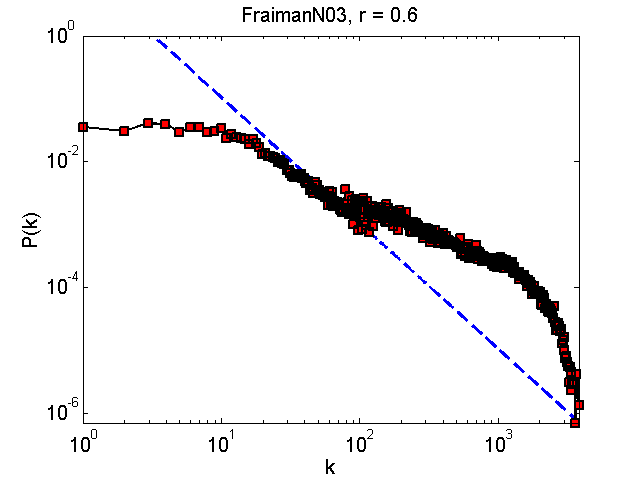
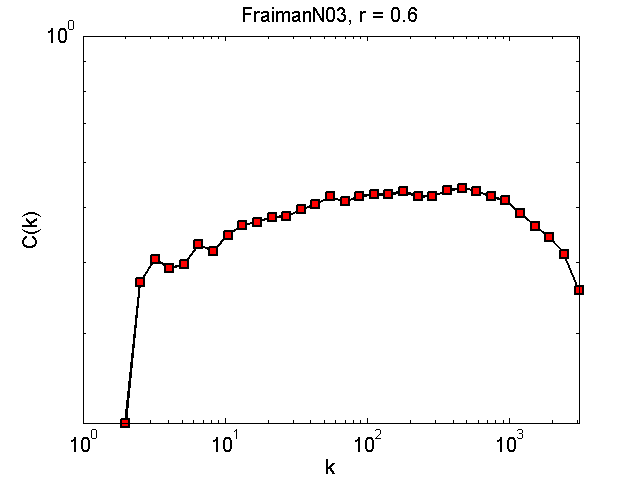
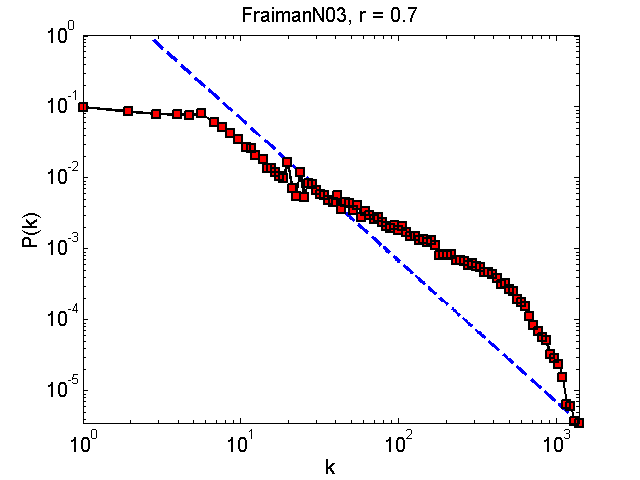
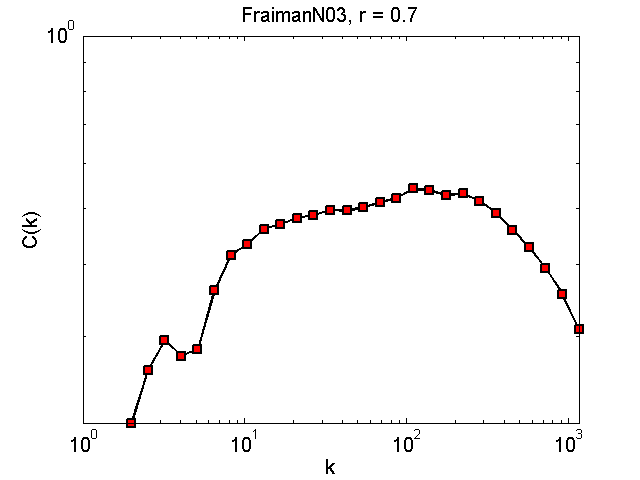
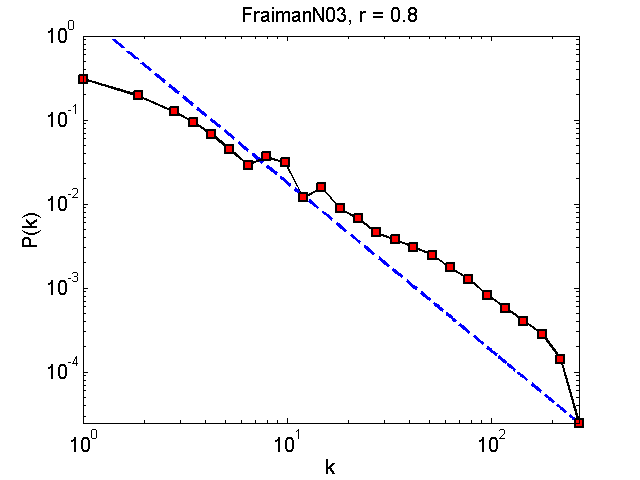
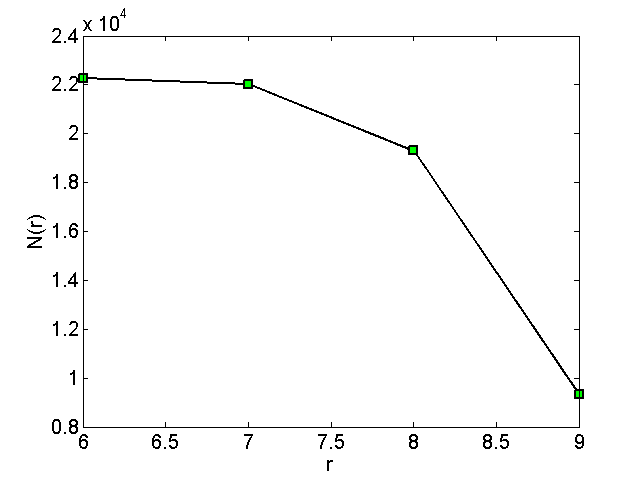
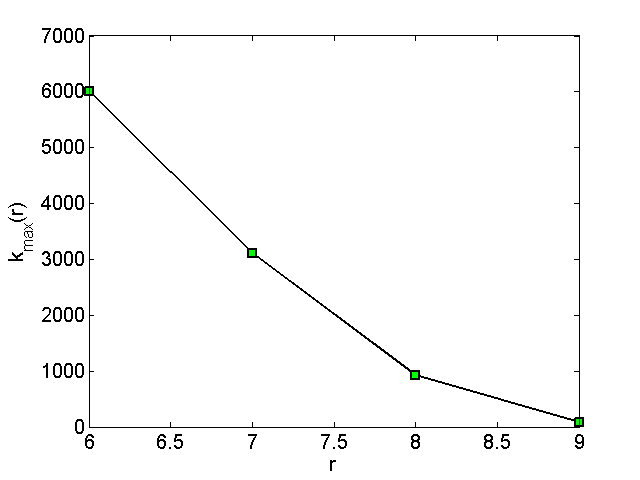
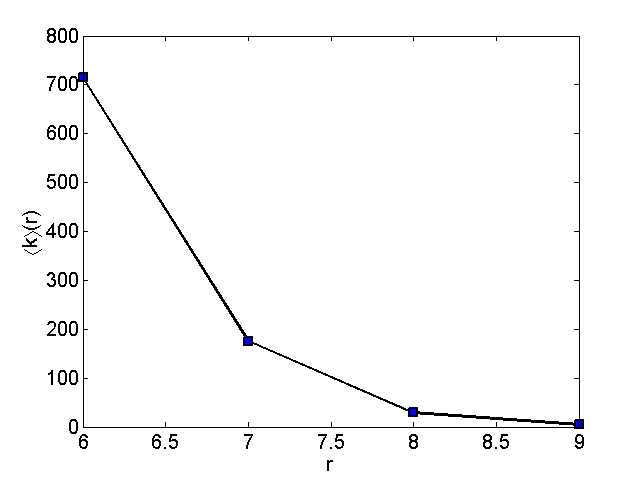
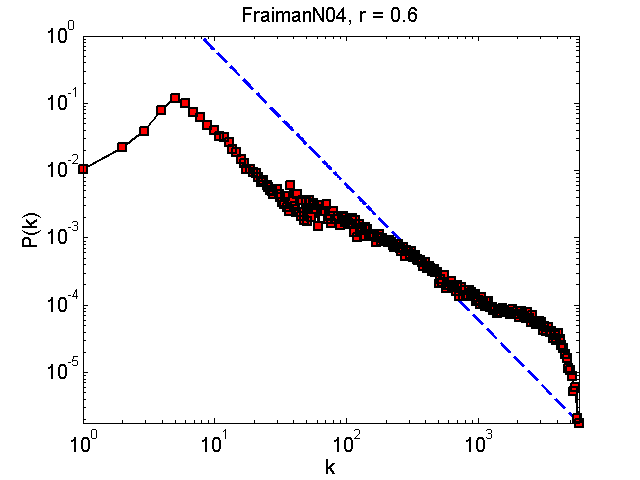
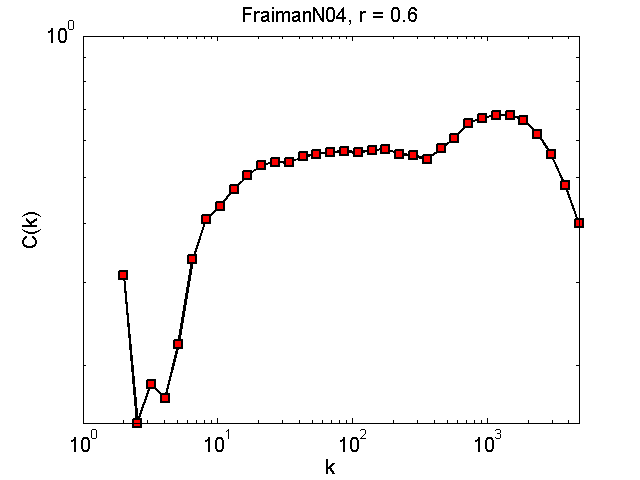
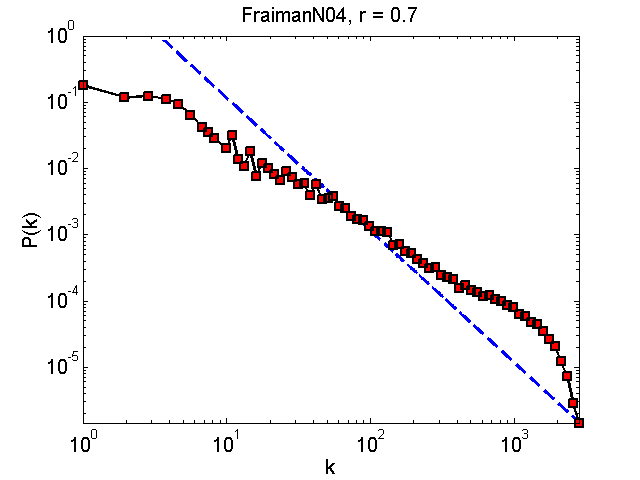
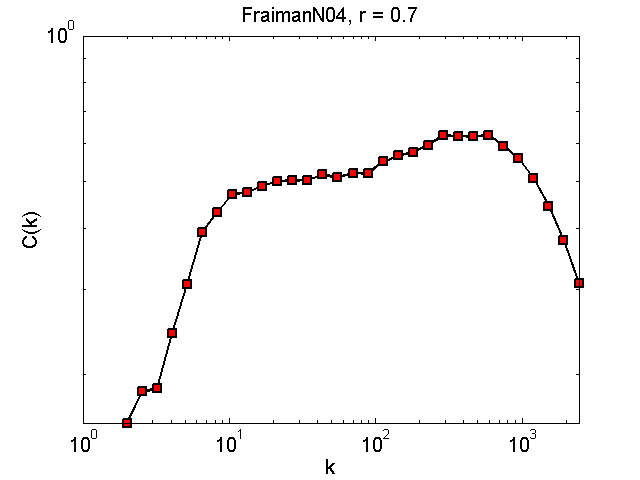
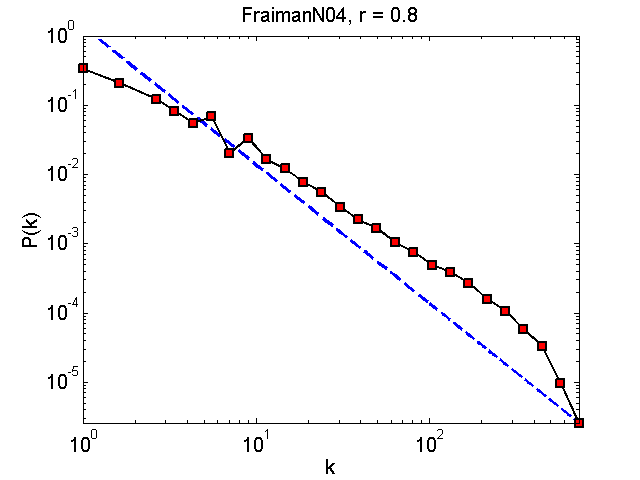
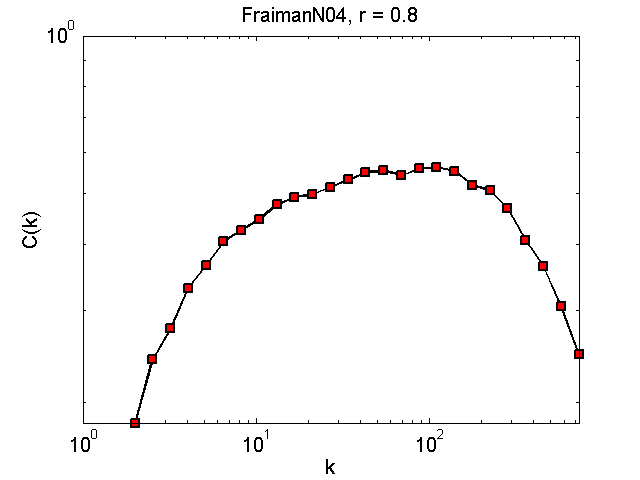
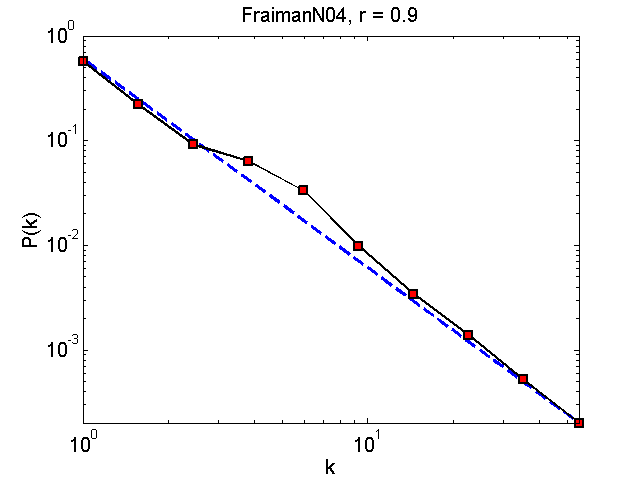
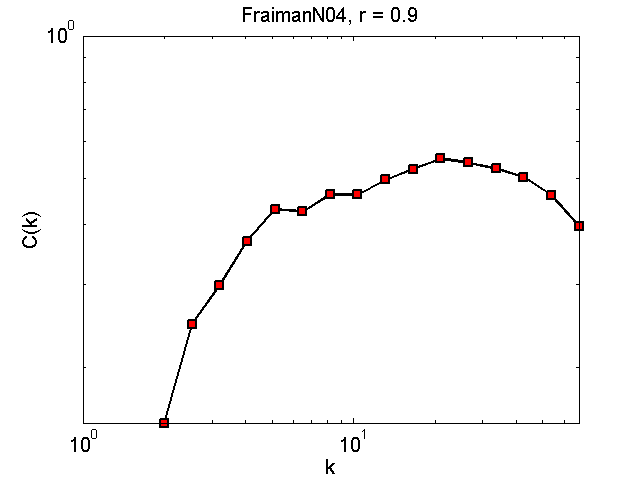
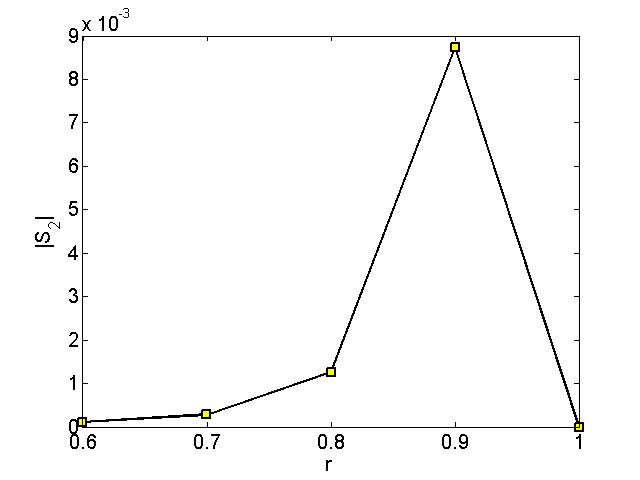
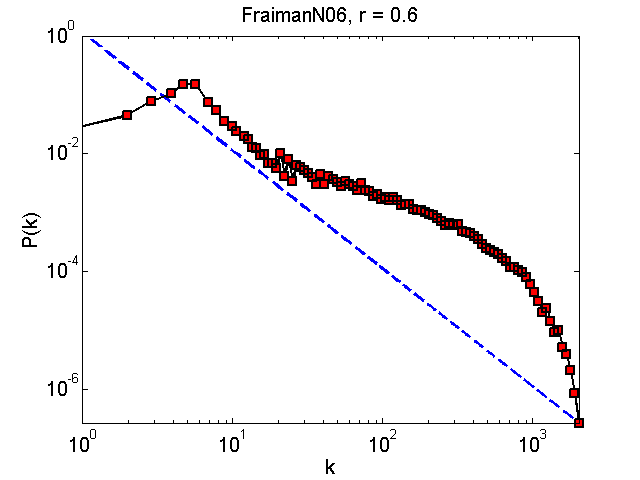
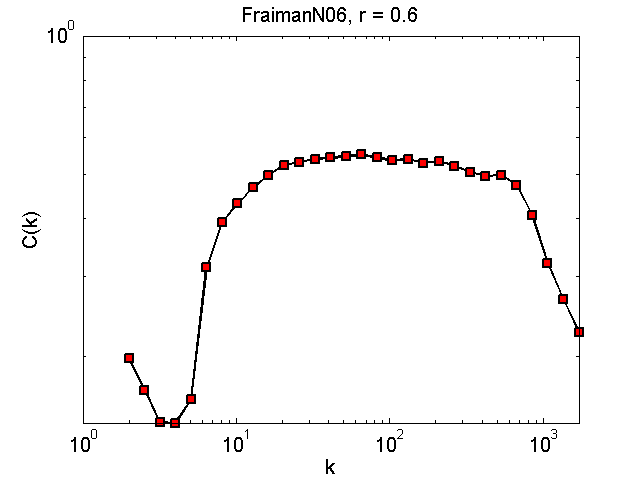
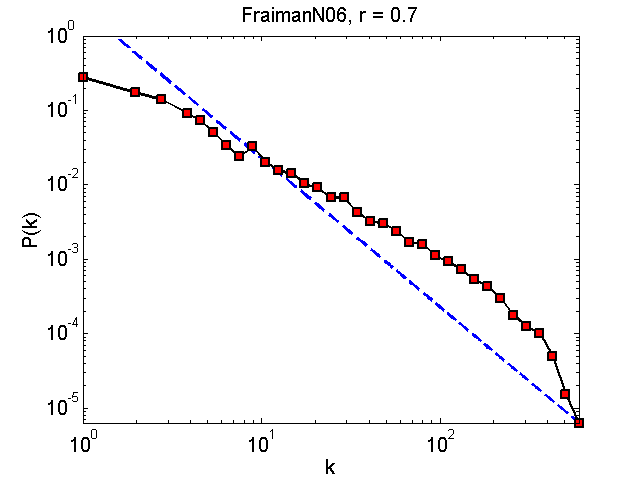
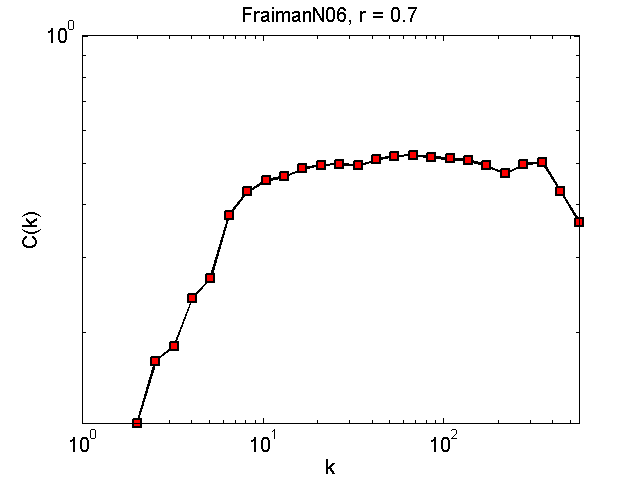
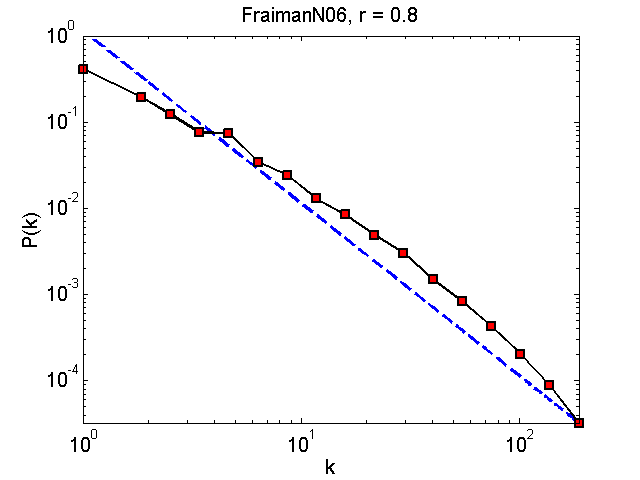
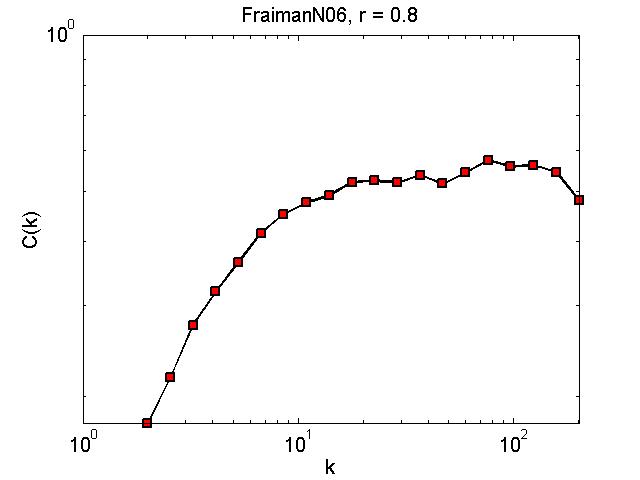
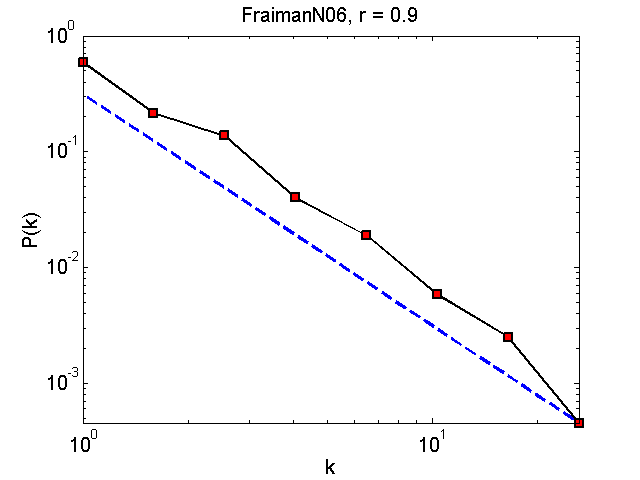
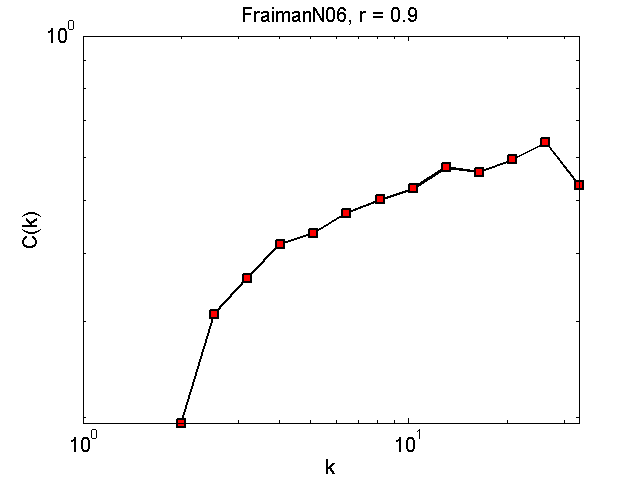
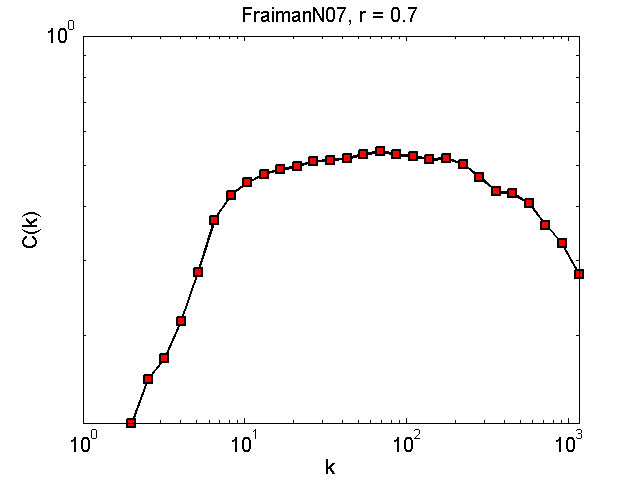
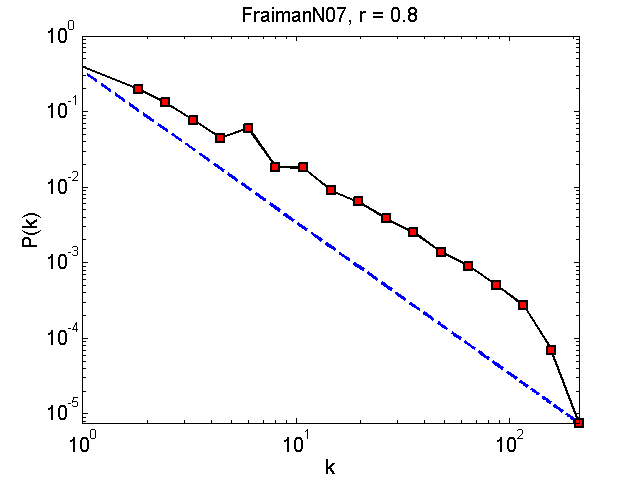
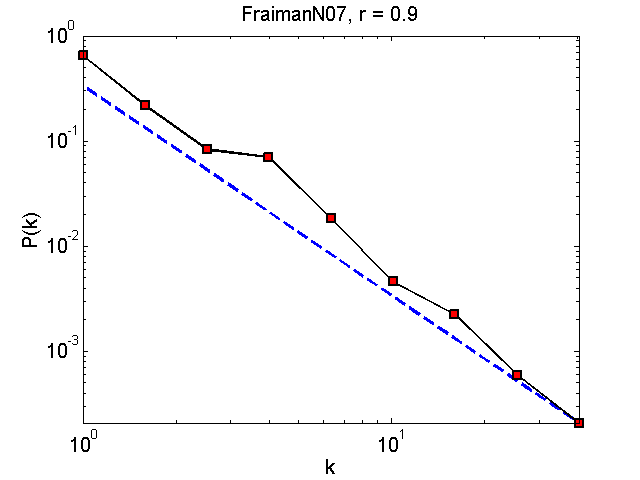
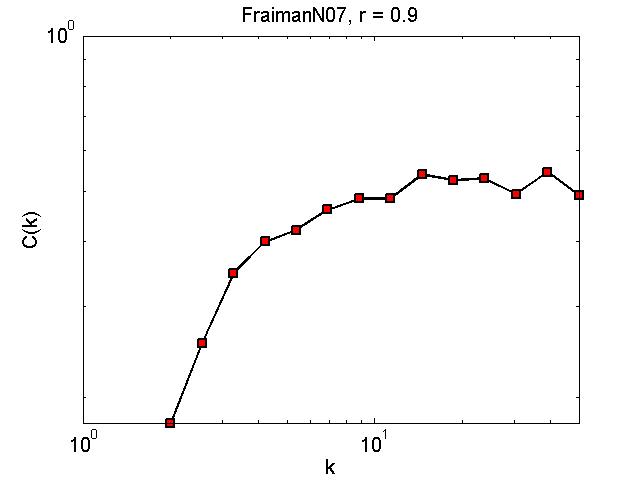
Each block shows the data for a single dataset "SetXY" from the paper Scale-Free Brain Functional Networks, where X is the subject number and Y is the experiment number for X. The functional tasks that the subjects are performing in each experiment are described in info.txt. The only experiment where the subject is at rest is Set14. 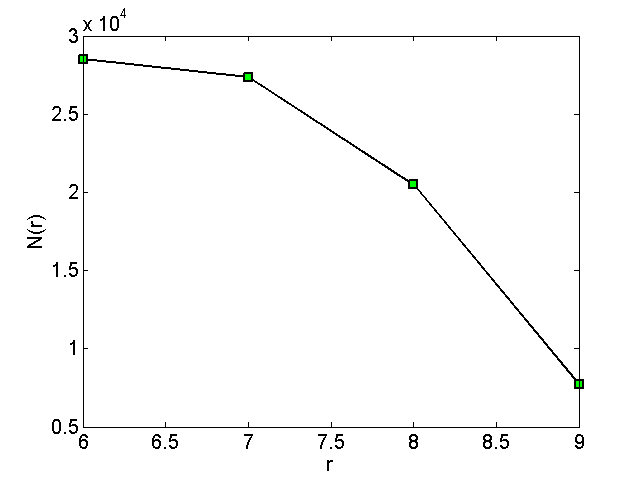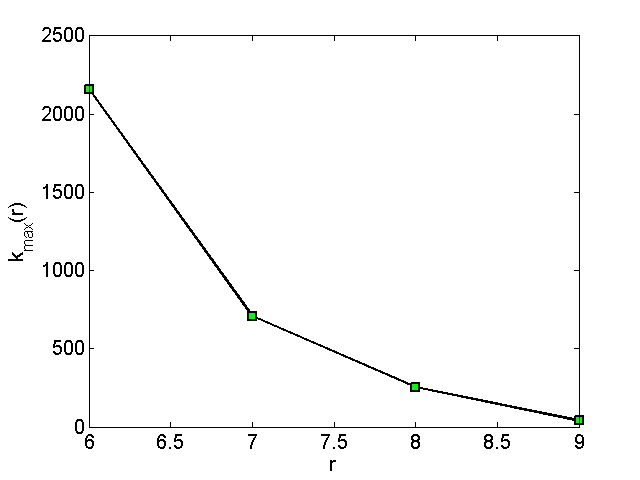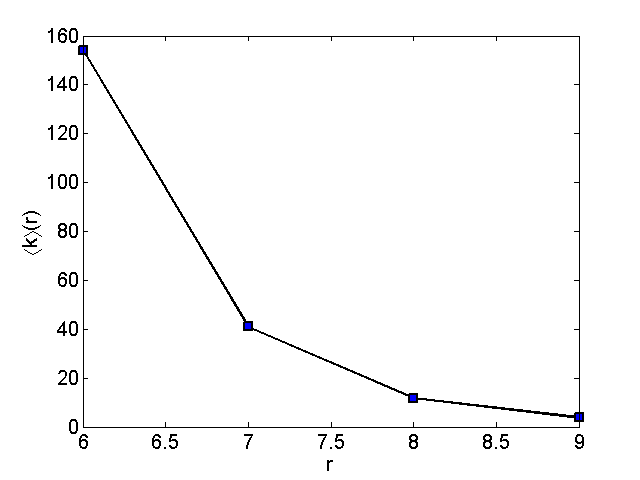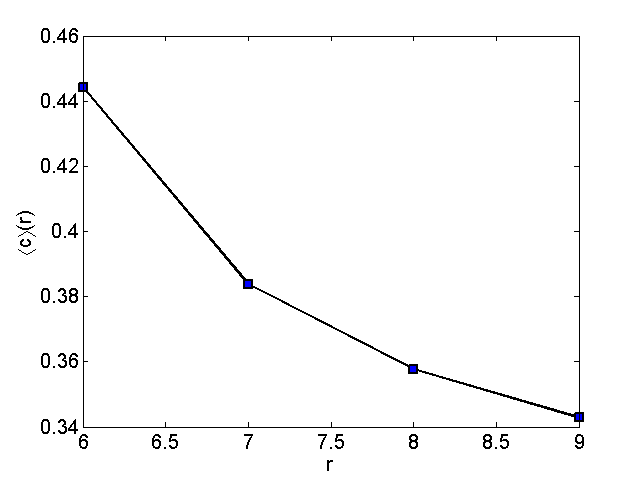At the bottom there are few additional datasets FraimanN0X from the paper Ising-like dynamics in large-scale functional brain networks. The data are for the graphs obtained from the full correlation matrices by thresholding them with a given correlation threshold, i.e., the link between two voxels exists if the positive correlation between the voxels exceeds the threshold.
Notations:
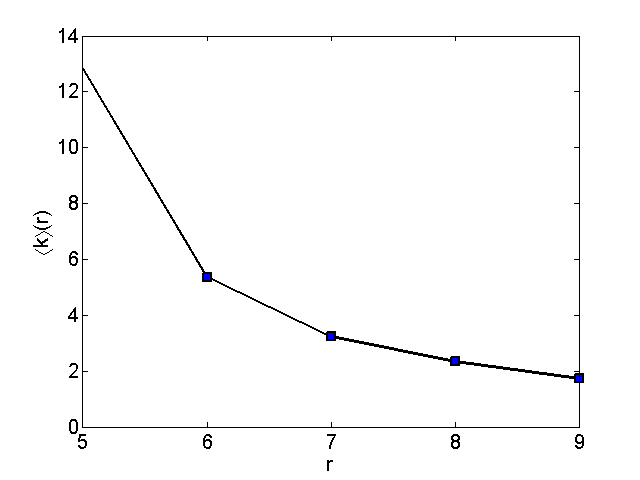
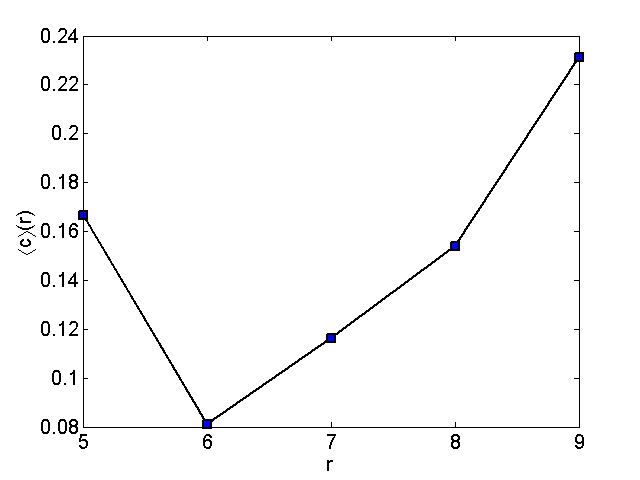
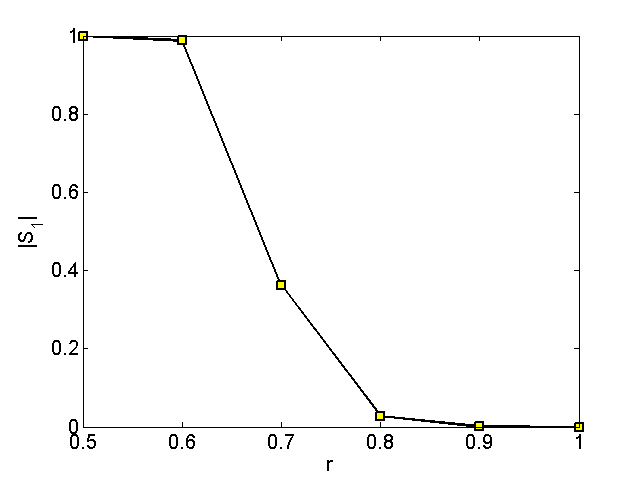
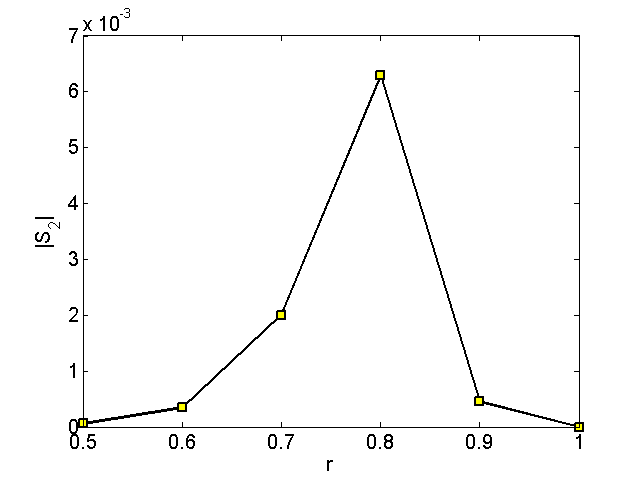
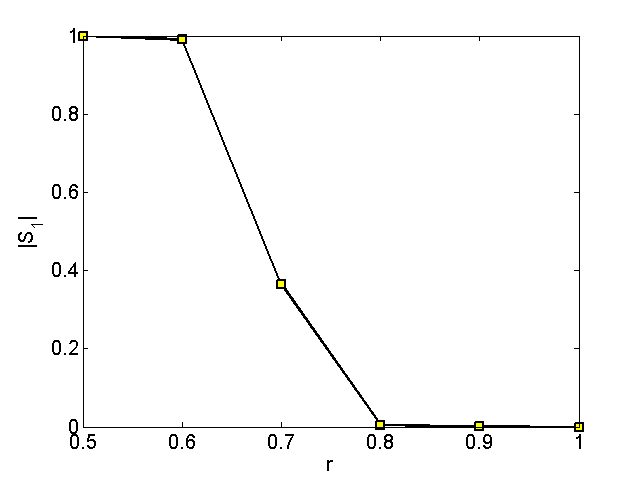
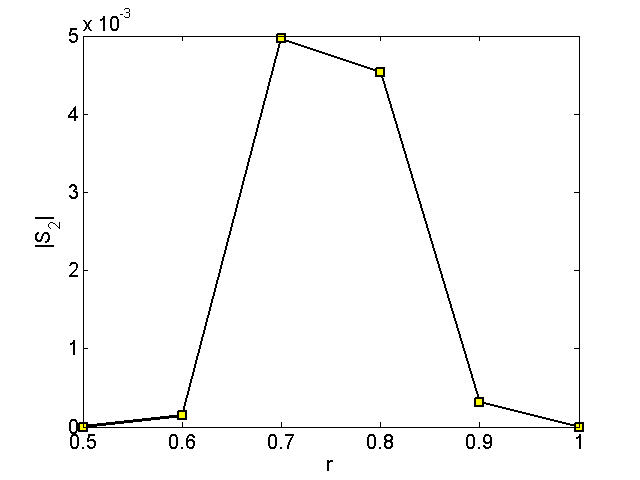
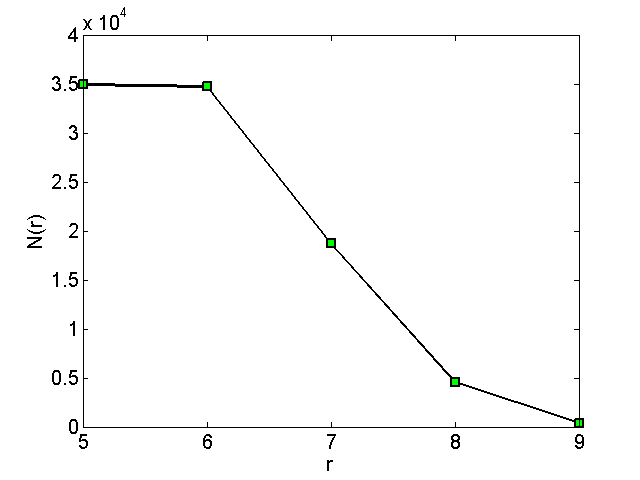
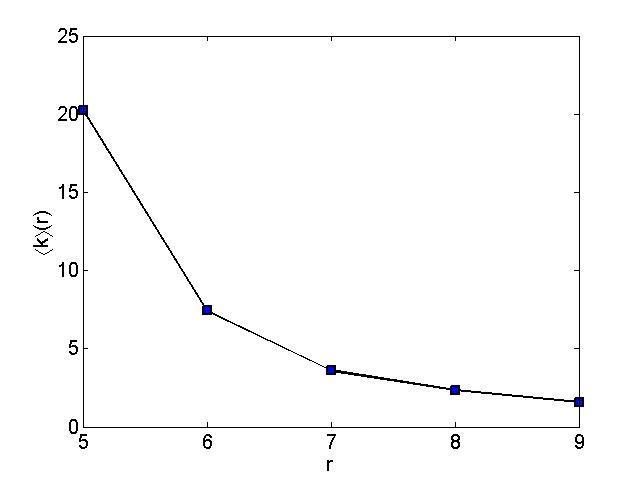
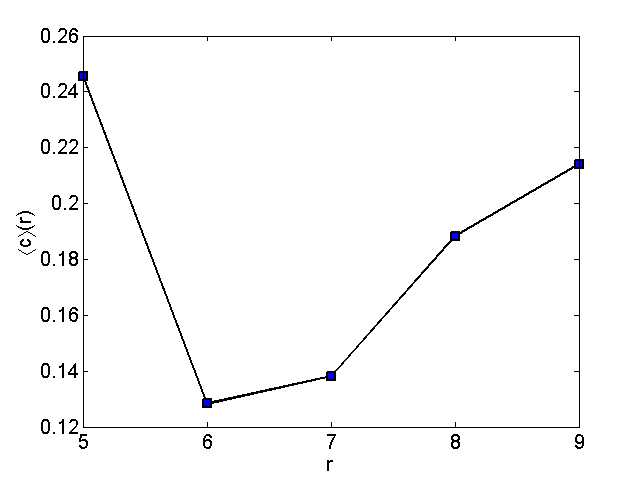
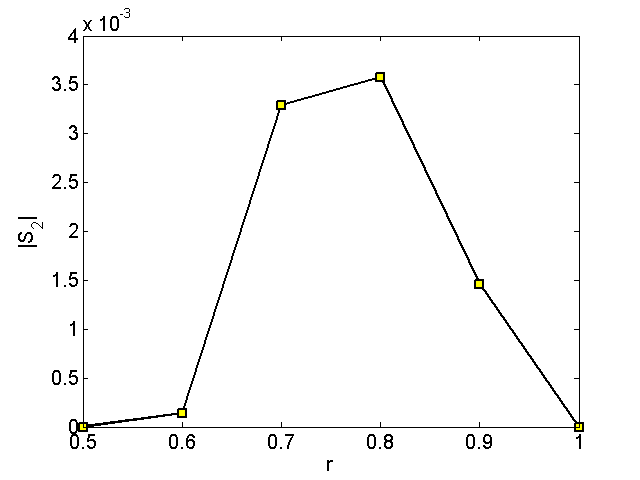
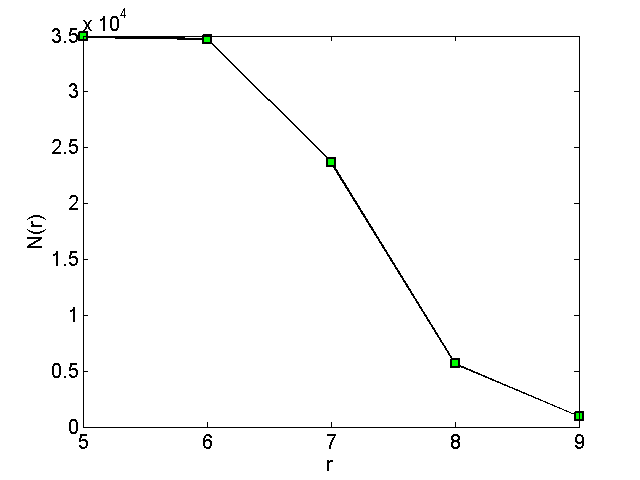
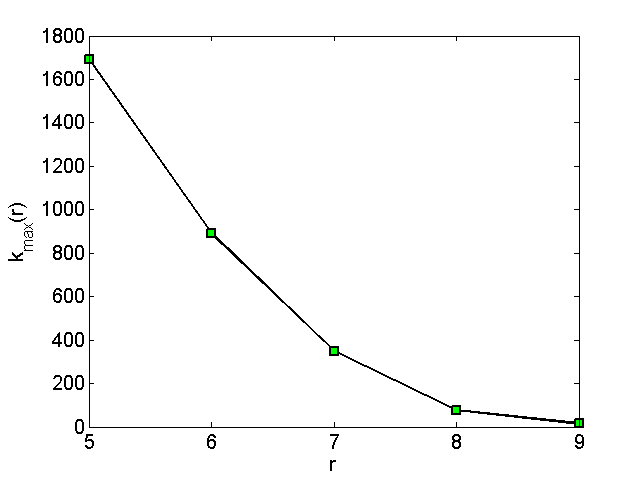
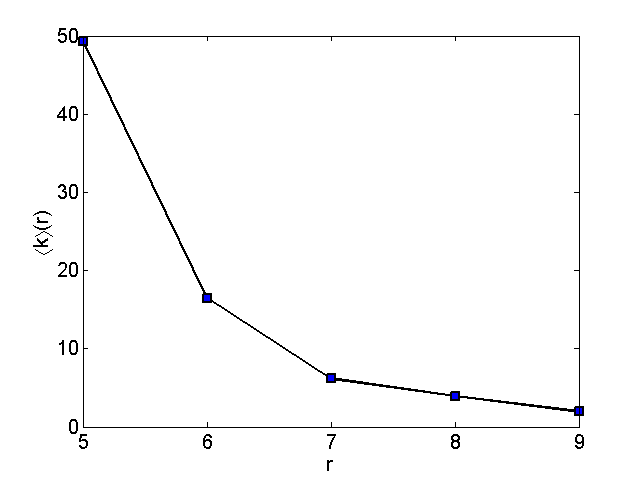
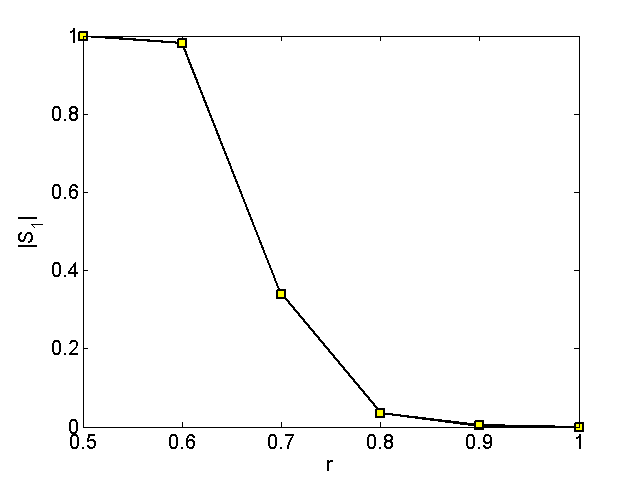
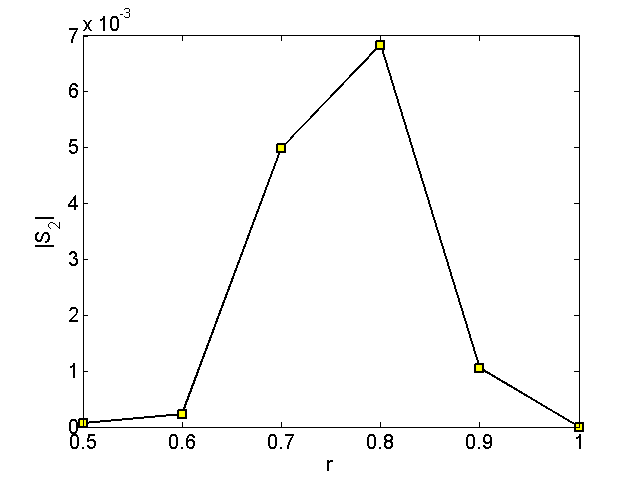
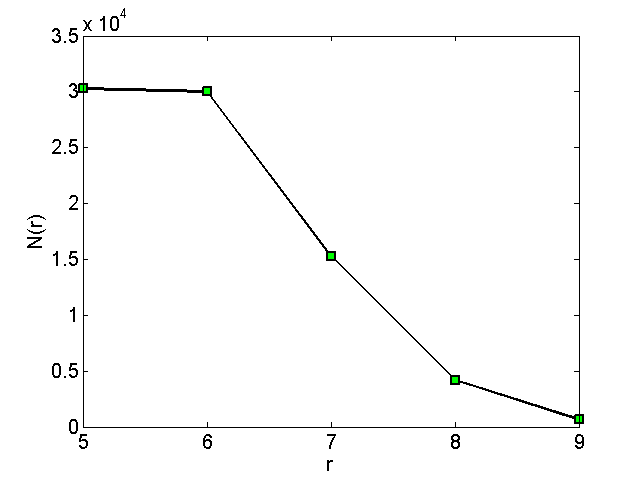
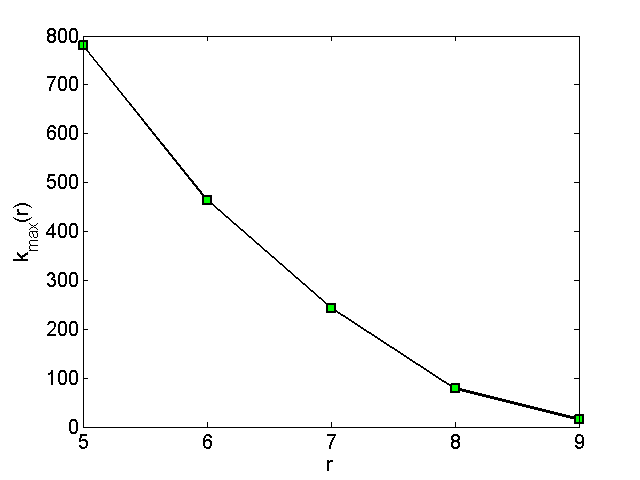
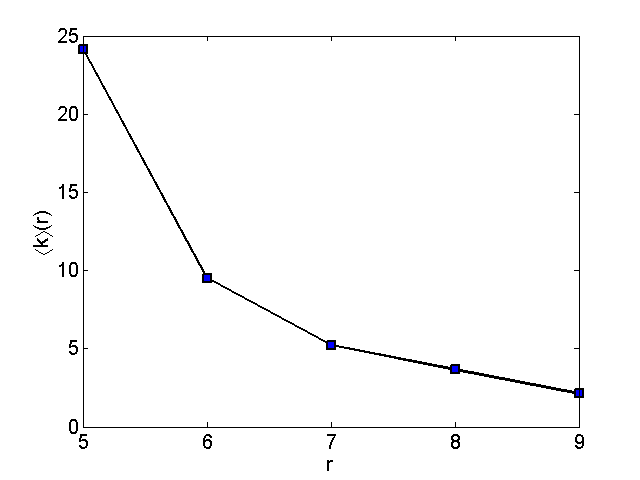
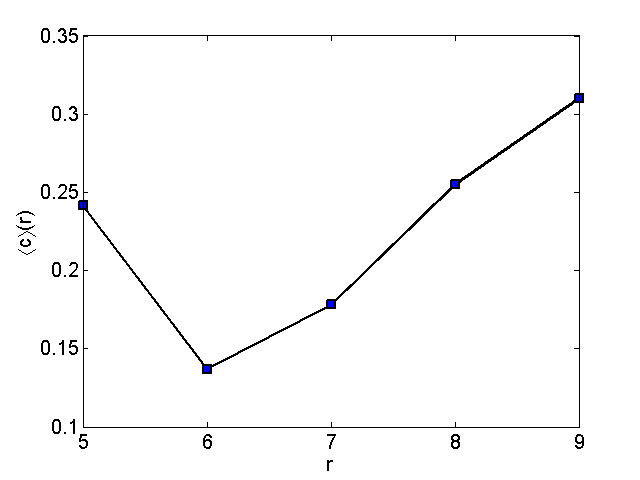
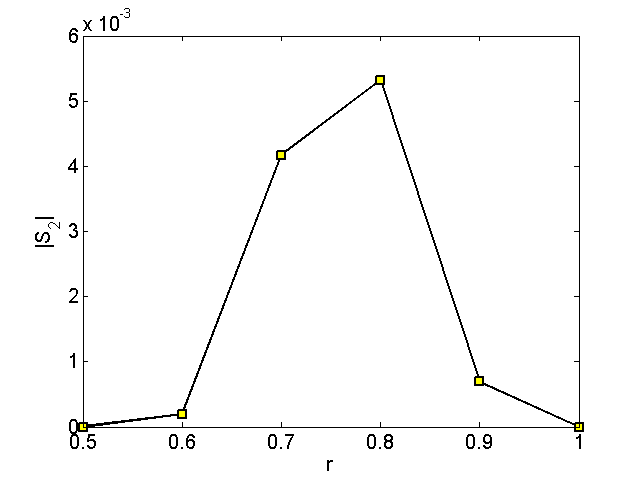
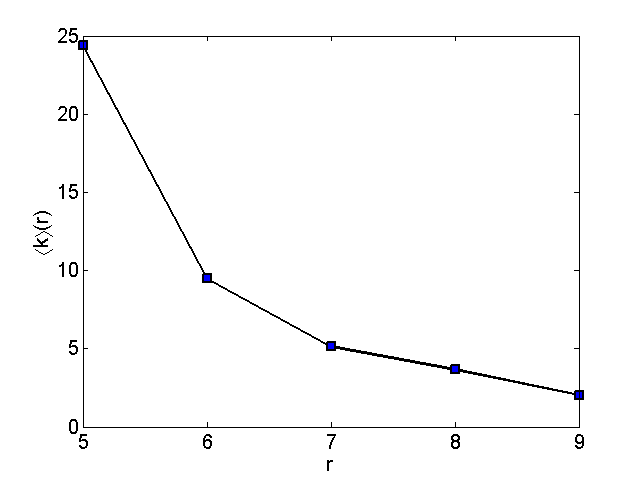
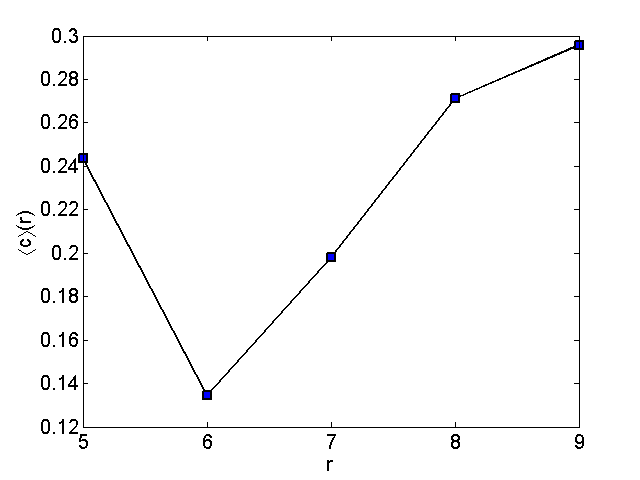
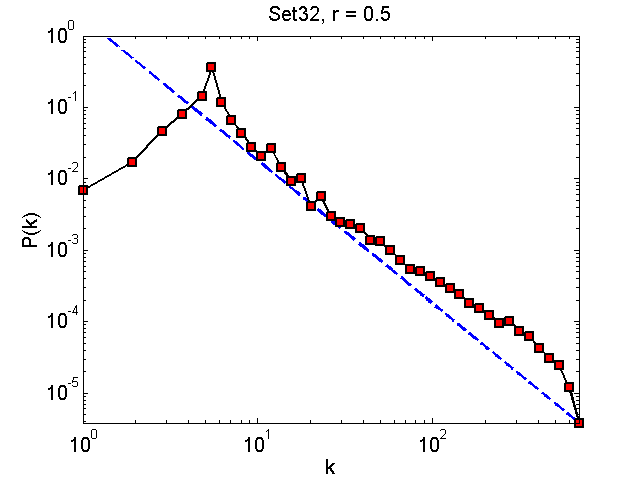
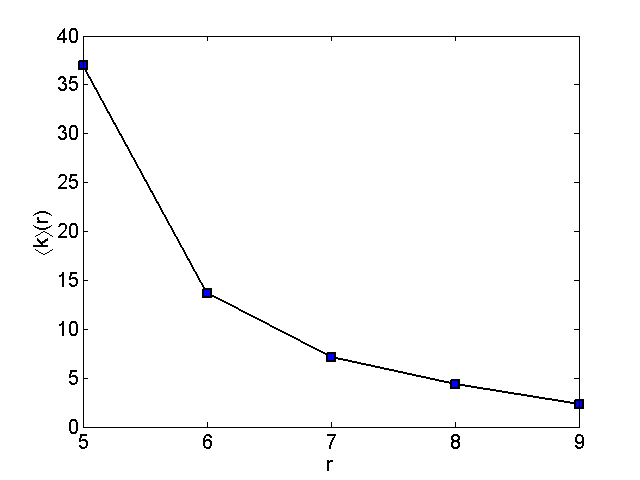
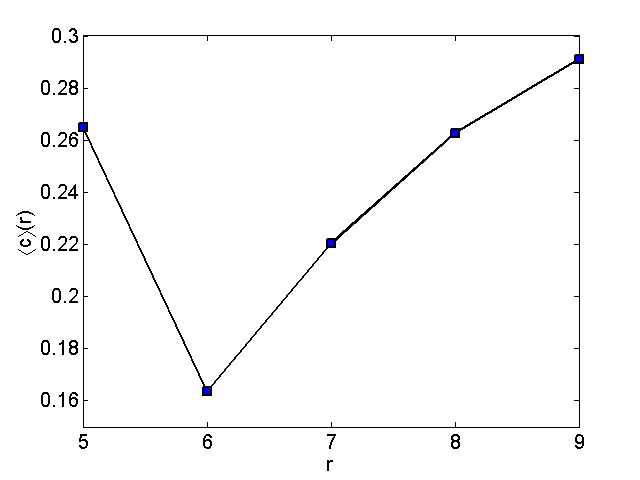
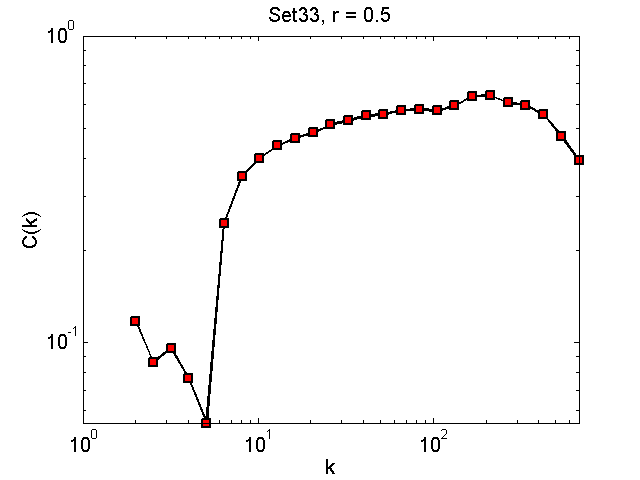
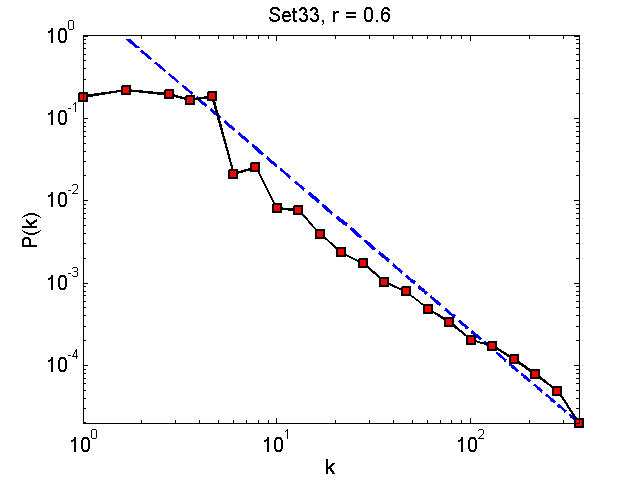
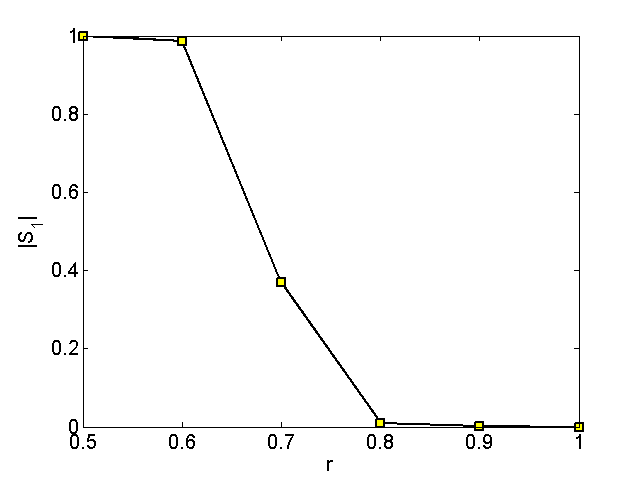
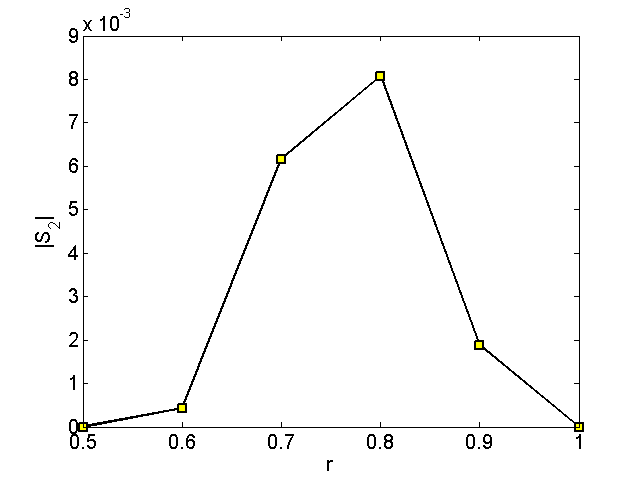
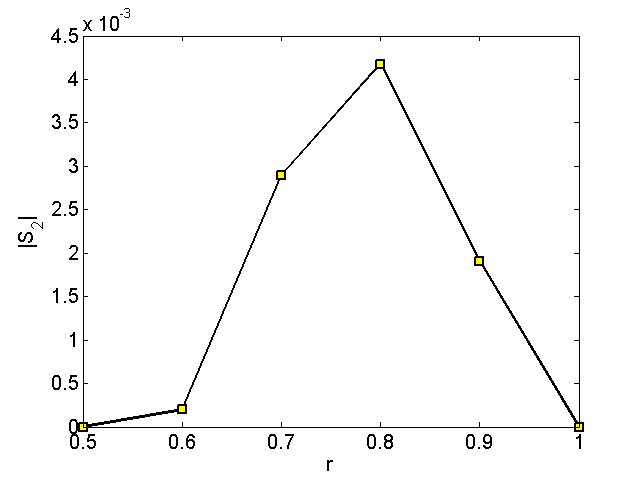
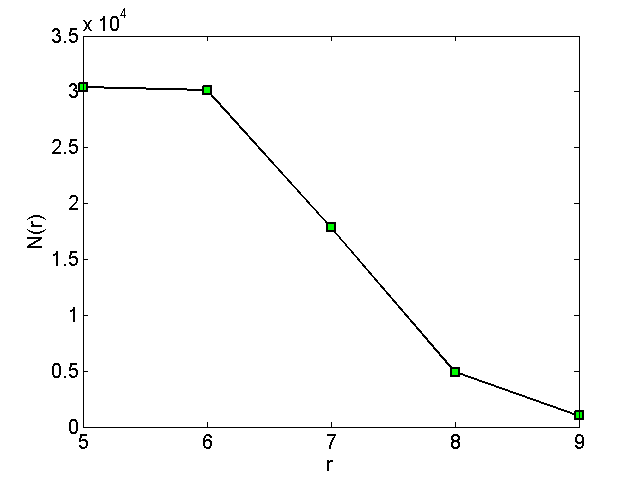
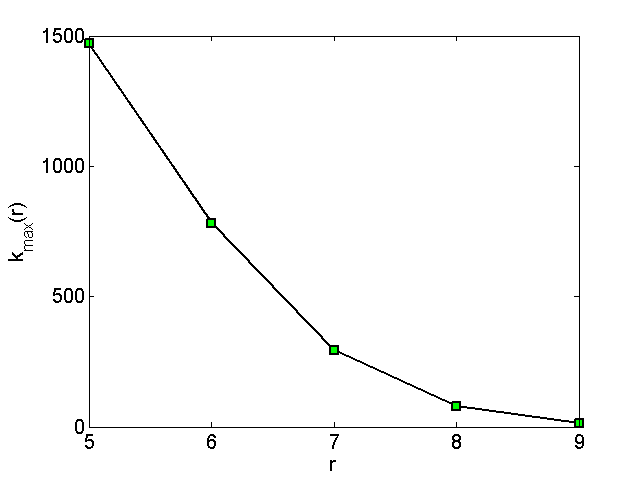
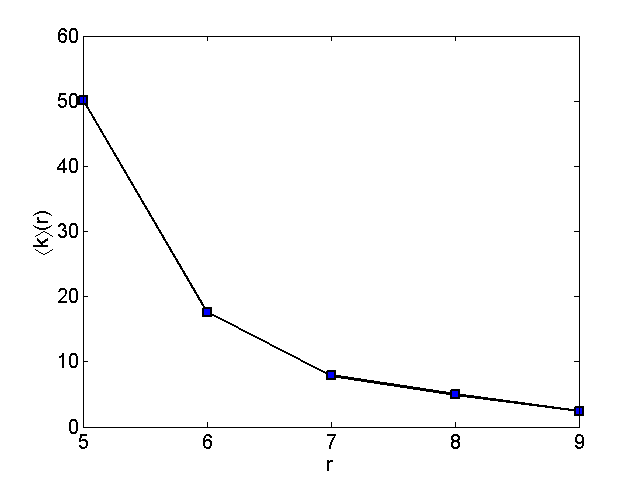
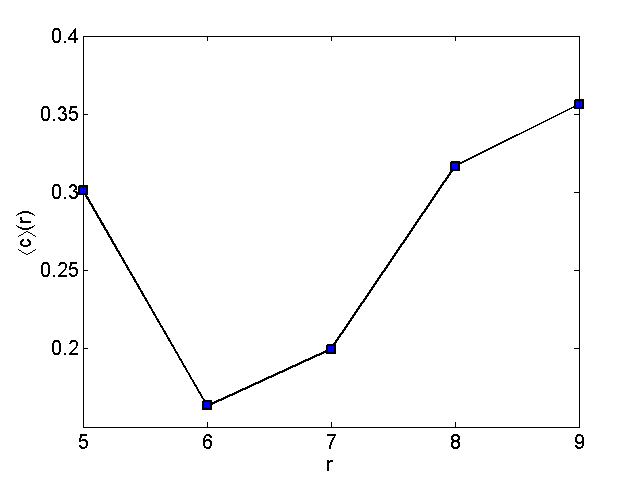
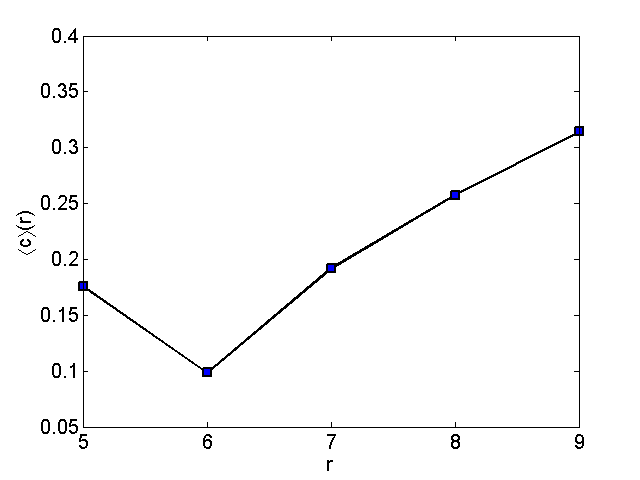
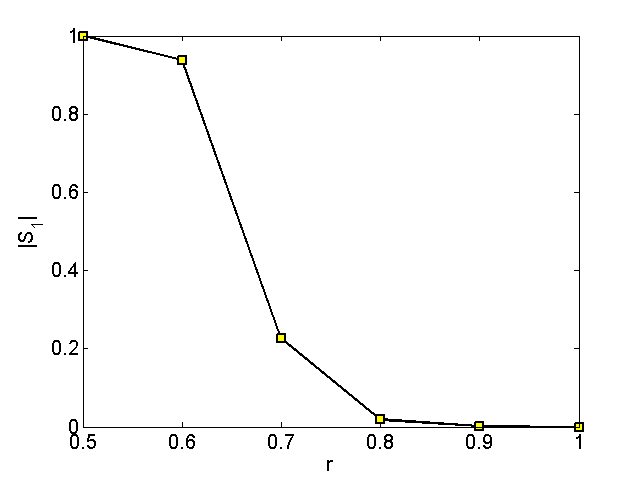
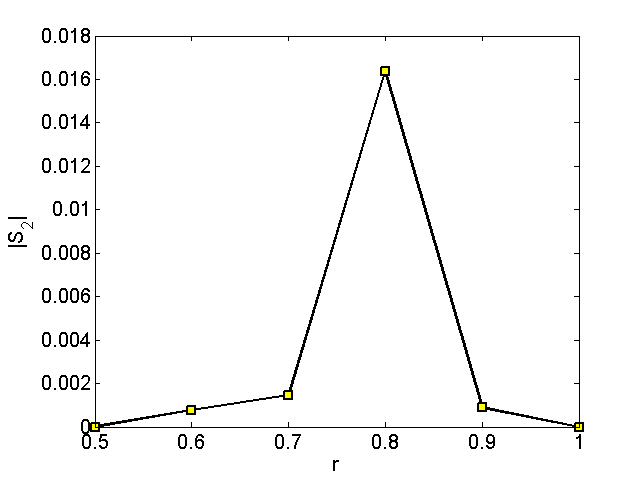
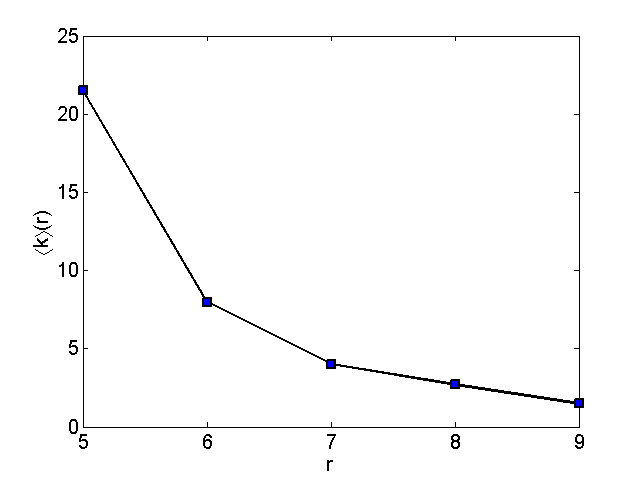
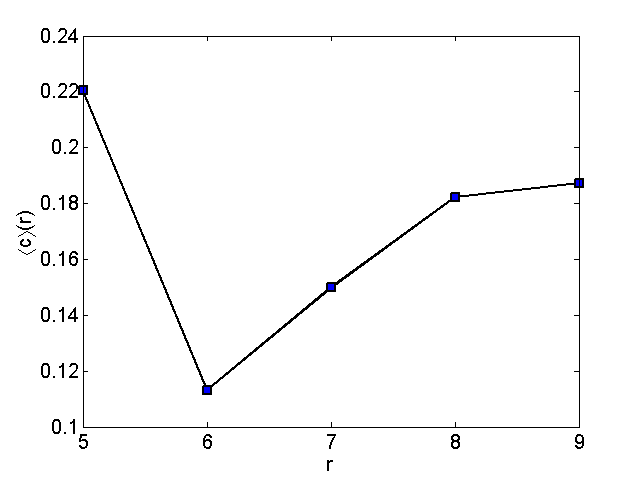
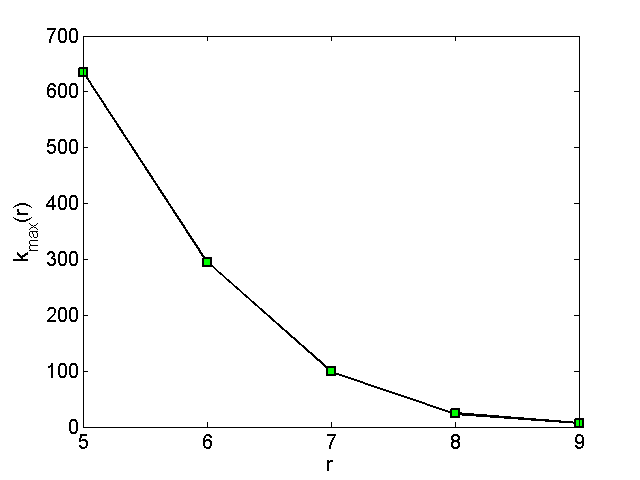
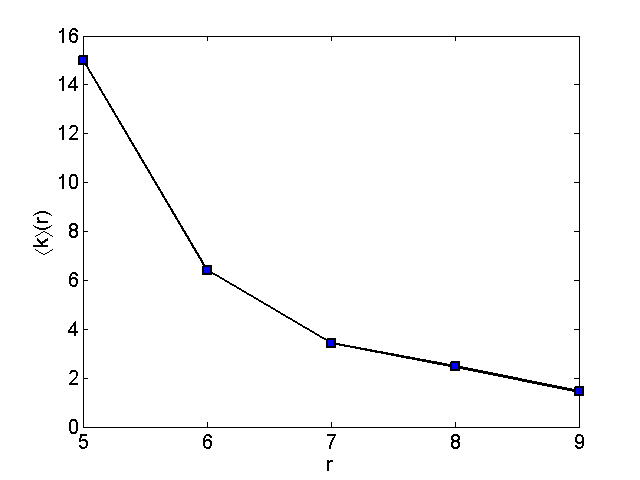
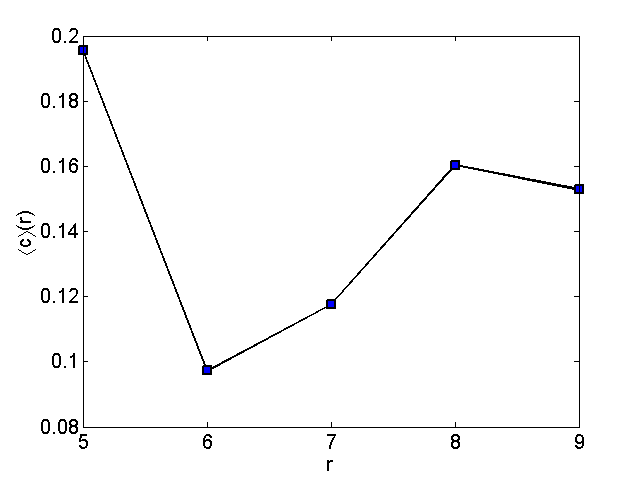
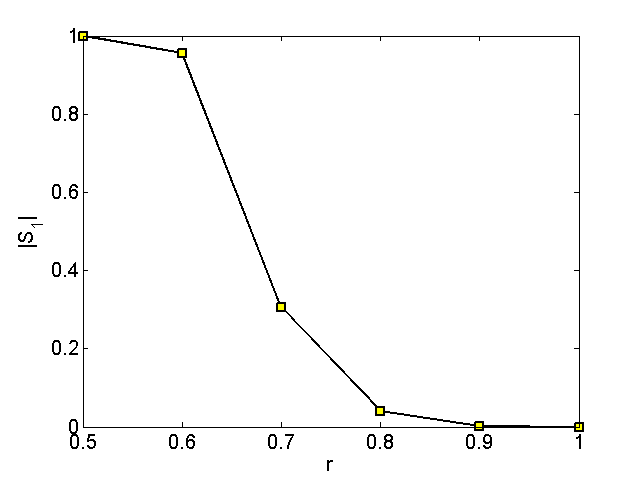
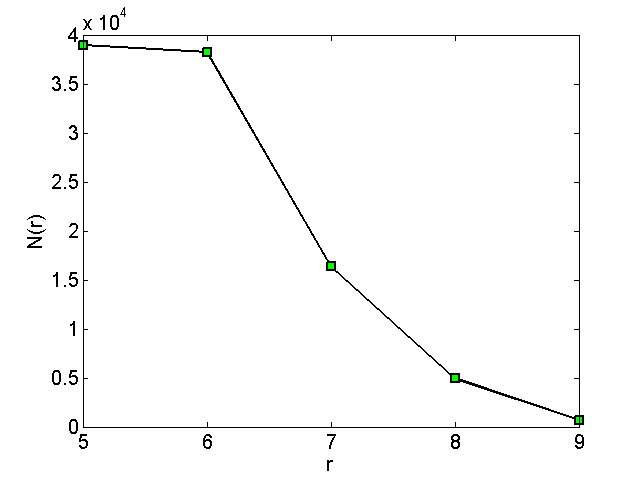
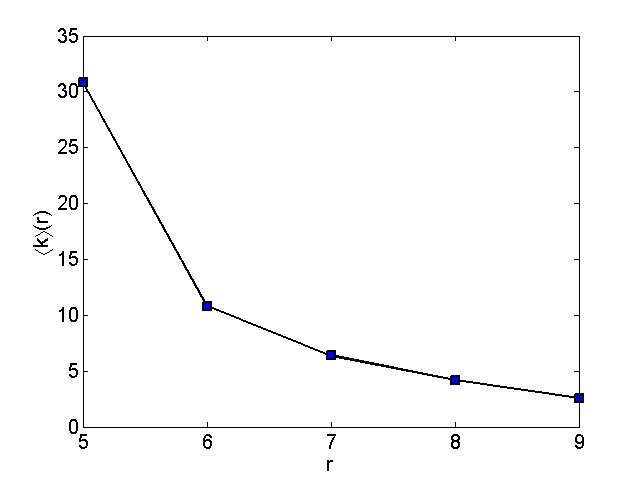
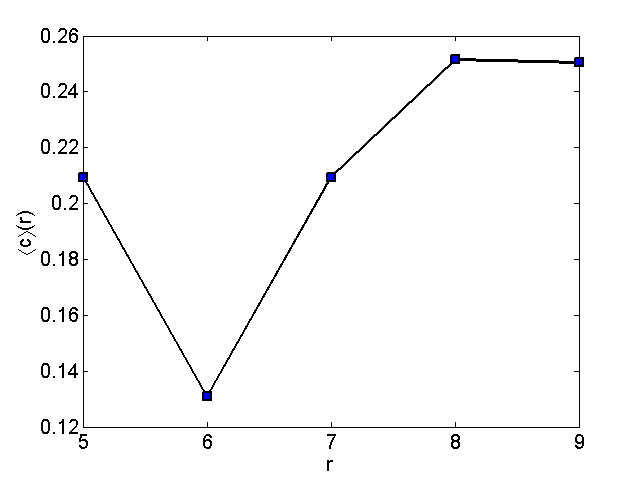
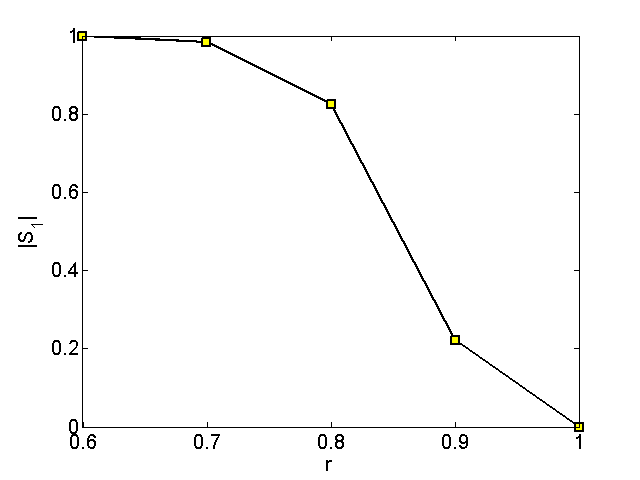
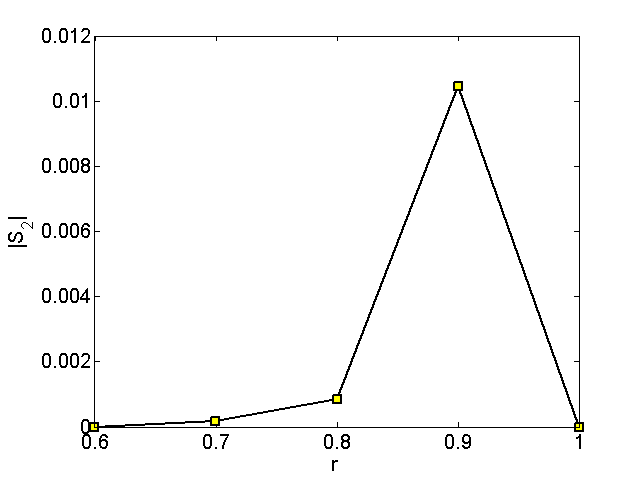
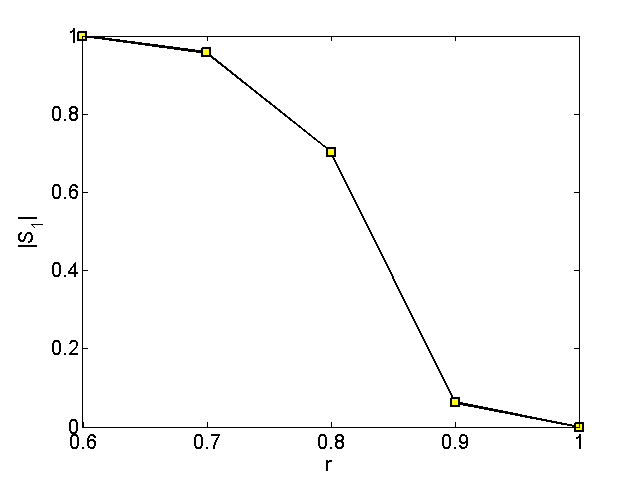
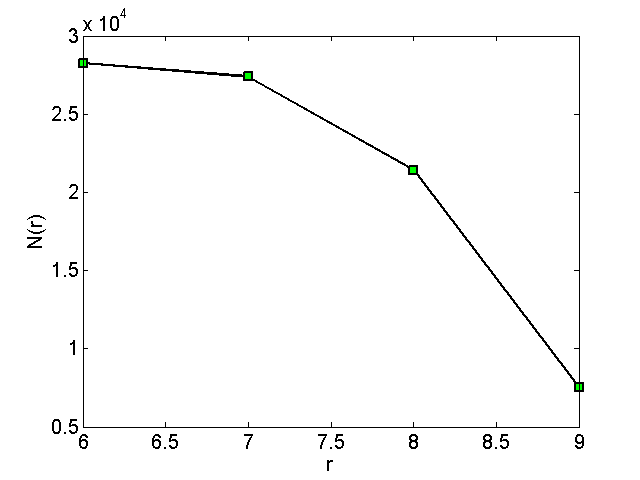
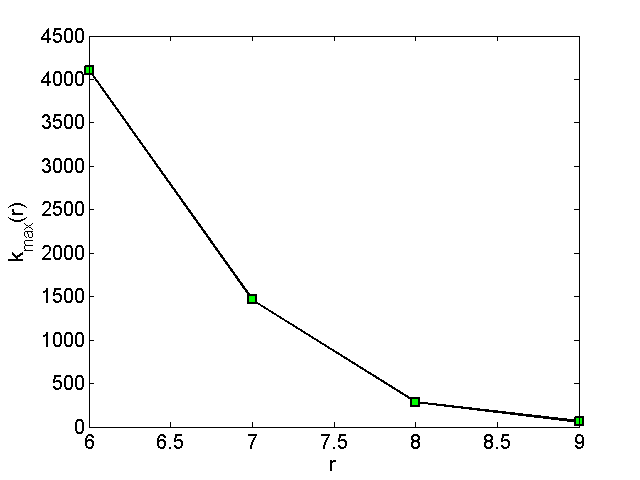
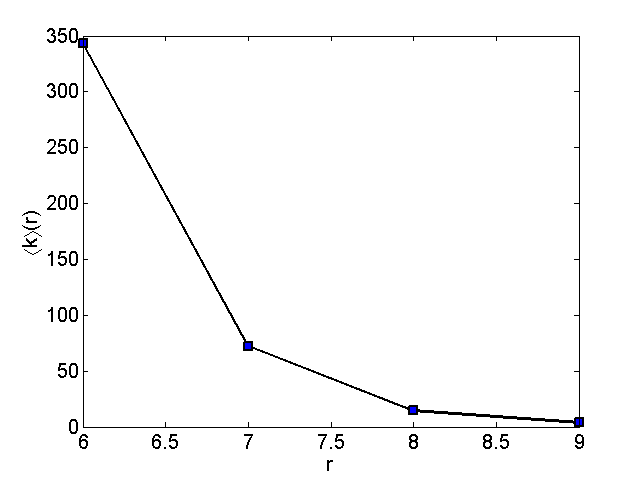
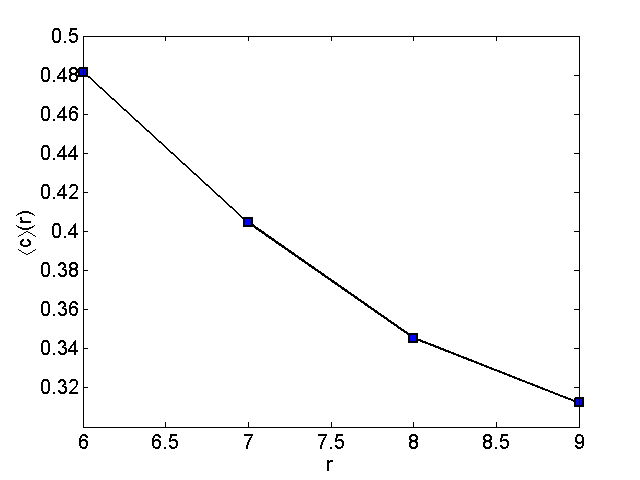
- r -- correlation threshold
- |S1| -- relative size of the largest connected component
- |S2| -- relative size of the second largest connected component
- N -- graph size
- kmax -- largest degree
- <k> -- average degree
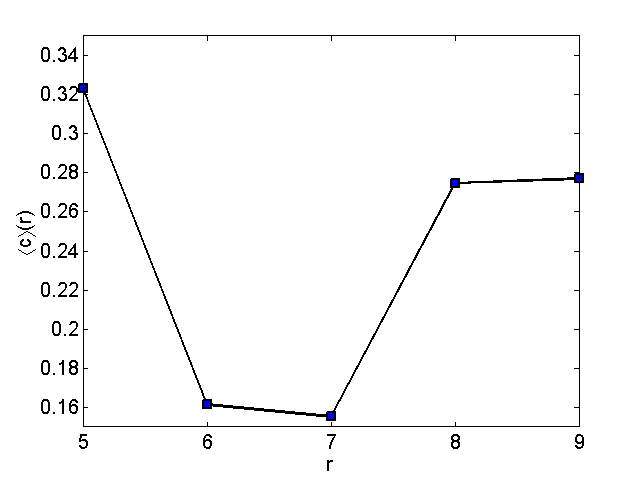
- <c> -- average clustering excluding nodes of degree 1
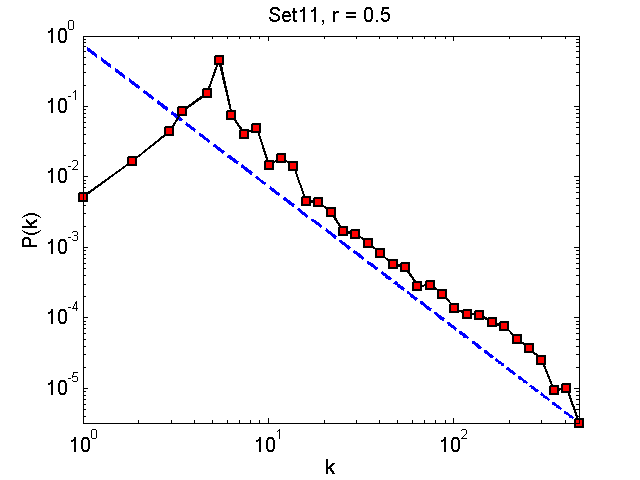
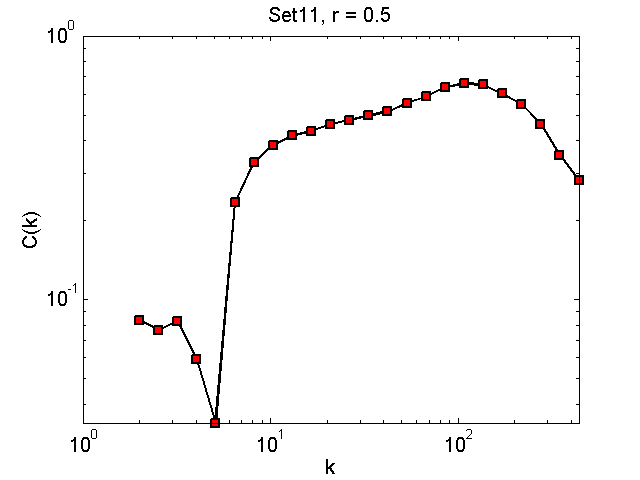
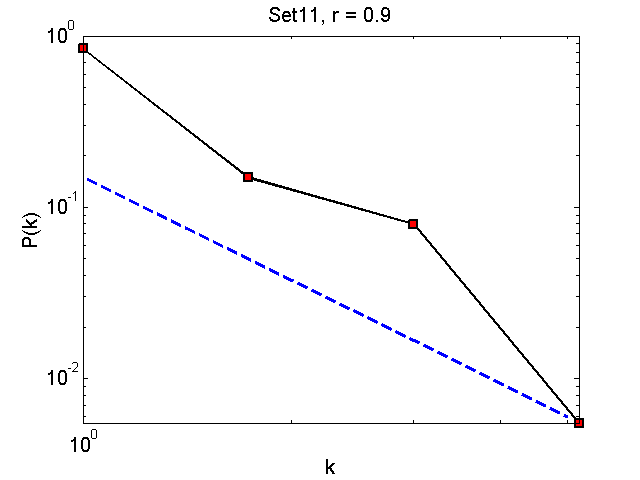
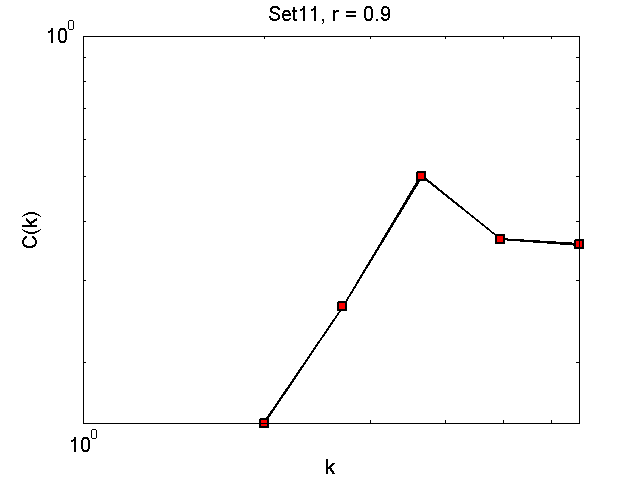
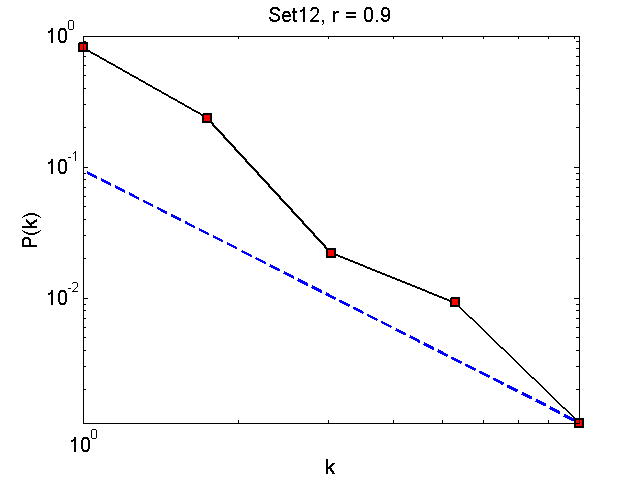
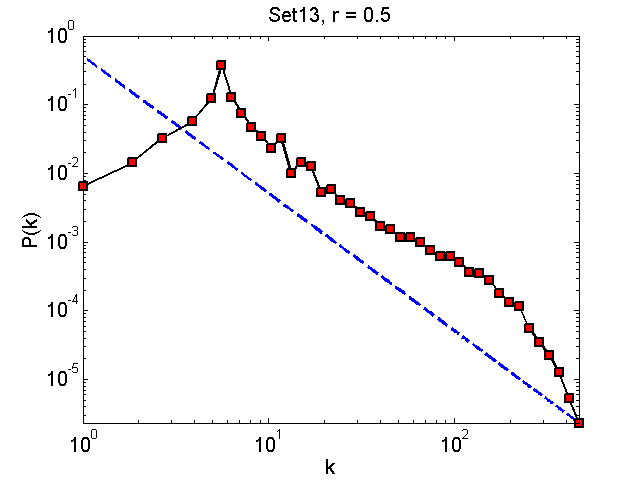
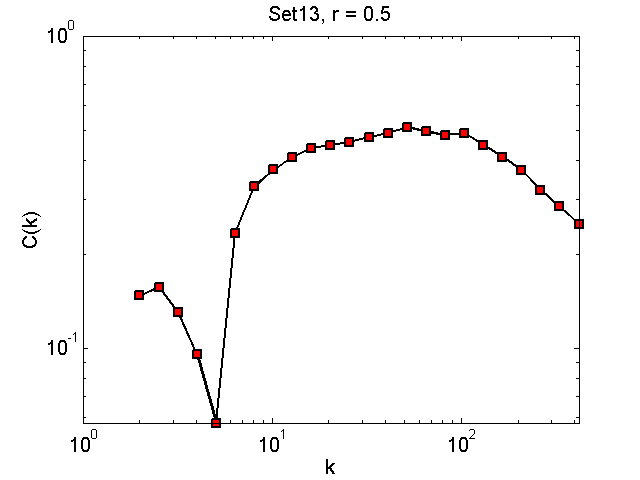
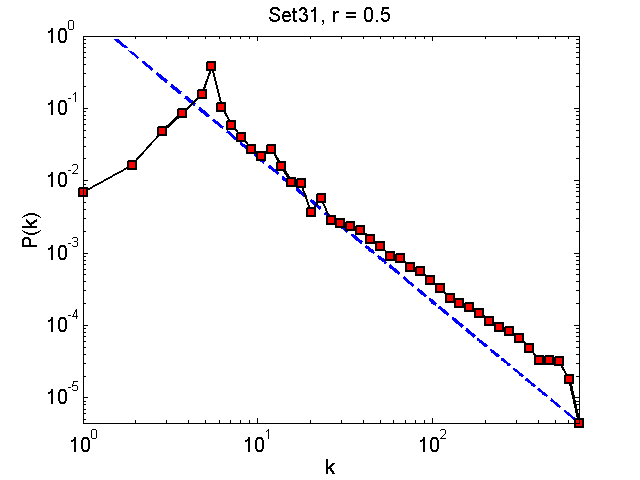
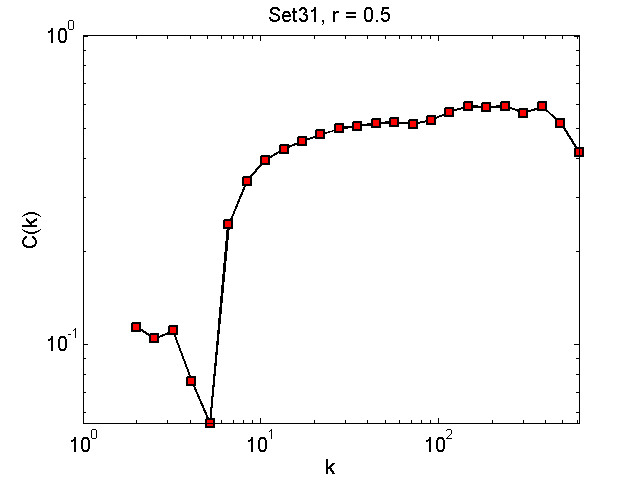
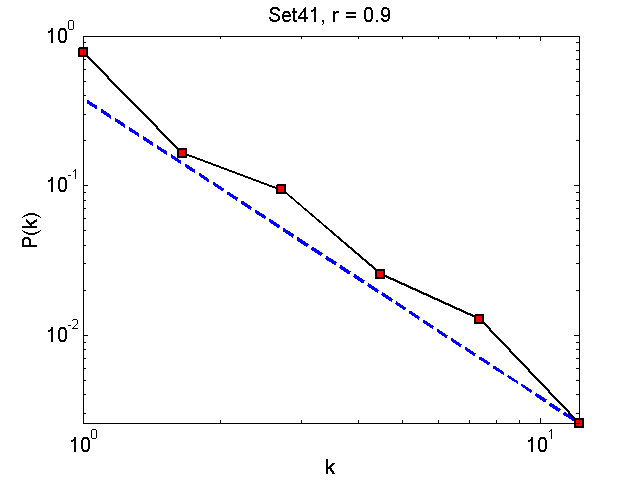
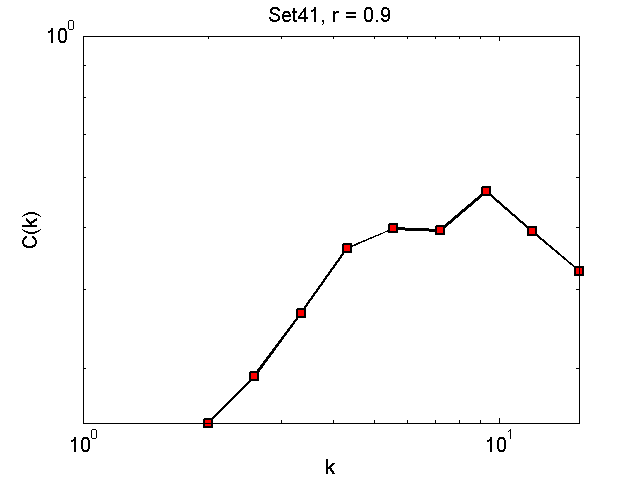
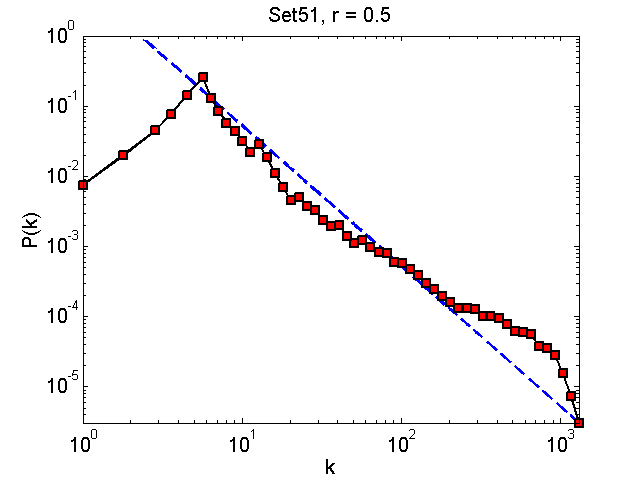
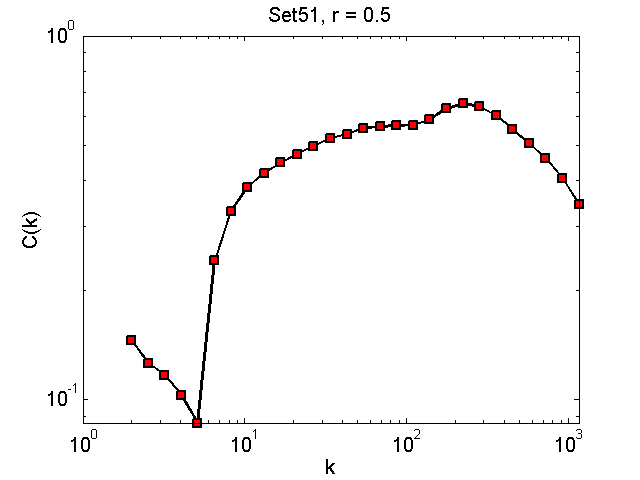
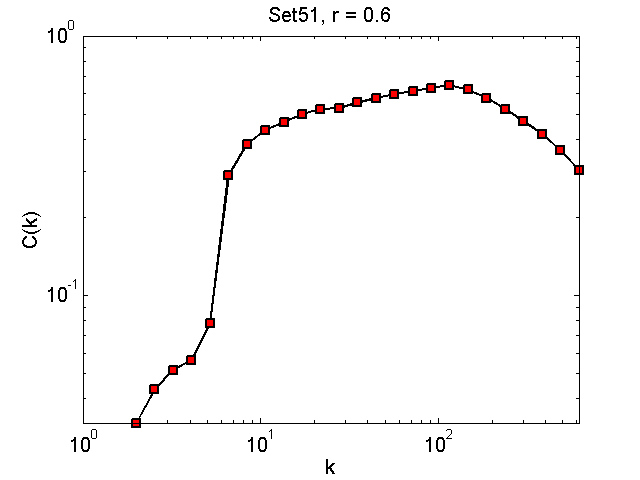
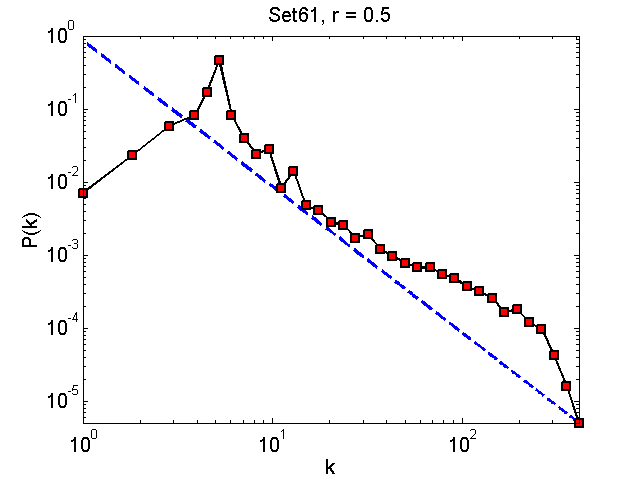
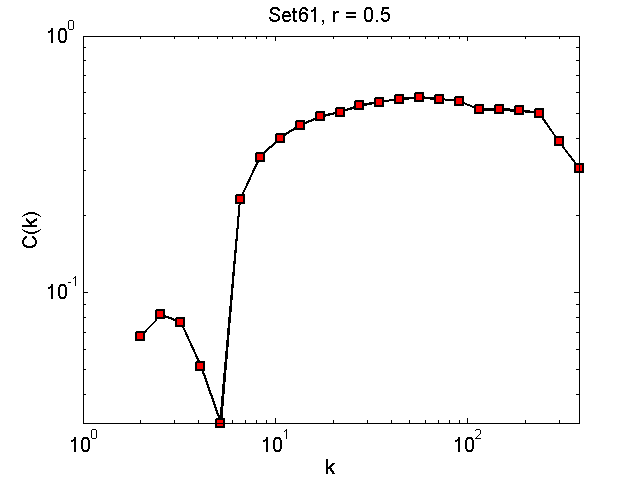
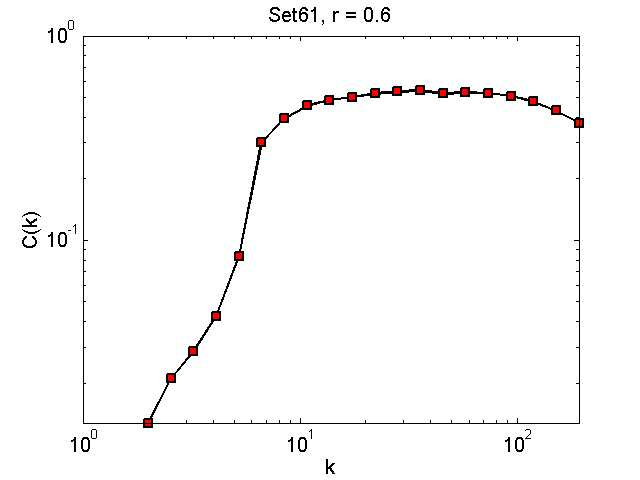
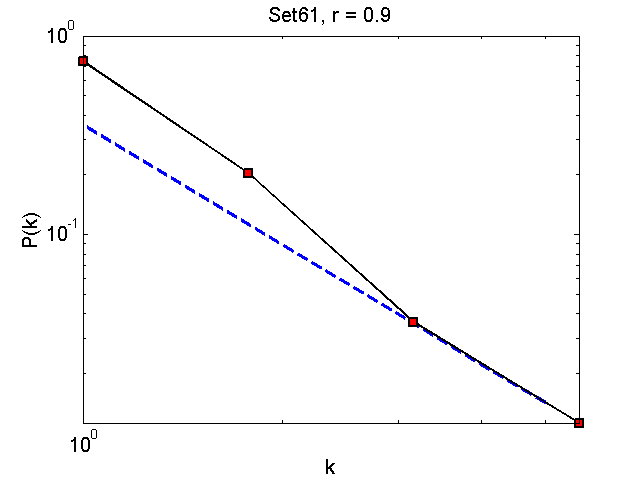
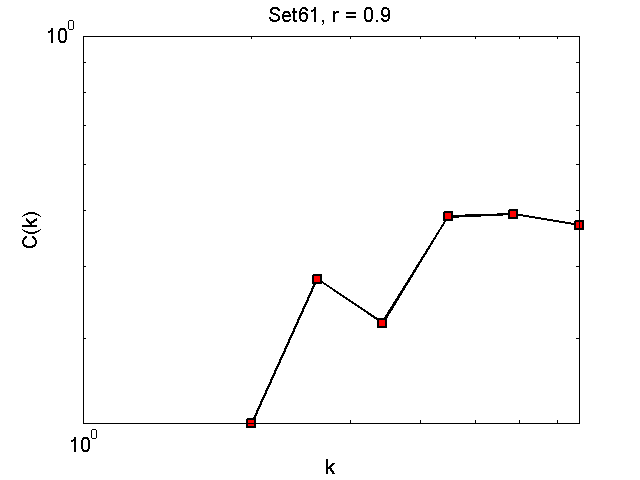
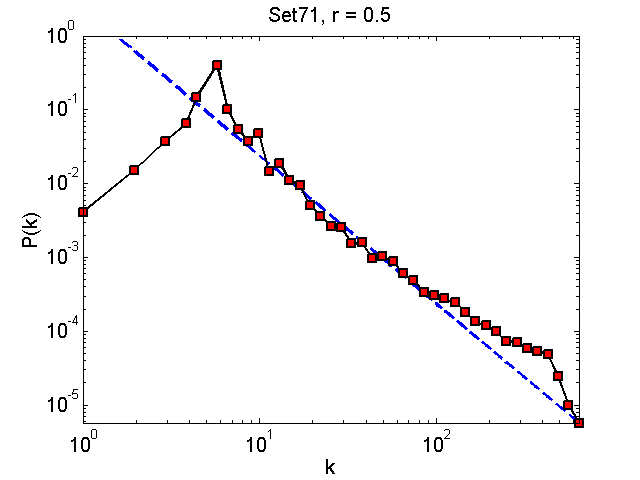
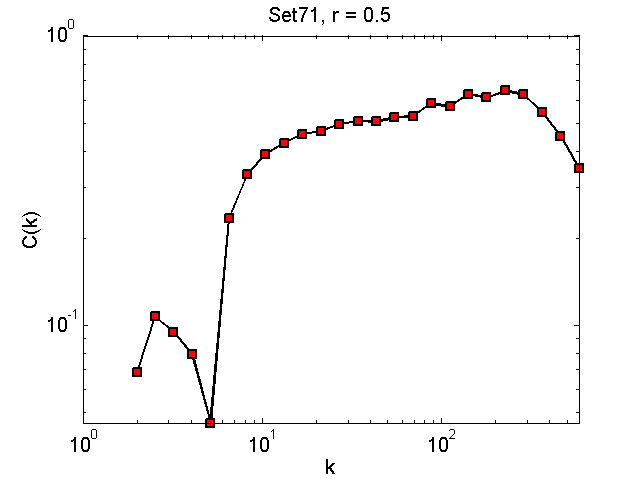
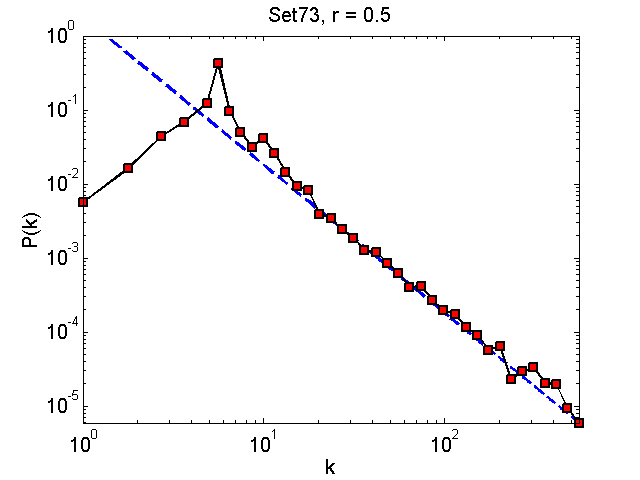
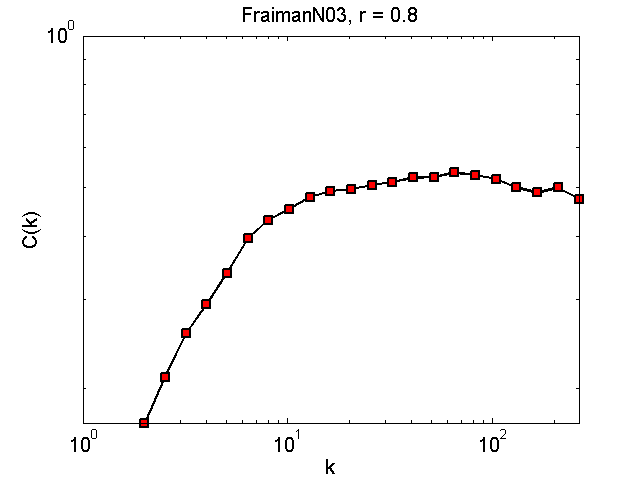
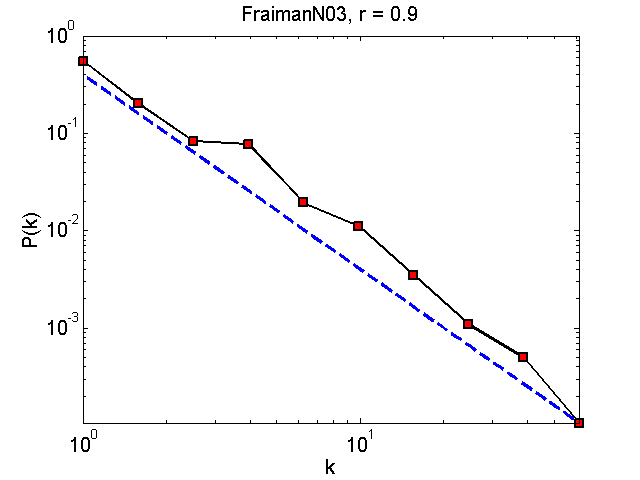
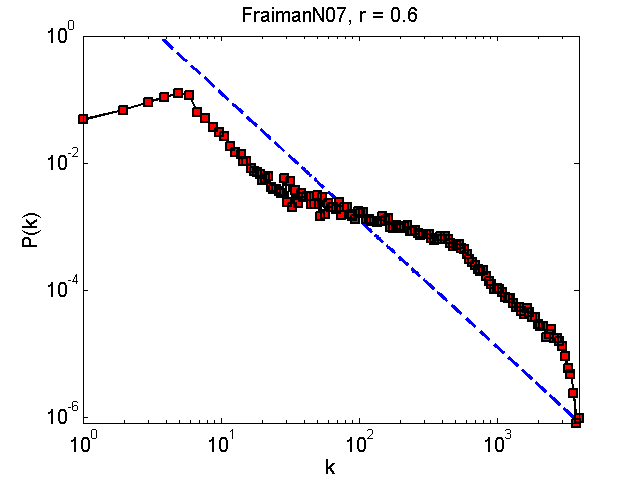
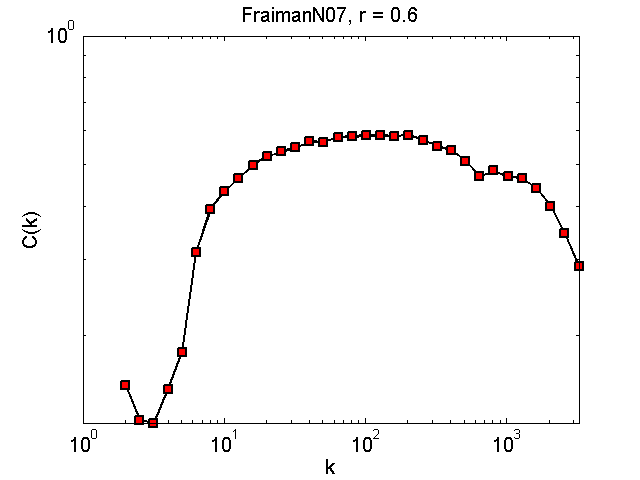
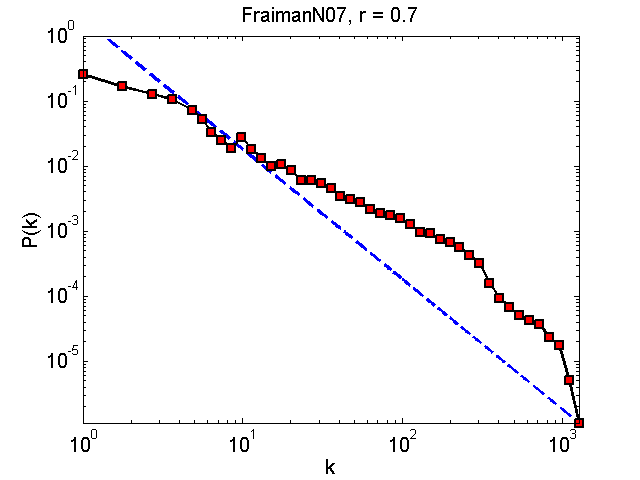
- k -- node degree
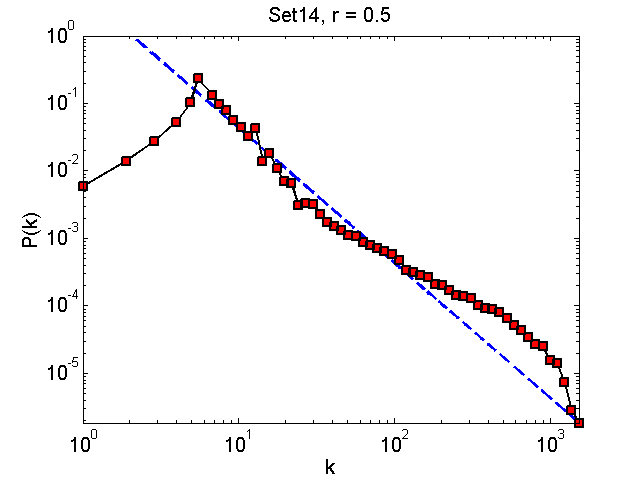
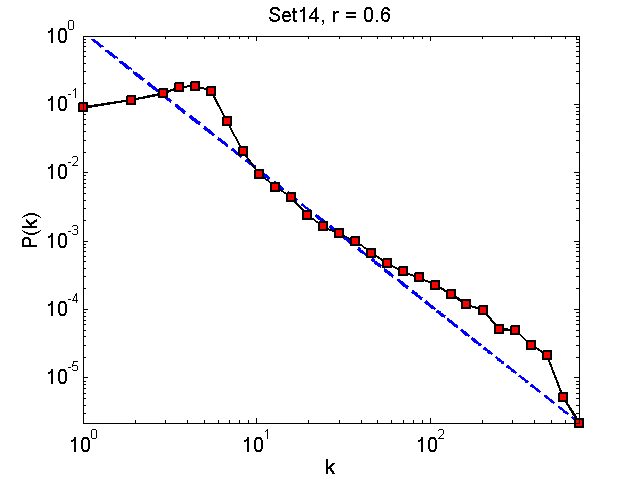
- P(k) -- degree distribution
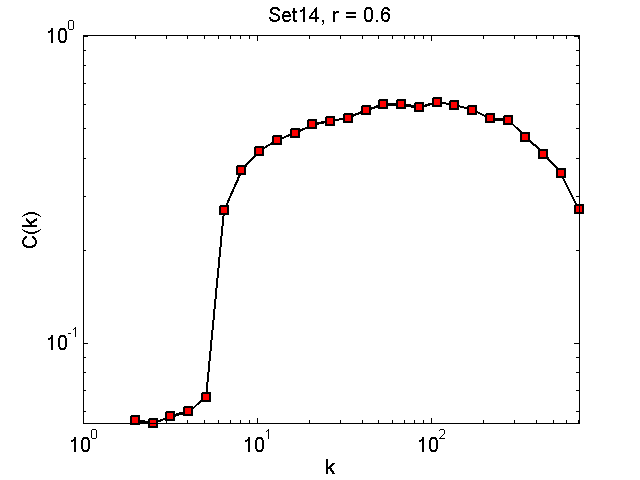
- c(k) -- degree-dependent clustering